
The relation between T and g is given by
$\begin{align}
& \text{A}\text{.}T\propto g \\
& \text{B}.T\propto {{g}^{2}} \\
& \text{C}.{{T}^{2}}\propto g \\
& \text{D}.T\propto \sqrt{\dfrac{l}{g}} \\
\end{align}$
Answer
531.6k+ views
Hint: In the question we are asked to find the relation between ‘T’ and ‘g’. Consider a mass “m” suspended on a wire of length ‘$l$ ’ undergoes simple harmonic motion, ‘T’ is the time period, the time required to complete one oscillation and ‘g’ is the acceleration due to gravity (9.8m/s). To solve this we know the equation of time period; by squaring the known equation we can formulate the relation between ‘T’ and ‘g’.
Formula used:
$T=2\pi \sqrt{\dfrac{l}{g}}$
Time period of a simple oscillation is given by
Complete answer:
To find the relation between ‘T’ and ‘g’,
Let us consider ‘$l$ ’ to be the length of the pendulum.
As we know, time period is given by the equation
$T=2\pi \sqrt{\dfrac{l}{g}}$
Squaring both sides of the equation, we get
${{T}^{2}}=4{{\pi }^{2}}\dfrac{l}{g}$
From this equation we get,
${{T}^{2}}\propto \dfrac{l}{g}$
Thus we can conclude that, $T\propto \sqrt{\dfrac{l}{g}}$, when l is unchanged.
So, the correct answer is “Option D”.
Additional Information:
Time of a simple pendulum derivation:
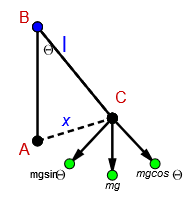
Consider a simple pendulum with a mass ‘m’ suspended on a wire of length ‘$l$ ’.
For one oscillation the pendulum is displaced at an angle of ‘$\theta $’ by ‘x’ distance.
Let ${{T}_{0}}$ be the time period at equilibrium.
${{T}_{0}}=mg$
When the pendulum oscillates, it is displaced at a small angle $\theta $
For this small displacement $\theta $, the restoring force acting will be
Restoring force=$-mg\sin \theta $
Since the angle of displacement $\theta $ is very small here, we can approximate $\sin \theta $ to $\theta $
I.e. $\sin \theta \approx \theta $
Hence the force here can be rewritten as
$F=-mg\theta $
Now let us consider the triangle ABC in the figure.
We know that sin of the angle $\theta $ is the ratio of the opposite side to the hypotenuse of the triangle. Since here $\sin \theta \approx \theta $, we can write this as
$\theta =\dfrac{opposite}{hypotenuse}$
Here the opposite side of the angle is the displacement ‘x’ and the hypotenuse of the triangle is length ‘$l$ ’ of the pendulum. Hence we can rewrite the equation as
$\theta =\dfrac{x}{l}$
Therefore the restoring force on the pendulum is
$F=-mg\theta =-mg\times \dfrac{x}{l}$
By Newton’s second law of motion, we have the equation of motion as
$F=ma$, Where ‘m’ is the mass of the body and ‘a’ is the acceleration.
We can rewrite this equation as
$a=\dfrac{F}{m}$
From the previous equation, we know that $F=-mg\dfrac{x}{l}$. Substituting this here, we get
$a=\dfrac{-mg\left( \dfrac{x}{l} \right)}{m}$
Eliminating the common terms, we get
$a=-\dfrac{g}{l}\times x$
For a simple harmonic motion we know that, $a=-{{\omega }^{2}}x$
On comparing both these equations, we get
$-{{\omega }^{2}}x=-\dfrac{g}{l}x$
By simplifying this,
${{\omega }^{2}}=\dfrac{g}{l}$
$\omega =\sqrt{\dfrac{g}{l}}$
Time period ‘T’ is given by the equation
$T=\dfrac{2\pi }{\omega }$
Substitute the value of $\omega $ in this equation
Therefore time period, $T=2\pi \sqrt{\dfrac{l}{g}}$
Note:
This question can be solved by another method.
We know that, for a simple pendulum its angular frequency $\omega $ is given by
$\omega =\sqrt{\dfrac{g}{l}}$
Time period of an oscillation can also be written as
$T=\dfrac{2\pi }{\omega }$
By substituting the value of angular frequency ($\omega $ ) in the above equation, we get
$T=2\pi \sqrt{\dfrac{l}{g}}$
Thus we get $T\propto \sqrt{\dfrac{l}{g}}$
Hence we get the same solution.
Formula used:
$T=2\pi \sqrt{\dfrac{l}{g}}$
Time period of a simple oscillation is given by
Complete answer:
To find the relation between ‘T’ and ‘g’,
Let us consider ‘$l$ ’ to be the length of the pendulum.
As we know, time period is given by the equation
$T=2\pi \sqrt{\dfrac{l}{g}}$
Squaring both sides of the equation, we get
${{T}^{2}}=4{{\pi }^{2}}\dfrac{l}{g}$
From this equation we get,
${{T}^{2}}\propto \dfrac{l}{g}$
Thus we can conclude that, $T\propto \sqrt{\dfrac{l}{g}}$, when l is unchanged.
So, the correct answer is “Option D”.
Additional Information:
Time of a simple pendulum derivation:

Consider a simple pendulum with a mass ‘m’ suspended on a wire of length ‘$l$ ’.
For one oscillation the pendulum is displaced at an angle of ‘$\theta $’ by ‘x’ distance.
Let ${{T}_{0}}$ be the time period at equilibrium.
${{T}_{0}}=mg$
When the pendulum oscillates, it is displaced at a small angle $\theta $
For this small displacement $\theta $, the restoring force acting will be
Restoring force=$-mg\sin \theta $
Since the angle of displacement $\theta $ is very small here, we can approximate $\sin \theta $ to $\theta $
I.e. $\sin \theta \approx \theta $
Hence the force here can be rewritten as
$F=-mg\theta $
Now let us consider the triangle ABC in the figure.
We know that sin of the angle $\theta $ is the ratio of the opposite side to the hypotenuse of the triangle. Since here $\sin \theta \approx \theta $, we can write this as
$\theta =\dfrac{opposite}{hypotenuse}$
Here the opposite side of the angle is the displacement ‘x’ and the hypotenuse of the triangle is length ‘$l$ ’ of the pendulum. Hence we can rewrite the equation as
$\theta =\dfrac{x}{l}$
Therefore the restoring force on the pendulum is
$F=-mg\theta =-mg\times \dfrac{x}{l}$
By Newton’s second law of motion, we have the equation of motion as
$F=ma$, Where ‘m’ is the mass of the body and ‘a’ is the acceleration.
We can rewrite this equation as
$a=\dfrac{F}{m}$
From the previous equation, we know that $F=-mg\dfrac{x}{l}$. Substituting this here, we get
$a=\dfrac{-mg\left( \dfrac{x}{l} \right)}{m}$
Eliminating the common terms, we get
$a=-\dfrac{g}{l}\times x$
For a simple harmonic motion we know that, $a=-{{\omega }^{2}}x$
On comparing both these equations, we get
$-{{\omega }^{2}}x=-\dfrac{g}{l}x$
By simplifying this,
${{\omega }^{2}}=\dfrac{g}{l}$
$\omega =\sqrt{\dfrac{g}{l}}$
Time period ‘T’ is given by the equation
$T=\dfrac{2\pi }{\omega }$
Substitute the value of $\omega $ in this equation
Therefore time period, $T=2\pi \sqrt{\dfrac{l}{g}}$
Note:
This question can be solved by another method.
We know that, for a simple pendulum its angular frequency $\omega $ is given by
$\omega =\sqrt{\dfrac{g}{l}}$
Time period of an oscillation can also be written as
$T=\dfrac{2\pi }{\omega }$
By substituting the value of angular frequency ($\omega $ ) in the above equation, we get
$T=2\pi \sqrt{\dfrac{l}{g}}$
Thus we get $T\propto \sqrt{\dfrac{l}{g}}$
Hence we get the same solution.
Recently Updated Pages
NCERT Solutions For Class 12 Maths Three Dimensional Geometry Exercise 11.2

NCERT Solutions For Class 11 Maths Sets Exercise 1.4

NCERT Solutions For Class 11 Maths Miscellaneous Exercise - Limits and Derivatives

NCERT Solutions For Class 12 Maths Integrals Exercise 7.9

NCERT Solutions For Class 11 Biology In Hindi - Excretory Products And Their Elimination

NCERT Solutions For Class 2 Hindi Sarangi - Gire Taal Mein Chanda Maama

Trending doubts
1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Proton was discovered by A Thomson B Rutherford C Chadwick class 11 chemistry CBSE

Draw a diagram of nephron and explain its structur class 11 biology CBSE




