
A resonance tube is old and has a jagged end. It is still used in the laboratory to determine velocity of sound in air. A tuning fork of frequency 512 Hz produces first resonance when the tube is filled with water to a mark 11 cm below a reference mark, near the open end of the tube. The experiment is repeated with another fork of frequency 256 Hz which produces first resonance when water reaches a mark 27 cm below the reference mark. The velocity of sound in air, obtained in the experiment, is close to:
A. $328m/s$
B. $322m/s$
C. $341m/s$
D. $335m/s$
Answer
490.2k+ views
Hint:
1. Velocity of sound is constant in a given medium.
2. First resonance frequency occurs for air column length, $l = \dfrac{\lambda }{4}$ in ideal scenarios.
3. End correction must be added to the path length of first resonance frequency.
Formula Used:
1. Distance between two consecutive nodes $ = \dfrac{\lambda }{2}$ …… (a)
2. Distance between Antinode and next successive node $ = \dfrac{\lambda }{4}$ ……. (b)
3.First resonance frequency with air column of length ${l_{air}} + e = \dfrac{\lambda }{4}$ ……. (c)
where $e$ is end correction
4. Constant speed of sound wave with $f$ be frequency of tuning fork and $\lambda $ be wavelength of sound wave $v = \lambda f$ $ \Rightarrow \lambda = \dfrac{v}{f}$ …… (d)
Figure 1 shows rough sketch for first resonance air column:
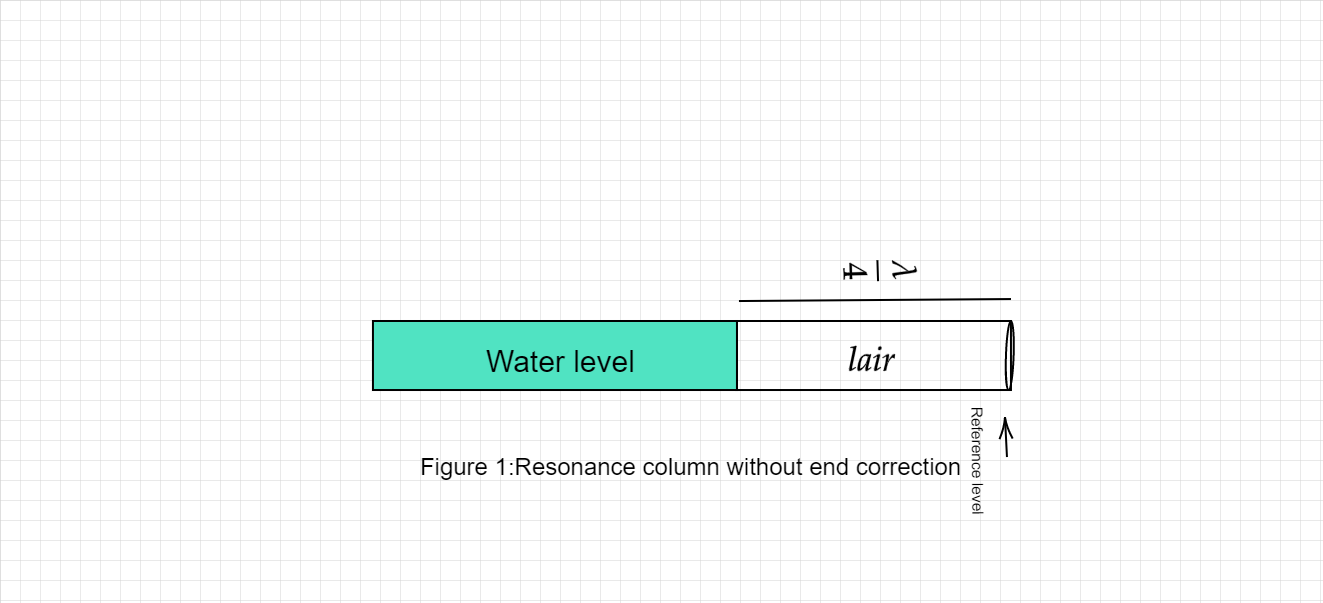
Complete step by step answer:
Given,
Two tuning forks of frequencies ${f_1}$ and ${f_2}$ be 512 Hz and 256 Hz respectively.
Corresponding to each frequency’s paths, the lengths of the first resonance air column are 11cm and 27 cm respectively.
Let, end correction be $e$
Step 1 of 4:
Using equation (c) and (b) for frequency ${f_1}$=512 Hz we get
$\dfrac{{{\lambda _1}}}{4} = {l_{1,air}} + e$ …… (1)
Given ${l_{air}} = 11cm$,
Putting substitutions from equation (1) and (d) we get,
$\dfrac{v}{{4 \times {f_1}}} = 11cm + e$ …… (2)
Step 2 of 4:
Similarly, using equation (c) and (b) for frequency ${f_2}$ =256 Hz we get
$\dfrac{{{\lambda _2}}}{4} = {l_{2,}}_{air} + e$ …… (3)
Putting substitutions from equation (1) and (d) we get,
$\dfrac{v}{{4 \times {f_2}}} = 27cm + e$ …… (4)
Step 3 of 4:
Subtracting equation (2) from equation (4) we get,
$\dfrac{v}{{4 \times {f_2}}} - \dfrac{v}{{4 \times {f_1}}} = 27cm + e - 11cm + e$
$ \Rightarrow \dfrac{v}{4}\left( {\dfrac{1}{{{f_2}}} - \dfrac{1}{{{f_1}}}} \right) = 16 \times {10^{ - 2}}m$ …… (5)
Step 4 of 4:
Putting values of ${f_1}$ and ${f_2}$ in equation (5) we get,
$v = 328m/s$
Correct Answer: A.$328m/s$
Additional Information: At the opening of a vessel there always lies an antinode. There lies a node at the interface between air and fluid since the fluid boundary acts as a closed vessel because at that point no displacement is possible.
Note: End correction should always be considered while solving sound wave problems in a vessel or tube.
1. Velocity of sound is constant in a given medium.
2. First resonance frequency occurs for air column length, $l = \dfrac{\lambda }{4}$ in ideal scenarios.
3. End correction must be added to the path length of first resonance frequency.
Formula Used:
1. Distance between two consecutive nodes $ = \dfrac{\lambda }{2}$ …… (a)
2. Distance between Antinode and next successive node $ = \dfrac{\lambda }{4}$ ……. (b)
3.First resonance frequency with air column of length ${l_{air}} + e = \dfrac{\lambda }{4}$ ……. (c)
where $e$ is end correction
4. Constant speed of sound wave with $f$ be frequency of tuning fork and $\lambda $ be wavelength of sound wave $v = \lambda f$ $ \Rightarrow \lambda = \dfrac{v}{f}$ …… (d)
Figure 1 shows rough sketch for first resonance air column:

Complete step by step answer:
Given,
Two tuning forks of frequencies ${f_1}$ and ${f_2}$ be 512 Hz and 256 Hz respectively.
Corresponding to each frequency’s paths, the lengths of the first resonance air column are 11cm and 27 cm respectively.
Let, end correction be $e$
Step 1 of 4:
Using equation (c) and (b) for frequency ${f_1}$=512 Hz we get
$\dfrac{{{\lambda _1}}}{4} = {l_{1,air}} + e$ …… (1)
Given ${l_{air}} = 11cm$,
Putting substitutions from equation (1) and (d) we get,
$\dfrac{v}{{4 \times {f_1}}} = 11cm + e$ …… (2)
Step 2 of 4:
Similarly, using equation (c) and (b) for frequency ${f_2}$ =256 Hz we get
$\dfrac{{{\lambda _2}}}{4} = {l_{2,}}_{air} + e$ …… (3)
Putting substitutions from equation (1) and (d) we get,
$\dfrac{v}{{4 \times {f_2}}} = 27cm + e$ …… (4)
Step 3 of 4:
Subtracting equation (2) from equation (4) we get,
$\dfrac{v}{{4 \times {f_2}}} - \dfrac{v}{{4 \times {f_1}}} = 27cm + e - 11cm + e$
$ \Rightarrow \dfrac{v}{4}\left( {\dfrac{1}{{{f_2}}} - \dfrac{1}{{{f_1}}}} \right) = 16 \times {10^{ - 2}}m$ …… (5)
Step 4 of 4:
Putting values of ${f_1}$ and ${f_2}$ in equation (5) we get,
$v = 328m/s$
Correct Answer: A.$328m/s$
Additional Information: At the opening of a vessel there always lies an antinode. There lies a node at the interface between air and fluid since the fluid boundary acts as a closed vessel because at that point no displacement is possible.
Note: End correction should always be considered while solving sound wave problems in a vessel or tube.
Recently Updated Pages
Glucose when reduced with HI and red Phosphorus gives class 11 chemistry CBSE

The highest possible oxidation states of Uranium and class 11 chemistry CBSE

Find the value of x if the mode of the following data class 11 maths CBSE

Which of the following can be used in the Friedel Crafts class 11 chemistry CBSE

A sphere of mass 40 kg is attracted by a second sphere class 11 physics CBSE

Statement I Reactivity of aluminium decreases when class 11 chemistry CBSE

Trending doubts
10 examples of friction in our daily life

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

State and prove Bernoullis theorem class 11 physics CBSE

What organs are located on the left side of your body class 11 biology CBSE

How many valence electrons does nitrogen have class 11 chemistry CBSE




