Stepwise Solutions to Application of Integrals Class 12 Miscellaneous Problems
If you’re preparing for CBSE Board exams and want complete confidence in calculus, these NCERT Solutions for Class 12 Maths Chapter 8 Miscellaneous Exercise are designed for you. This section brings together critical application questions on integration, focusing especially on areas under curves and methods to find the area between two functions. With attentive explanations, each step matches the latest board marking scheme for 2025.
Table of Content
Questions in this miscellaneous exercise challenge you with calculation-rich scenarios and real-life contexts. Students often ask how to solve area between curves problems for CBSE Board or search for application of integrals class 12 miscellaneous solutions that simplify visualising bounded regions. Here, you’ll find answers that break down these advanced applications clearly.
Scoring is strategic too—Application of Integrals carries a reliable weightage of 6 marks each year and is a must-practice for full marks. Vedantu’s expert-reviewed stepwise solutions and exam-ready tricks help you master definite integrals and avoid common mistakes, making your revision targeted and efficient. For complete coverage, always refer to the Class 12 Maths syllabus as per the latest CBSE guidelines.
Miscellaneous Exercise
1. Find the area under the given curves and given lines:
(i) \[\text{y=}{{\text{x}}^{\text{2}}}\text{,x=1,x=2}\] and \[\text{x-axis}\]
Ans:
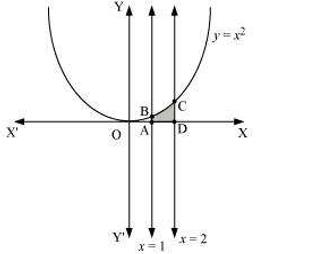
\[\text{AreaADCBA=}\int_{\text{1}}^{\text{2}}{\text{y}}\text{dx}\]
substitute $y={{x}^{2}}$
\[\text{=}\int_{\text{1}}^{\text{2}}{{{\text{x}}^{\text{2}}}}\text{dx}\]
Substituting the limits,
\[\text{=}\frac{\text{8}}{\text{3}}\text{-}\frac{\text{1}}{\text{3}}\]
\[\text{=}\frac{\text{7}}{\text{3}}\text{units}\]
(ii) \[\text{y=}{{\text{x}}^{4}}\text{,x=1,x=5}\] and \[\text{x-axis}\]
Ans:
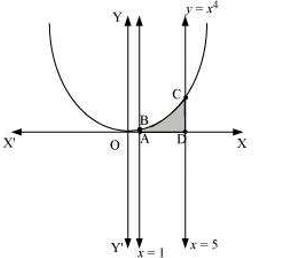
\[\text{AreaofADCBA=}\int_{\text{1}}^{\text{5}}{{{\text{x}}^{\text{4}}}}\text{dx}\]
Integrating using the power rule,
\[\text{=}\left[ \frac{{{\text{x}}^{\text{5}}}}{\text{5}} \right]_{\text{1}}^{\text{5}}\]
Substituting the limits,
\[\text{=}\frac{{{\text{(5)}}^{\text{5}}}}{\text{5}}\text{-}\frac{\text{1}}{\text{5}}\]
Simplifying,
\[\text{=(5}{{\text{)}}^{\text{4}}}\text{-}\frac{\text{1}}{\text{5}}\]
\[\text{=625-}\frac{\text{1}}{\text{5}}\]
\[\text{=624}\text{.8 units}\]
2. Sketch the graph of \[\text{y=}\left| \text{x+3} \right|\] and evaluate \[\int_{-6}^{0}{\left| \text{x+3} \right|}\text{dx}\].
Ans:
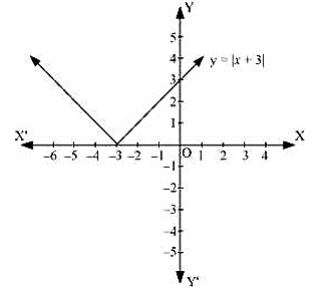
\[\left( \text{x+3} \right)\le 0\] for \[\text{-6}\le \text{x}\le \text{-3}\]
\[\left( \text{x+3} \right)\ge \text{0}\] for \[\text{-3}\le \text{x}\le 0\]
Therefore,
\[\int_{\text{-6}}^{\text{0}}{\text{ }\!\!|\!\!\text{ }}\text{(x+3) }\!\!|\!\!\text{ dx=-}\int_{\text{-6}}^{\text{-3}}{\text{(x+3)}}\text{dx+}\int_{\text{-3}}^{\text{0}}{\text{(x+3)}}\text{dx}\]
Integrating using the power rule
\[\text{= -}\left[ \frac{{{\text{x}}^{\text{2}}}}{\text{2}}\text{+3x} \right]_{\text{-6}}^{\text{-3}}\text{+}\left[ \frac{{{\text{x}}^{\text{2}}}}{\text{2}}\text{+3x} \right]_{\text{-3}}^{\text{0}}\]
Substituting the limits,
\[\text{=-}\left[ \left( \frac{{{\text{(-3)}}^{\text{2}}}}{\text{2}}\text{+3(-3)} \right)\text{-}\left( \frac{{{\text{(-6)}}^{\text{2}}}}{\text{2}}\text{+3(-6)} \right) \right]\text{+}\left[ \text{0-}\left( \frac{{{\text{(-3)}}^{\text{2}}}}{\text{2}}\text{+3(-3)} \right) \right]\]
Simplifying,
\[\text{= -}\left[ \text{-}\frac{\text{9}}{\text{2}} \right]\text{-}\left[ \text{-}\frac{\text{9}}{\text{2}} \right]\]
\[\text{=9}\]
3. Find the area bounded by the curve \[\text{y=sinx}\] between \[\text{x=0}\] and \[\text{x=2 }\!\!\pi\!\!\text{ }\].
Ans:
Therefore, \[\text{area = Area OABO+ Area BCDB}\]
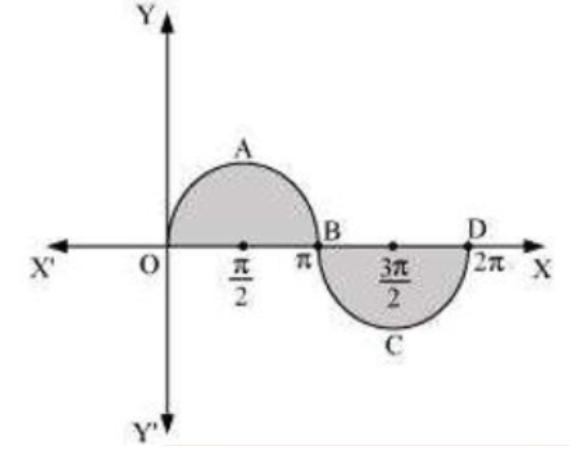
Area Bounded by Curve y=sinx
\[\text{=}\int_{\text{0}}^{\text{ }\!\!\pi\!\!\text{ }}{\text{sin}}\text{xdx+}\left| \int_{\text{ }\!\!\pi\!\!\text{ }}^{\text{2 }\!\!\pi\!\!\text{ }}{\text{sin}}\text{xdx} \right|\]
\[\text{= }\!\![\!\!\text{ -cosx }\!\!]\!\!\text{ }_{\text{0}}^{\text{ }\!\!\pi\!\!\text{ }}\text{+}\left| \text{ }\!\![\!\!\text{ -cosx }\!\!]\!\!\text{ }_{\text{ }\!\!\pi\!\!\text{ }}^{\text{2 }\!\!\pi\!\!\text{ }} \right|\]
Substituting the limits,
\[\text{= }\!\![\!\!\text{ -cos }\!\!\pi\!\!\text{ +cos0 }\!\!]\!\!\text{ + }\!\!|\!\!\text{ -cos2 }\!\!\pi\!\!\text{ +cos }\!\!\pi\!\!\text{ }\!\!|\!\!\text{ }\]
Simplifying,
\[\text{=1+1+ }\!\!|\!\!\text{ (-1-1) }\!\!|\!\!\text{ }\]
\[\text{=2+ }\!\!|\!\!\text{ -2 }\!\!|\!\!\text{ }\]
\[\text{=2+2}\]
\[\text{=4 units}\]
4. Area bounded by the curve \[\text{y=}{{\text{x}}^{3}}\], the \[\text{x-axis}\] and the ordinates \[\text{x = -2}\] and \[\text{x = 1}\] is
A. \[\text{-9}\]
B. \[\text{-}\frac{\text{15}}{\text{4}}\]
C. \[\frac{\text{15}}{\text{4}}\]
D. \[\frac{\text{17}}{\text{4}}\]
Ans:
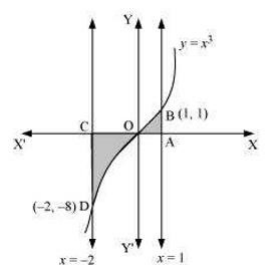
As shown in the diagram, the required area is:
\[\text{Required area =}\int_{\text{-2}}^{\text{1}}{\text{y}}\text{dx}\]
\[\text{=}\int_{\text{-2}}^{\text{1}}{{{\text{x}}^{\text{3}}}}\text{dx}\]
Integrating using the power rule
\[\text{=}\left[ \frac{{{\text{x}}^{\text{4}}}}{\text{4}} \right]_{\text{-2}}^{\text{1}}\]
Substituting the limits,
\[\text{=}\left[ \frac{\text{1}}{\text{4}}\text{-}\frac{{{\text{(-2)}}^{\text{4}}}}{\text{4}} \right]\]
Simplifying,
\[\text{=}\left( \frac{\text{1}}{\text{4}}\text{-4} \right)\]
\[\text{= -}\frac{\text{15}}{\text{4}}\text{ units}\]
So, the correct answer is \[\text{ -}\frac{\text{15}}{\text{4}}\text{ units}\] option B.
5. The area bounded by the curve \[\text{y=x}\left| \text{x} \right|\text{,x-axis}\] and the ordinate \[\text{x = 1}\] and \[\text{x = -1}\] is given by (Hint \[\text{y = }{{\text{x}}^{2}}\] if \[x>0\] and \[\text{y = -}{{\text{x}}^{2}}\] if \[x<0\])
A. \[0\]
B. \[\frac{\text{1}}{\text{3}}\]
C. \[\frac{2}{\text{3}}\]
D. \[\frac{4}{\text{3}}\]
Ans:
\[\text{Required area=}\int_{\text{-1}}^{\text{1}}{\text{y}}\text{dx}\]
\[\text{=}\int_{\text{-1}}^{\text{1}}{\text{x}}\text{ }\!\!|\!\!\text{ x }\!\!|\!\!\text{ dx}\]
\[\text{= -}\int_{\text{-1}}^{\text{0}}{{{\text{x}}^{\text{2}}}}\text{dx+}\int_{\text{0}}^{\text{1}}{{{\text{x}}^{\text{2}}}}\text{dx}\]
Integrating using the power rule
\[\text{= -}\left[ \frac{{{\text{x}}^{\text{3}}}}{\text{3}} \right]_{\text{-1}}^{\text{0}}\text{+}\left[ \frac{{{\text{x}}^{\text{3}}}}{\text{3}} \right]_{\text{0}}^{\text{1}}\]
Substituting the limits,
\[\text{= -}\left( \text{-}\frac{\text{1}}{\text{3}} \right)\text{+}\frac{\text{1}}{\text{3}}\]
\[\text{=}\frac{\text{2}}{\text{3}}\text{units}\]
So, the correct answer is \[\frac{\text{2}}{\text{3}}\text{units}\] option C.
Conclusion
The Class 12 Maths Chapter 8 Miscellaneous Exercise Solutions is important for understanding various concepts thoroughly. Application of Integrals Class 12 Miscellaneous includes a variety of issues that call for the use of several formulas and methods. It's crucial to concentrate on comprehending the fundamental ideas behind every question, as opposed to merely learning the answers by heart. To successfully complete this task, keep in mind that you must comprehend the theory underlying each idea, practise frequently, and consult solved examples.
Class 12 Maths Chapter 8: Exercises Breakdown
CBSE Class 12 Maths Chapter 8 Other Study Materials
Chapter-Specific NCERT Solutions for Class 12 Maths
Given below are the chapter-wise NCERT Solutions for Class 12 Maths. Go through these chapter-wise solutions to be thoroughly familiar with the concepts.
