
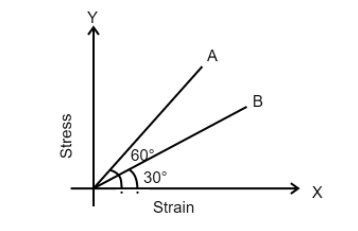
The stress versus strain graphs for wires of two materials (A)and (B) are as shown in the figure. If ${Y_A}$and ${Y_B}$ are Young's moduli of the materials, then:
(A) ${Y_B} = 2{Y_A}$
(B) ${Y_B} = {Y_A}$
(C) ${Y_B} = 3{Y_A}$
(D) $3{Y_B} = {Y_A}$
Answer
173.4k+ views
.Hint. We solve this question by finding the values of Young’s modulus for both the materials. We find Young’s modulus by finding the slope of the line in the graph for each material. We find the slope by using trigonometric properties. The angle made by the line in the stress vs strain graph is already given. By using these angles in trigonometry properties to find Young’s modulus we get a relation between ${Y_A}$and ${Y_B}$
Complete step by step answer. The slope of stress vs strain graph gives us Young’s modulus.
In the graph, we are given the angle made by the line in the stress vs strain graph in both cases.
We know that slope is equal to $\tan \theta $
In the case of material (A)
The slope is equal to $\tan 60^\circ $
Material (B)
The slope is equal to $\tan 30^\circ $
We know that slope is equal to Young’s modulus hence
${Y_A} = \tan 60^\circ = \sqrt 3 $
${Y_B} = \tan 30^\circ = \dfrac{1}{{\sqrt 3 }}$
Diving Young’s modulus of (A) with Young’s modulus of (B) we get $\dfrac{{{Y_A}}}{{{Y_B}}} = \dfrac{{\sqrt 3 }}{{\dfrac{1}{{\sqrt 3 }}}} = 3$
Hence $3{Y_B} = {Y_A}$
Option (D) $3{Y_B} = {Y_A}$ is the correct answer.
Additional information Young’s modulus is defined as the measure of ability of a material to withstand changes in length when it is under a lengthwise tension of compression. It is also referred to as modulus of elasticity.
Note We find the slope in the graph by using tanθ because we are assuming the graph as a right-angled triangle. And $\tan \theta $is equal to the opposite by the adjacent side of the assumed triangle. In the case of stress vs strain graph opposite is equal to stress and adjacent is equal to the strain. Hence $\tan \theta $ is equal to Young’s modulus. Therefore, we use $\tan \theta $ to find the slope.
Complete step by step answer. The slope of stress vs strain graph gives us Young’s modulus.
In the graph, we are given the angle made by the line in the stress vs strain graph in both cases.
We know that slope is equal to $\tan \theta $
In the case of material (A)
The slope is equal to $\tan 60^\circ $
Material (B)
The slope is equal to $\tan 30^\circ $
We know that slope is equal to Young’s modulus hence
${Y_A} = \tan 60^\circ = \sqrt 3 $
${Y_B} = \tan 30^\circ = \dfrac{1}{{\sqrt 3 }}$
Diving Young’s modulus of (A) with Young’s modulus of (B) we get $\dfrac{{{Y_A}}}{{{Y_B}}} = \dfrac{{\sqrt 3 }}{{\dfrac{1}{{\sqrt 3 }}}} = 3$
Hence $3{Y_B} = {Y_A}$
Option (D) $3{Y_B} = {Y_A}$ is the correct answer.
Additional information Young’s modulus is defined as the measure of ability of a material to withstand changes in length when it is under a lengthwise tension of compression. It is also referred to as modulus of elasticity.
Note We find the slope in the graph by using tanθ because we are assuming the graph as a right-angled triangle. And $\tan \theta $is equal to the opposite by the adjacent side of the assumed triangle. In the case of stress vs strain graph opposite is equal to stress and adjacent is equal to the strain. Hence $\tan \theta $ is equal to Young’s modulus. Therefore, we use $\tan \theta $ to find the slope.
Recently Updated Pages
Sets, Relations, and Functions Mock Test 2025-26

JEE Main Mock Test 2025-26: Purification & Characterisation of Organic Compounds

JEE Main 2025 Coordination Compounds Mock Test – Free Practice Online

JEE Main 2025-26 Equilibrium Mock Test: Free Practice Online

JEE Main Mock Test 2025-26: D and F Block Elements Practice

JEE Main Mock Test 2025-26: Chapter-Wise Practice Papers

Trending doubts
Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Instantaneous Velocity - Formula based Examples for JEE

Electron Gain Enthalpy and Electron Affinity for JEE

Degree of Dissociation and Its Formula With Solved Example for JEE

What is Hybridisation in Chemistry?

Assertion Minimum number of nonequal Vectors in a plane class 11 physics JEE_Main

Other Pages
NCERT Solutions For Class 11 Physics Chapter 8 Mechanical Properties Of Solids - 2025-26

JEE Advanced 2025 Notes

Work Energy and Power Class 11 Physics Chapter 5 CBSE Notes - 2025-26

Find the frictional force between the two blocks in class 11 physics JEE_MAIN

A pilot in a plane wants to go 500km towards the north class 11 physics JEE_Main

Atomic Structure - Electrons, Protons, Neutrons and Atomic Models




