
The coefficient of static friction between a block of mass $m$ and an incline is ${\mu _s} = 0.3$ . What can be the maximum angle $\theta $ of the incline with the horizontal so that the block does not slip on the plane? If the inclined makes an angle $\dfrac{\theta }{2}$ with the horizontal, find the frictional force on the block.
Answer
180k+ views
Hint: Maximum angle of the incline so that the block does not slip will be obtained when the friction force opposing it’s slipping will be almost equal to the component of its weight along the incline.
Frictional force on the block when angle will be $\dfrac{\theta }{2}$ , will be equal to its weight component at that moment along the incline.
Complete step by step solution:
The maximum angle $\theta $ of the incline with the horizontal so that the block does not slip on the plane will be the condition when the block will just about to slip. So at that point, static friction will be working at its peak.
At the time, when the block is about to slip, friction force on the block in upwards direction will be equal to the component of weight of block.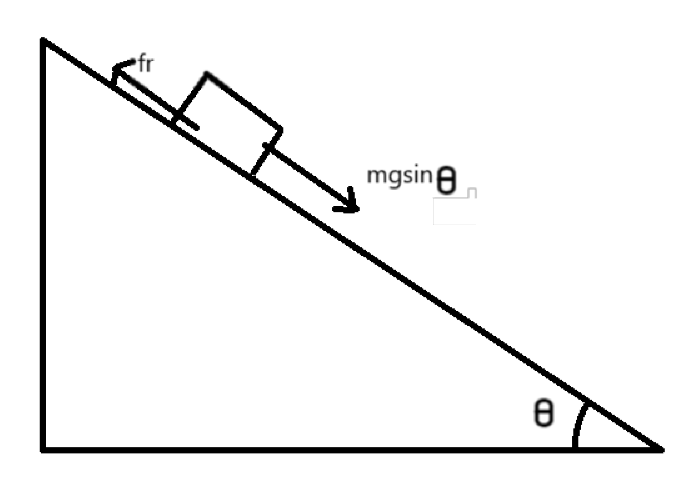
This friction force is equal to the multiplication of coefficient of static friction and the normal force acting on the block.
Normal force acting on the block is $\cos $ component of weight.
So normal force is $mg\cos \theta $
Friction force acting on the block at this condition will be $\mu mg\cos \theta $
Since, this is equivalent to $mg\sin \theta $ ,
Therefore, $mg\sin \theta = \mu mg\cos \theta $ ,
On simplifying this we get,
$\sin \theta = \mu \cos \theta $
So we get,
$\dfrac{{\sin \theta }}{{\cos \theta }} = \mu $ ,
Hence, $\tan \theta = \mu $
So we get our answer as, $\theta = {\tan ^{ - 1}}\mu $ ,
Putting, value of coefficient of friction in above equation we get,
$\theta = {\tan ^{ - 1}}\left( {0.3} \right)$
Hence, when the $\theta = {\tan ^{ - 1}}\left( {0.3} \right)$ , the block will be about to slip.
When the angle of inclination will be $\theta ' = \dfrac{\theta }{2}$ ,
The frictional force acting on the block will be equal to the component of weight of block along the incline which is $mg\sin \theta '$ .
So, ${\text{Frictional Force = mg sin}}\theta '$
On putting value of $\theta ' = \dfrac{\theta }{2}$ in above equation we get,
${\text{Frictional Force = mg sin}}\left( {\dfrac{\theta }{2}} \right)$ ,
Note: Since, we got $\theta = {\tan ^{ - 1}}\mu $ , for the block which is just about to slip from the incline place, therefore, we can observe that this angle does not depend on the mass of the block, it only depends on the incline angle of wedge. In the second example, frictional force will not be equal to the multiplication of coefficient of static friction and the normal force acting on the block because it is not at its peak, rather it’s only balancing the weight component of the block along the incline.
Frictional force on the block when angle will be $\dfrac{\theta }{2}$ , will be equal to its weight component at that moment along the incline.
Complete step by step solution:
The maximum angle $\theta $ of the incline with the horizontal so that the block does not slip on the plane will be the condition when the block will just about to slip. So at that point, static friction will be working at its peak.
At the time, when the block is about to slip, friction force on the block in upwards direction will be equal to the component of weight of block.

This friction force is equal to the multiplication of coefficient of static friction and the normal force acting on the block.
Normal force acting on the block is $\cos $ component of weight.
So normal force is $mg\cos \theta $
Friction force acting on the block at this condition will be $\mu mg\cos \theta $
Since, this is equivalent to $mg\sin \theta $ ,
Therefore, $mg\sin \theta = \mu mg\cos \theta $ ,
On simplifying this we get,
$\sin \theta = \mu \cos \theta $
So we get,
$\dfrac{{\sin \theta }}{{\cos \theta }} = \mu $ ,
Hence, $\tan \theta = \mu $
So we get our answer as, $\theta = {\tan ^{ - 1}}\mu $ ,
Putting, value of coefficient of friction in above equation we get,
$\theta = {\tan ^{ - 1}}\left( {0.3} \right)$
Hence, when the $\theta = {\tan ^{ - 1}}\left( {0.3} \right)$ , the block will be about to slip.
When the angle of inclination will be $\theta ' = \dfrac{\theta }{2}$ ,
The frictional force acting on the block will be equal to the component of weight of block along the incline which is $mg\sin \theta '$ .
So, ${\text{Frictional Force = mg sin}}\theta '$
On putting value of $\theta ' = \dfrac{\theta }{2}$ in above equation we get,
${\text{Frictional Force = mg sin}}\left( {\dfrac{\theta }{2}} \right)$ ,
Note: Since, we got $\theta = {\tan ^{ - 1}}\mu $ , for the block which is just about to slip from the incline place, therefore, we can observe that this angle does not depend on the mass of the block, it only depends on the incline angle of wedge. In the second example, frictional force will not be equal to the multiplication of coefficient of static friction and the normal force acting on the block because it is not at its peak, rather it’s only balancing the weight component of the block along the incline.
Recently Updated Pages
JEE Main 2022 (June 27th Shift 2) Chemistry Question Paper with Answer Key

JEE Main 2022 (July 27th Shift 2) Chemistry Question Paper with Answer Key

Degree of Dissociation Important Concepts and Tips for JEE

Learn Conversion of Galvanometer into Ammeter and Voltmeter for JEE Main

JEE Main Chemistry Question Paper PDF Download with Answer Key

Electric Field Due To Uniformly Charged Ring - JEE Main 2025

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Displacement-Time Graph and Velocity-Time Graph for JEE

Atomic Structure - Electrons, Protons, Neutrons and Atomic Models

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

What is Hybridisation in Chemistry?

Other Pages
NCERT Solutions For Class 11 Physics Chapter 2 Motion In A Straight Line - 2025-26

NCERT Solutions For Class 11 Physics Chapter 1 Units and Measurements - 2025-26

NCERT Solutions For Class 11 Physics Chapter 3 Motion In A Plane - 2025-26

JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Units And Measurements Class 11 Physics Chapter 1 CBSE Notes - 2025-26

Motion in a Straight Line Class 11 Physics Chapter 2 CBSE Notes - 2025-26




