




How to Derive the Equation of Trajectory Step by Step for JEE/Boards?
Understanding the Derivation Of Equation Of Trajectory is essential for every JEE Main aspirant. It explains how and why projectiles like balls or stones follow a curved path under gravity. If you get the derivation and its formulae clear, many Physics questions on motion, range, and projectile path become less tricky. Let’s unpack the physical concept, see the main equations stepwise, and learn common exam approaches.
In Physics, a projectile’s path in two dimensions is governed by a set of motion equations. The trajectory equation mathematically describes this curved path, often a parabola, and helps predict a particle’s position at any point. Concepts like “proof of trajectory,” “projectile motion path formula,” and “parabola in projectile” are tightly linked to this topic.
Physical Meaning and Variables in the Derivation Of Equation Of Trajectory
A projectile is an object thrown with some velocity, making an angle θ with the horizontal. It moves under gravity’s influence only, with air resistance ignored for JEE. The initial velocity (u) can be split into horizontal (u cosθ) and vertical (u sinθ) components.
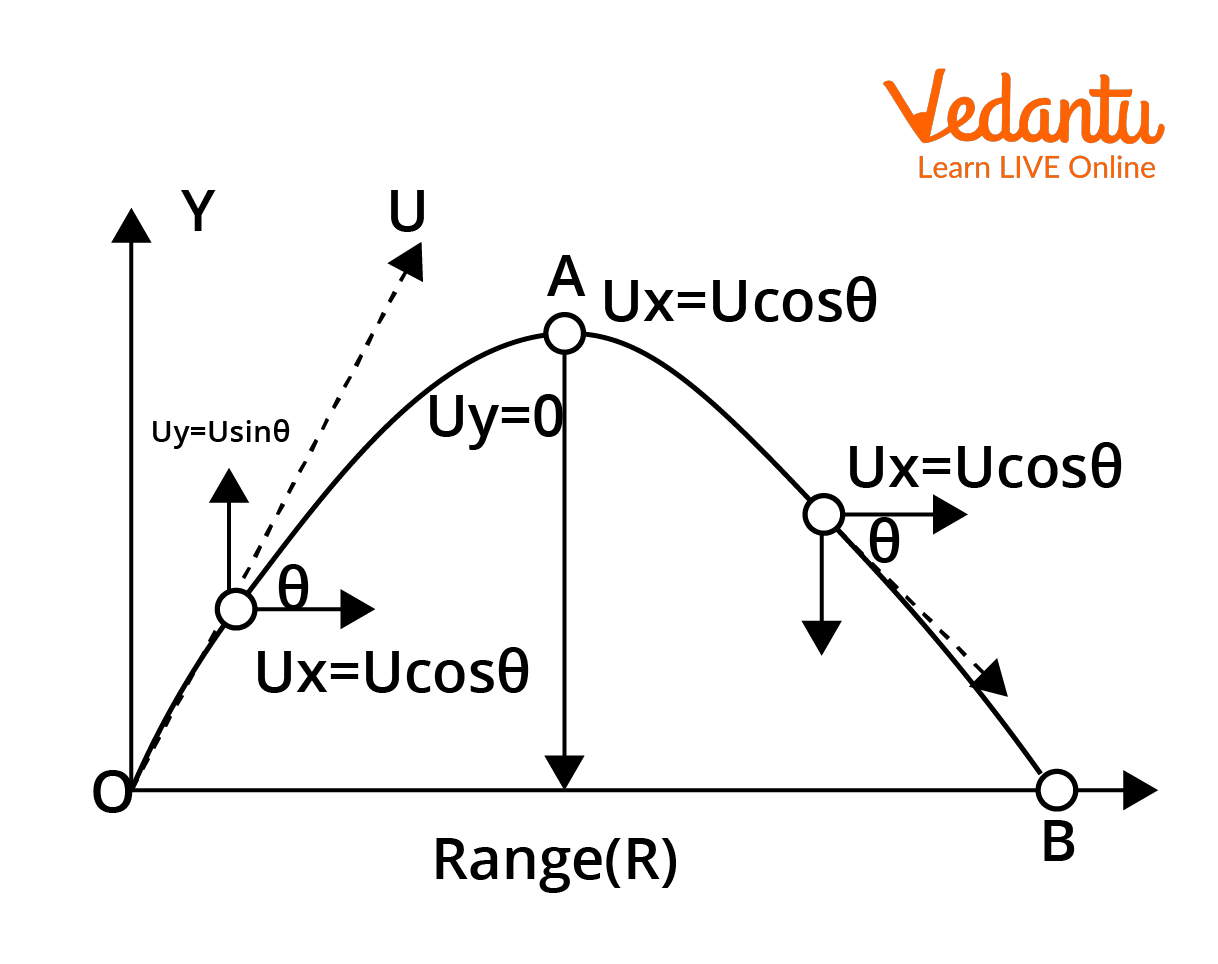
For clear understanding, see the variables below as they appear in different motion formulas:
| Symbol | Meaning | SI Unit |
|---|---|---|
| u | Initial velocity | m/s |
| θ | Angle of projection | radian (° for diagrams) |
| g | Acceleration due to gravity | m/s2 |
| x, y | Coordinates at time t | metre (m) |
Stepwise Derivation Of Equation Of Trajectory (Projectile Motion)
The Derivation Of Equation Of Trajectory follows a sequence based on the equations of motion in the x and y directions. Watch for the substitutions and how the time variable is eliminated.
- Write horizontal displacement: x = u cosθ · t
- Write vertical displacement: y = u sinθ · t – (1/2)gt2
- Solve for t from x equation: t = x/(u cosθ)
- Substitute this value of t in the y equation
- Expand and simplify; replace t2 accordingly
- You obtain: y = x tanθ – (g x2)/(2u2 cos2θ)
The final equation is:
y = x tanθ – (g x2)/(2u2 cos2θ)
Try applying this with different values for numerical practice, especially for angle θ and initial velocity u. Check out motion in 2D dimensions for more context.
Parabolic Nature: Proving the Equation of Trajectory Forms a Parabola
By rearranging, observe how the equation matches the general form of a parabola (y = ax + bx2). Thus, any object in projectile motion traces a parabolic path.
- The term x tanθ is linear (straight-line tendency)
- The –(g x2)/(2u2 cos2θ) is quadratic (parabolic), causing the curve
- No “y2” (not a circle or ellipse), confirming it’s a parabola
A typical JEE trap: forgetting the sign for the quadratic term or mishandling cosθ in denominators. Refer to graphical analysis of kinematics for more visuals.
Applications, Special Cases, and Exam Relevance of Derivation Of Equation Of Trajectory
- Horizontal projectile (θ = 0°): simplifies to y = –(g x2)/(2u2)
- Ground-to-ground: Use for total range or finding maximum height
- In sports (cricket, football), forensics, or ballistics
- Used in exam numericals for finding y at given x, or vice versa
- Essential for verifying options in quick JEE calculations
As Vedantu faculty often advise, always relate this formula to real-life projectile paths—like a baseball hit into the air or a launched rocket. Don’t forget to check your units and the angle’s quadrant when applying the formula.
Read more about the related projectile motion concepts or use the range of projectile article to strengthen your basics. For practice on solving kinematics, refer to kinematics practice paper.
- equations of motion page for each formula step
- time of flight for duration derivation tricks
- vertical projectile motion for contrast (θ = 90° case)
- graph of force vs mass to interpret motion
- friction for assumptions regarding resistance
The Derivation Of Equation Of Trajectory often appears as a 3–5 mark question or a step in numericals at JEE Main level. Typical questions may ask for the proof, application to a sample scenario, or for extracting the maximum height/range using this derived formula.
A simple example: A ball is thrown with u = 20 m/s at θ = 45°. Using g = 10 m/s2, calculate y for x = 20 m. Substituting:
tanθ = 1; cosθ = 1/√2
y = 20 × 1 – (10 × 400) / (2 × 400 × (1/2)) = 20 – (4000) / (400) = 20 – 10 = 10 m above ground.
JEE examiners like to ask for “proof of equation of trajectory” and then combine this with questions on time of flight or horizontal range.
- Initial velocity split in correct directions (cosθ, sinθ)
- No sign mix-up for gravity (downwards is negative y)
- Proper time elimination before substituting back
- Use SI units only, as with all JEE Physics
For revision, jot down:
- The definition of “trajectory” for a projectile motion
- The key formula: y = x tanθ – (g x2)/(2u2 cos2θ)
- Steps which eliminate t between x and y equations
- That the path is always a parabola (for constant g, no air)
- Common pitfalls: neglecting angle units or gravity’s sign
To reinforce the Derivation Of Equation Of Trajectory, tackle some kinematics mock test problems or check laws of motion mock test for more projectile questions. For deeper practice, consult the full JEE Main Physics question paper library on Vedantu.
In essence, once you master the Derivation Of Equation Of Trajectory, you build a stronger base for all projectile numericals and graph interpretations in JEE. Remember, focus on the stepwise substitution and stay attentive to JEE exam language and variables. For last-minute notes, download focused Physics resources and summary sheets from Vedantu’s study section.
FAQs on Equation of Trajectory in Projectile Motion: Derivation & Proof
5. How is the derivation of the equation of trajectory important for exam numericals?
The derivation is vital for solving numerical problems on projectile motion, especially in JEE Main, NEET, and board exams. Its importance includes:
- Understanding how to use formulas for maximum height, range, and time of flight.
- Solving path-based (trajectory) and position-based questions.
- Connecting conceptual theory with practical calculations.
6. What is the mathematical equation for the trajectory of a projectile?
The mathematical equation for a projectile’s trajectory is:
y = x tanθ - (g x2)/(2 u2 cos2θ).
- This shows the parabolic path followed by projectiles under gravity.
- Used widely in Class 11 Physics and JEE Main questions.
- Core to understanding projectile motion and related applications.
7. Is the equation of trajectory valid when air resistance is present?
The standard equation of trajectory assumes no air resistance and constant gravity. When air resistance is present:
- The path is no longer a perfect parabola.
- Calculations become more complex and require advanced mathematics or simulation.
- The CBSE and JEE syllabus usually ignores air resistance for basic trajectory questions.
9. Does the equation of trajectory change if the projectile is launched from a height?
Yes, the equation of trajectory modifies if projected from a height. The vertical equation includes initial height h:
y = h + x tanθ - (g x2)/(2 u2 cos2θ). This form is commonly asked in advanced questions and JEE exams.
10. How can the equation of trajectory help in real-life applications?
The equation of trajectory is crucial in real-world applications like:
- Sports (ball throwing, long jump, golf)
- Engineering (ballistics, missile trajectories)
- Animation and game design (parabolic motion of objects)
- Space missions (launch and landing predictions)
11. What are the applications of the equation of trajectory in JEE and board exam questions?
The equation of trajectory is widely used in:
- Calculating range, time of flight, and maximum height problems.
- Solving for angle or velocity when given other variables.
- Interpreting graphical questions about parabolic motion.


































