




Derivation and Formula for Electric Field on the Axis of a Charged Ring
The Electric Field Due To A Uniformly Charged Ring is a key electrostatics concept for JEE Main learners. This scenario involves a ring of fixed radius carrying a constant total charge distributed smoothly along its circumference. It’s a classic case to understand continuous charge distributions, axial symmetry, and how fields combine through superposition. The electric field at the center, at an axial point, and trends along the axis often appear in exams. With careful logic and formula derivation, you can tackle both straightforward and application-style questions with confidence.
Suppose a charged ring offers a way to visualize the effect of a continuous non-uniform source versus a point charge. Real-world parallels include charge arrangements inside cathode ray tubes and in advanced sensors. You must master axis formulas, graphs, and careful reasoning for reliable MCQ accuracy and conceptual clarity.
Concept of Electric Field Due To A Uniformly Charged Ring
In this system, the ring has total charge Q spread evenly, and radius R. We study the electric field at any point along the central axis, at the ring’s center, or at far-off locations. The field components perpendicular to the axis cancel due to symmetry, leaving only the axial component. This symmetry is crucial for simplifying complex integrals in electrostatics. Comparing with other sources like wires or discs sharpens your exam toolkit.
Axis-based sources like the electric field due to infinite linear charge exploit this same logic. The ring’s field depends on distance from center (x) and the system’s total geometry.
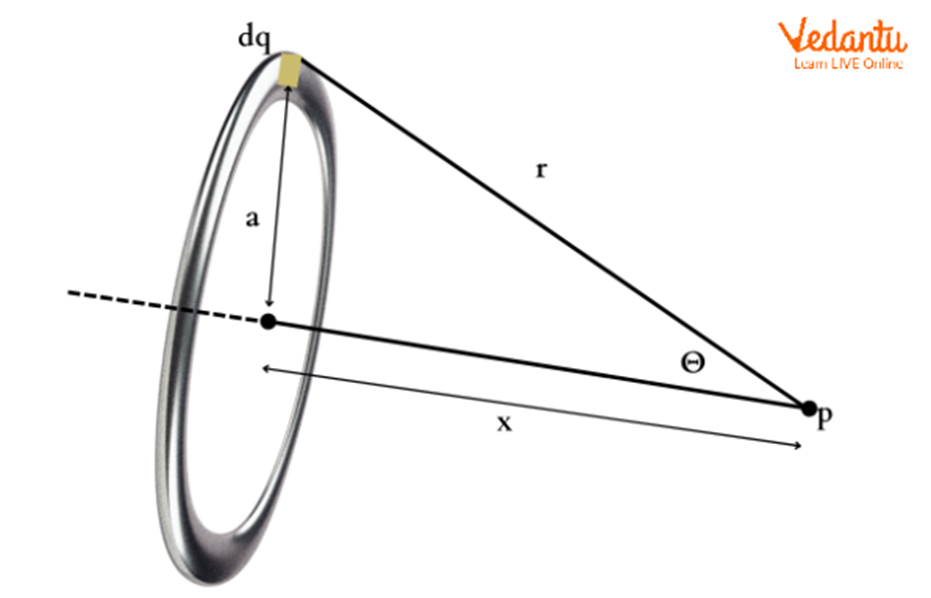
Derivation and Formula for Electric Field Due To A Uniformly Charged Ring
Let the axis of the ring pass through its center and observe a point at distance x from the center. Assume total ring charge Q distributed over length 2πR. Linear charge density is λ = Q/2πR (λ is charge per unit length). At the observation point, each ring element creates a field vector, but only the component along the axis (x-direction) adds up. Perpendicular parts cancel by symmetry.
- Place the ring in the yz-plane, center at origin, axis is x-axis.
- Take a small segment dq = λ Rdθ, located at angle θ.
- Distance from this dq to observation point is r = √(R² + x²).
- Field from dq is dE = (1/4πε0)(dq/r²).
- Only x-component remains: dEx = dE·cosα = dE·x/r.
- Integrate over θ from 0 to 2π (full ring): net field is sum of all dEx.
On integrating, we get the main result for the electric field at a point on the axis:
E = [1/(4πε0)] × (Qx) / (R² + x²)3/2
where E is the field at distance x on the axis, Q is the total charge, and R is the ring’s radius.
At the center (x = 0), the numerator vanishes: the electric field at the ring’s center is zero. For very large x (far away), the formula reduces to that for a point charge, since the denominator dominates: E ≈ (1/4πε0) Q/x².
Key Formulas and Special Results for Electric Field Due To A Uniformly Charged Ring
| Scenario | Formula | Remarks |
|---|---|---|
| At an axial point (distance x) | E = [1/(4πε0)] × (Qx)/(R² + x²)3/2 | Direction along axis; field points away for +Q |
| At center (x = 0) | E = 0 | Perfect cancellation by symmetry |
| Far away (x >> R) | E ≈ [1/(4πε0)] Q/x² | Behaves like point charge |
Always check the denominator: (R2 + x2)3/2. This controls how quickly the field falls off with distance. Mistakenly omitting the 3/2 power or the x numerator is a common error to avoid.
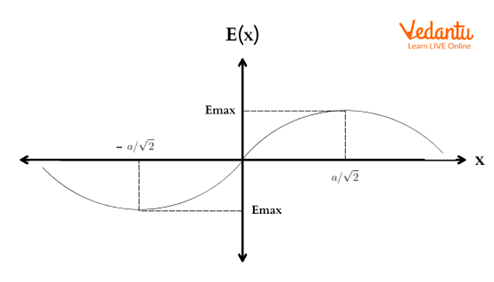
Graphical Trends and Practical Applications
The field is zero at the center, rises to a maximum at some point x ≈ R/√2, then falls off at greater distances. The curve is symmetric about the center. MCQs may ask for the distance where E is maximum; differentiate E(x) with respect to x, set derivative zero and solve for x.
- Cathode ray tube focusing uses charged rings for beam control.
- Particle accelerator elements use this principle for trajectory adjustment.
- Charged ring fields appear as “building blocks” for more complex field distributions, like discs or spheres.
- Often tested in JEE practice on superposition—combining field due to multiple sources along an axis.
- Exam caution: never apply Gauss’s law for ring symmetry directly; this situation lacks enough symmetry for that method.
Compare with topics like gauss law or electric field due to infinite plane to understand where ring symmetry is unique.
Worked Example: Solving for Electric Field On the Axis
A uniformly charged ring has radius 0.20 m and charge 6.0 μC. What is the electric field at a point on the axis, 0.20 m from the center? Use ε0 = 8.85 × 10-12 C2N-1m-2.
- Here, Q = 6.0 × 10-6 C, R = 0.20 m, x = 0.20 m.
- E = [1/(4πε0)] × (Qx) / (R² + x²)3/2
- (R² + x²) = (0.20)² + (0.20)² = 0.08; (R² + x²)3/2 = (0.08)3/2 ≈ 0.0226
- E = (9 × 109) × (6 × 10-6 × 0.20)/(0.0226)
- E ≈ (9 × 109 × 1.2 × 10-6)/(0.0226) ≈ 4.78 × 105 N/C (along the axis)
Always express the direction relative to the ring. For positive charge, field points away from the ring along the axis. Note the formula’s resemblance to coulombs law for a point charge at large distances.
Comparison: Electric Field Due To A Uniformly Charged Ring and Other Charge Distributions
| Distribution | Key Formula | Center Field | Field at Large x |
|---|---|---|---|
| Uniformly charged ring | E = [1/(4πε0)] (Qx)/(R2 + x2)3/2 | 0 | Like point charge |
| Infinite straight wire | E = λ/(2πε0r) | Infinite at wire | Falls more slowly |
Mastering these contrasts helps with linked JEE questions. Review materials like electric field lines and charge in a magnetic field for system-specific field directions.
- Uniformly charged ring: field zero at center; all field lies along axis.
- Infinite wire: field is azimuthal, strength never zero at any finite r.
- Disc: center has nonzero field; edge effects matter.
- Sphere: internal field has unique pattern, needs gauss law for easy computation.
Rings also help you understand limiting cases—a dipole field emerges when observing very far away from the ring plane.
JEE aspirants should practice variations and graphs using Vedantu’s topic pages and MCQ banks:
- electric field due to a uniformly charged ring concept and examples for strong foundation.
- coulombs law for point charge comparison.
- gauss law for symmetrical charge bodies.
- electric field due to infinite plane for plane symmetry.
- electric field lines visualization and properties.
- electric field due to infinite linear charge and cylinders for axial contrasts.
- electric dipole for distant-approximation logic.
- electrostatics practice paper to self-test understanding.
- electrostatics mock test 2 for MCQ drills.
- preparation tips for JEE Main Physics exam mastery.
- electrostatics revision notes for last-minute summaries.
- electric potential due to charge distributions.
With practice and clear diagrams, JEE Main concepts like the Electric Field Due To A Uniformly Charged Ring can be solved quickly and confidently. For detailed stepwise theory, worked numericals, and high-quality mocks, Vedantu remains an excellent learning partner.
FAQs on Electric Field Due to a Uniformly Charged Ring Explained
1. What is the electric field due to a uniformly charged ring at a point on its axis?
The electric field at a point on the axis of a uniformly charged ring is given by a specific formula derived from electrostatics principles. For a ring of radius R, carrying total charge Q, the electric field at distance x from the center (along the axis) is:
E = [1/(4πε₀)] × (Qx) / (R² + x²)3/2
Main points:
- This formula shows the electric field is maximum at a certain distance from the center, not at the center itself.
- Direction is along the axis, pointing away from the ring if charge is positive.
- It is an important application of the superposition principle in electrostatics and is frequently asked in JEE Main/NEET exams.
2. How do you derive the electric field due to a uniformly charged ring?
To derive the electric field of a uniformly charged ring at a point on its axis:
- Consider a ring of radius R and total charge Q distributed uniformly.
- Pick a small charge element dq on the ring.
- Calculate the contribution of dq at the axial point—due to symmetry, only the axial components sum up.
- Integrate these contributions over the whole ring using Coulomb's law.
- The final result is E = [1/(4πε₀)] × (Qx) / (R² + x²)3/2.
3. What is the electric field at the centre of a uniformly charged ring?
The electric field at the exact center of a uniformly charged ring is always zero.
Reason:
- At the center, contributions from all parts of the ring are equal in magnitude but opposite in direction.
- By symmetry, these cancel each other out, resulting in net electric field = 0 at the center.
4. How does the electric field vary along the axis of a charged ring?
The electric field along the axis of a uniformly charged ring:
- Is zero at the center (x = 0).
- Increases with distance from the center, reaches a maximum, then decreases as distance increases further.
- At very large distances, the field decreases as 1/x2 and behaves like a point charge.
5. What is the formula for the electric field due to a uniformly charged ring at an axial point?
The formula for the electric field at an axial point of a uniformly charged ring is:
E = (1/4πε₀) × (Qx) / (R² + x²)3/2
Where:
- E: electric field at a distance x from the center along the axis
- Q: total charge on the ring
- R: radius of the ring
- x: axial distance from center
6. Why is the electric field at the geometric center of a uniformly charged ring zero?
The electric field at the center of a uniformly charged ring is zero due to symmetry:
- Every small charge element on the ring has an opposite element directly across the ring.
- Their electric fields at the center cancel out pairwise.
7. What is the difference between the electric field due to a ring and an infinite wire?
The electric field due to a ring and an infinite wire differ in their geometry and field patterns:
- Ring: Field is strongest at a certain point on the axis; zero at center; decreases at large distances like a point charge.
- Infinite wire: Field is radial, decreases as 1/r, and is uniform along the wire’s length.
8. How does the electric field from a ring behave at very far distances?
At very large distances (x ≫ R), the electric field due to a charged ring acts like the field from a point charge:
- The formula reduces to E ≈ [1/(4πε₀)] × Q / x².
- This is identical to the field of a point charge situated at the center of the ring.
9. Can the formula for electric field due to a uniformly charged ring be used for non-uniform rings?
No, the standard formula applies only if the ring is uniformly charged.
For a non-uniform charge distribution:
- The field must be calculated by integrating the varying dq (small charge elements) for the actual charge density.
- This requires a modified approach using calculus and precise knowledge of the charge variation around the ring.
10. What are some applications of the electric field due to a uniformly charged ring?
The concept of electric field from a uniformly charged ring is used in many practical and exam-relevant scenarios:
- Fundamental for understanding particle motion in devices like cyclotrons or CRTs (cathode ray tubes).
- Used to model fields in circular accelerators and certain sensors.
- Important for MCQs and numericals in JEE Main, NEET, CBSE and competitive physics exams.
11. What is the direction of the electric field produced by a uniformly charged ring on its axis?
The electric field produced by a uniformly charged ring at any point on its axis is directed along the axis of the ring.
- If the ring’s charge is positive, the field points away from the ring; if negative, towards the ring.
- This direction remains consistent on both sides of the central plane.
12. How can I apply the formula for electric field due to a ring to solve JEE or NEET numericals?
To use the electric field due to a ring in numericals:
- Identify or assign variables: Q (charge), R (radius), x (distance from center on axis).
- Use the standard formula: E = (1/4πε₀) × (Qx)/(R² + x²)3/2.
- Insert quantities with correct units (Coulombs, meters).
- Pay attention to sign and direction, especially in vector-based MCQs.
































