Important Practice Problems for CBSE Class 7 Maths Chapter 9: Perimeter and Area FREE PDF
Very Short Answer Questions (1 – 5) 1 Marks
1. Write the formula to find the area of the triangle.
Ans: The formula to find the area of triangle is
A=$\frac{1}{2}bh$
where, b= Base of triangle
h= Height of triangle
2. Write the formula to find the circumference of a circle.
Ans: The formula to find the circumference of a circle is
C=$2\pi r$
Where, C= Circumference of circle
r= Radius of circle
3. Write the formula to find the area of the circle.
Ans: The formula to find the area of circle is
A=$\pi {{r}^{2}}$
where, A= Area of circle
r= Radius of circle
4. Write the formula to find the area and perimeter of a rectangle.
Ans: The formula to find the area and perimeter of a rectangle are
Area of rectangle=$l\times b$
Perimeter of rectangle=$2(l+b)$
where, l= Length of rectangle
b= Breadth of rectangle
5. The distance around a circular region is known as its_______.
Ans: Circumference
Short Answer Questions (6 – 15) 2 Marks
6. Find the area of a square park whose perimeter is $480$m.
Ans: Given, Perimeter(P)= $480$m
s=Side of square
P= $4\times s$
$480$= $4\times s$
s=$\frac{480}{4}$
s=$120$m
Area of Square=s2
=${{(120)}^{2}}$
=$14,400$sq.m
7. If the perimeter of a rectangle is $390$cm and the length is $30$cm. Find its breadth in the area.
Ans: Given, Perimeter= $390$cm , length=$30$cm
B= Breadth
A= Area
P=$2(l+b)$
$390=2(30+b)$
$\frac{390}{2}=(30+b)$
$195=(30+b)$
$b=195-30$
$b=165$cm
A=$l\times b$
A=$30\times 165$
A=$4,950$sq.cm
8. A wire bent in the shape of a rectangle. Its length is $30$cm and breadth is $15$cm and if the same wire is rebent in the shape of a square. What will be the measure of slides and which encloses more area.
Ans: We know, perimeter of rectangle
P=$2(l+b)$
Where, l=length and b = breadth
=$2(30+15)$
=$2(45)$
=$90$cm
Perimeter of Square= Perimeter of rectangle
$90=4s$ [s=side]
$s=\frac{90}{4}$
$s=22.5$cm
Area of rectangle = $l\times b$
= $30\times 15$
=$450$sq. cm
Area of Square=s2
=${{(22.5)}^{2}}$
=$506.25$sq.cm
Hence, Square encloses a large area.
9. Find the area of
Ans: Given
height=$3$cm
breadth=$6$cm
Area of parallelogram = $b\times h$
= $6\times 3$
= $18$cm2
10. Find the area of
Ans: Given base=$5.5$cm , height=$2.2$cm
Area of triangle = $\frac{1}{2}\times b\times h$
= $\frac{1}{2}\times 5.5\times 2.2$
= $6.05$sq. cm
11. $\Delta ABC$ is isosceles with AB = AC = $5.5$cm and BC = $8$cm. What will be the height from C to AB i.e., CE? If the height AD from A to BC is $4.5$cm. Find the area of $\Delta ABC$?
Ans: Given BC=base=$8$cm , AD=heigth=$4.5$cm
Area of $\Delta ABC$ = $\frac{1}{2}\times b\times h$
= $\frac{1}{2}\times 8\times 4.5$
= $18$sq.cm
Area of $\Delta ABC$ = $\frac{1}{2}\times b\times h$
= $\frac{1}{2}\times AB\times CE$
$18$=$\frac{1}{2}\times 5.5\times CE$
CE=$\frac{36}{5.5}$
CE=$6.55$cm
12. If the circumference of a circular sheet is $132$cm. Find its radius and area.
Ans: Given, Circumference=$132$cm
$C=2\pi r$ [r=radius]
$132=2\times \frac{22}{7}\times r$
$r=\frac{132\times 7}{2\times 22}$
$r=21$cm
Area=$\pi {{r}^{2}}$
Area=$\frac{22}{7}\times {{(21)}^{2}}$
Area=$66\times 21$
Area=$1386$sq. cm
13. Find the circumference and area of the circle if radius is $7$cm.
Ans: Given, radius=$7$cm
$C=2\pi r$ [C=Circumference]
$C=2\times \frac{22}{7}\times 7$
$C=44$cm
Area=$\pi {{r}^{2}}$
Area=\[\frac{22}{7}\times {{(7)}^{2}}\]
Area=$22\times 7$
Area=$154$sq. cm
14. A garden is $50$m long and $42$m broad. A path of $2$m wide is built outside and around it. Find the area of the path in hectares?
Ans: Given,
Length of garden=$50$m
Breadth of garden =$42$m
Area of garden = $l\times b$
= $50\times 42$
=$2100$sq. m
Area of garden where path is included
Area of garden = $l\times b$
= $54\times 46$
=$2484$sq. m
Area of path = Area of garden including path – Area of garden
=$2484-2100$
= $384$sq.m
$1$hectare = $10000$m2
Area of garden= $\frac{384}{10000}$
= $0.0384$hectare
15. Find the area and perimeter of the square whose side is $4$cm.
Ans: We know,
Area of square= ${{s}^{2}}$ [s=side]
=${{4}^{2}}$
=$16$sq. cm
Perimeter = $4s$
=$4\times 4$
=$16$cm
Long Answer Questions (16 – 20) 3 Marks
16. The length and breadth of a rectangular piece of land are $350$m and $150$m respectively.
Find
a. The area
Ans: We know,
Area of garden = $l\times b$
l=length
b= Breadth
= $350\times 150$
=$52,500$sq. m
b. The cost of the land, if $1{{m}^{2}}$ of the land costs Rs. $10,000$
Ans: Given, Cost of $1{{m}^{2}}$of land = Rs. $10,000$
Cost of $52,500{{m}^{2}}$of land = Rs. $52,500\times 10,000$
=Rs. $52,50,00,000$
17. A rectangular field of length $50$m and breadth $45$m need to be fenced. Find the cost of fencing if the changes are Rs. $4$ per metre.
Ans: We know, perimeter of rectangle
P=$2(l+b)$
l=length
b= Breadth
=$2(50+45)$
=$2(95)$
=$190$m
Cost of fencing= $190\times 4$
= Rs. $760$
18. $\Delta ABC$ is right angles at A, AD BC ⊥ .If AB = $8$cm, BC = $17$cm and AC = $15$cm. Find the area of ABC and also length of AD.
Ans: Here,
Area of $\Delta ABC$ = $\frac{1}{2}\times b\times h$
b= base
h=height
=$\frac{1}{2}\times AC\times AB$
= $\frac{1}{2}\times 8\times 15$
= $60$sq.cm
Area of $\Delta ABC$ = $\frac{1}{2}\times b\times h$
= $\frac{1}{2}\times BC\times AD$
$60$=$\frac{1}{2}\times 17\times AD$
AD=$7.03$cm
19. Find the area and circumference of the circle whose radius is
a. $2$cm
Ans: Given, radius=$2$cm
$C=2\pi r$ [C=Circumference]
$C=2\times \frac{22}{7}\times 2$
$C=\frac{88}{7}$cm
Area=$\pi {{r}^{2}}$
Area=\[\frac{22}{7}\times {{(2)}^{2}}\]
Area=$12\frac{4}{7}$sq.cm
b. $21$cm
Ans: Given, radius=$21$cm
$C=2\pi r$
$C=2\times \frac{22}{7}\times 21$
$C=132$cm
Area=$\pi {{r}^{2}}$
Area=\[\frac{22}{7}\times {{(21)}^{2}}\]
Area=$66\times 21$
Area=$1386$sq. cm
20. Find the area of quadrilateral ABCD here AC = $20$cm, BM = $4$cm, DN = $4$cm and BM $\bot $AC and DN $\bot $AC .
Ans: Using formula
Area of $\Delta ABC$ = $\frac{1}{2}\times b\times h$
B= Base
H=Height
= $\frac{1}{2}\times 4\times 20$
= $40$sq.cm
Area of $\Delta ADC$ = $\frac{1}{2}\times b\times h$
=$\frac{1}{2}\times 20\times 4$
= $40$sq.cm
Area of quadrilateral ABCD = Area of $\Delta ABC$+ Area of $\Delta ADC$
=$40+40$
=$80$sq. cm
5 Important Formulas of Class 7 Chapter 9 Perimeter and Area You Shouldn’t Miss!
Chapter 9, "Perimeter and Area," introduces important formulas that help calculate the boundary (perimeter) and the space inside (area) of various shapes. Here are five key formulas you should know:
1. Perimeter of a Rectangle
To find the total boundary length of a rectangle:
$ \text{Perimeter} = 2 \times (\text{Length} + \text{Breadth}) $
2. Area of a Rectangle
To calculate the space inside a rectangle:
$\text{Area} = \text{Length} \times \text{Breadth}$
3. Perimeter of a Square
Since all sides of a square are equal, the perimeter is:
$\text{Perimeter} = 4 \times \text{Side}$
4. Area of a Square
The area of a square, or the space inside it, is:
$\text{Area} = \text{Side} \times \text{Side} = \text{Side}^2$
5. Area of a Triangle
To find the area of a triangle when the base and height are known:
$\text{Area} = \dfrac{1}{2} \times \text{Base} \times \text{Height}$
Benefits of Important Questions for Chapter 9 Perimeter and Area Class 7 Maths
Important Questions for Chapter 9, "Perimeter and Area," helps students learn how to calculate the perimeter (boundary length) and area (space inside) of various shapes like rectangles, squares, triangles, and circles. These are foundational concepts used in geometry.
This FREE PDF offers a range of important practice problems. By working through these problems, students can better understand and apply the formulas for calculating the perimeter and area, making it easier to solve related questions in exams and daily life.
The problems in this PDF are designed according to the latest CBSE syllabus. This ensures that students cover the topics they need to know and practice questions that are likely to appear in their exams.
Students can download the PDF for FREE from Vedantu’s Landing Page and access it anytime. This makes it easy to study at their own pace and revisit important questions whenever they need a quick revision.
Regular practice with these questions prepares students thoroughly for exams by reinforcing key concepts and improving their calculation skills for perimeter and area problems.
Conclusion
Perimeter and Area is an integral part of Class 7 Maths and plays a crucial role from an examination perspective. The important questions for Class 7 Maths, cover a wide range of topics within the subject. They also provide a concise guide to critical points and details related to the topic.
A solid understanding of each section of Class 7 Maths is fundamental as it forms the basis for higher-level studies. However, this section primarily focuses on important questions within the context of Class 7 Maths.
Related Study Materials for Class 7 Maths Chapter 9 Perimeter and Area
Chapter-wise Important Questions Links for Class 7 Maths
Important Study Materials for Class 7 Maths
FAQs on CBSE Class 7 Maths Important Questions Chapter 9 - Perimeter and Area
1. How can I benefit from practising Maths Important Questions?
The examination pattern is divided into four sections- Section A, B, C, and D. To solve questions from each section you need to practise a lot of questions regularly. Practising important questions will make you familiar with the type of questions. If you are well versed with the concepts you will be easily able to solve direct questions.
To solve word problems you will need to convert them into solvable equations first before you attempt them. Practising important questions will give you the confidence to solve any difficult question that comes in the examination.
One great way to practise important questions from each chapter is to solve sample papers. If you solve sample papers under the stipulated time it will benefit you because you will be familiar with working under time pressure. Focus on understanding the concept of the important questions and the pattern they follow.
2. What is the difference between perimeter and area?
The perimeter of a closed figure is the distance around it, whereas its area is the fraction of a plane or region it encloses. A regular polygon's perimeter is equal to the number of sides multiplied by the length of one side. Similarly, the perimeter and area of various forms vary and are calculated using different formulae based on this concept. Perimeter and area are important concepts of maths and have significant weightage in the exam. You can prepare them well with Vedantu.
3. How can I download the PDF file for the Solutions of NCERT Class 7 Maths Chapter 9?
The solutions are easily available on the Vedantu site.
Click on this link
The webpage with Vedantu’s solutions for Class 7 Maths
Chapter 9 will open.
To download this, click on the Download PDF button and you can view the solutions offline.
The Vedantu website has a variety of instructors that can aid you with this or any other chapter.
4. What is an irregular shape Class 7?
Any size and length is a possibility for an irregular form. A kite, a diamond shape, a leaf, and other irregular forms may be seen all around us. An irregular form is defined as one whose sides and angles are not of equal length. Rocks, prisms, beans, and screws are examples of irregular solids. Each of these things has a unique irregular form that isn't made up of regular shapes. The difficulty to estimate an irregular solid area, volume, or mass by measuring its length, breadth, and height distinguishes it.
5. What is the difference between irregular and regular shapes?
An irregular form can be any size and length, whereas a regular shape has equal sides and angles. Thus, the area of a regular shape may be calculated directly using appropriate formulae, but the area of an irregular shape can be calculated by decomposing an irregular shape into numerous regular ones. Irregular shapes’ areas are generally harder to calculate due to their unpredictability. Hence, an approach different from the norm, while calculating the area of regular shapes, has to be taken for irregular shapes.
6. How do you find areas of irregular shapes?
To calculate the area of an irregular form, first split it into regular shapes that you can identify, such as triangles, rectangles, circles, squares, and so on. Then, to create an area of irregular forms, determine the area of each individual shape and put them together. Due to their unpredictability, the areas of irregular forms are typically more difficult to compute. For more problems regarding this, you can visit the Vedantu site.
7. How does knowing perimeter and area help in real life?
Perimeter and area are useful for things like measuring land, building walls, painting surfaces, and even in designing spaces like gardens and rooms.
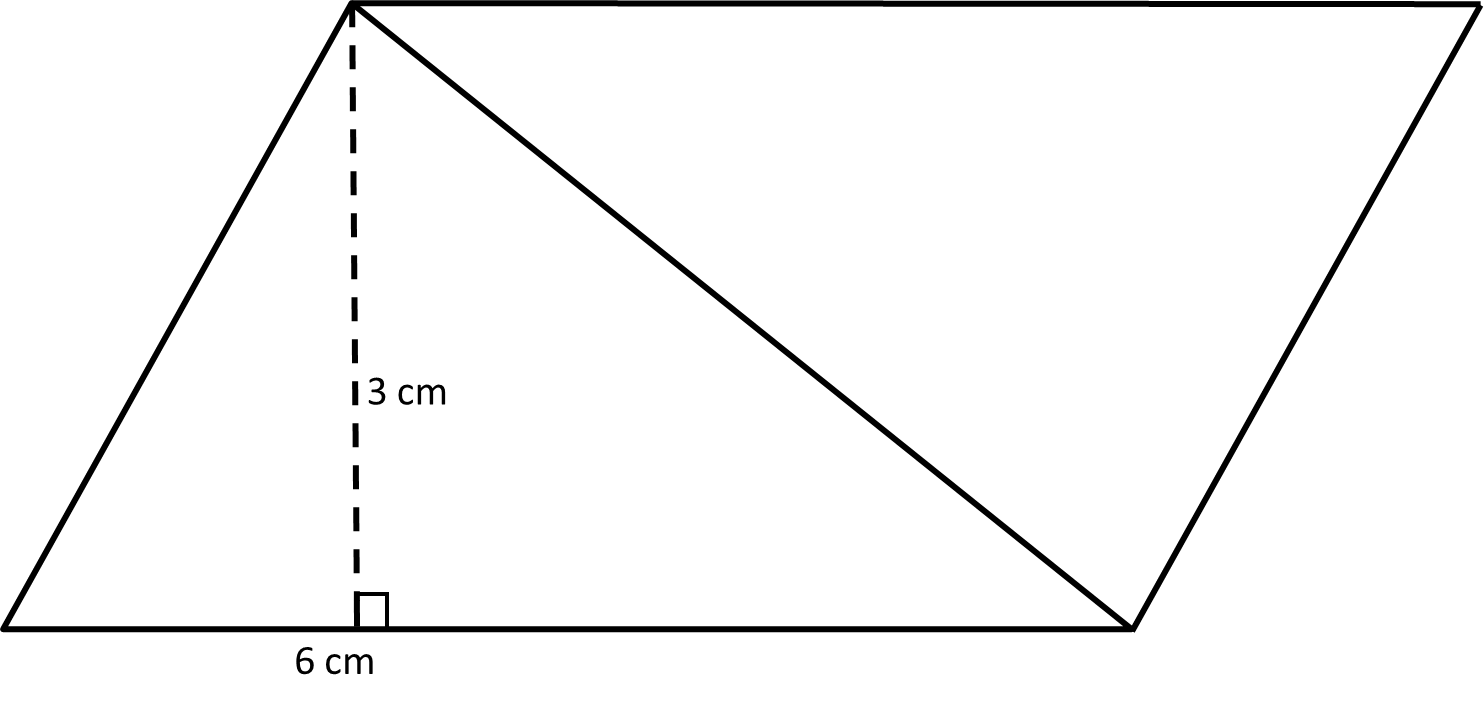
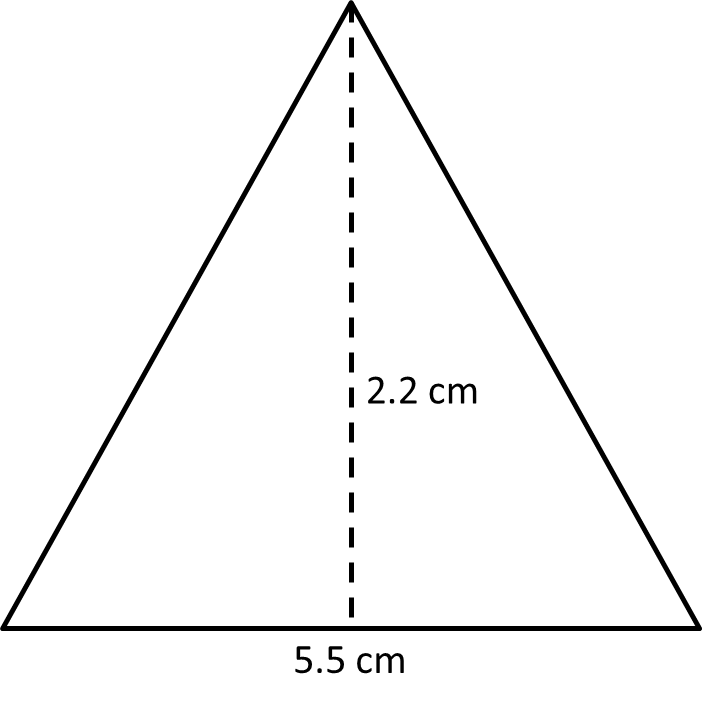
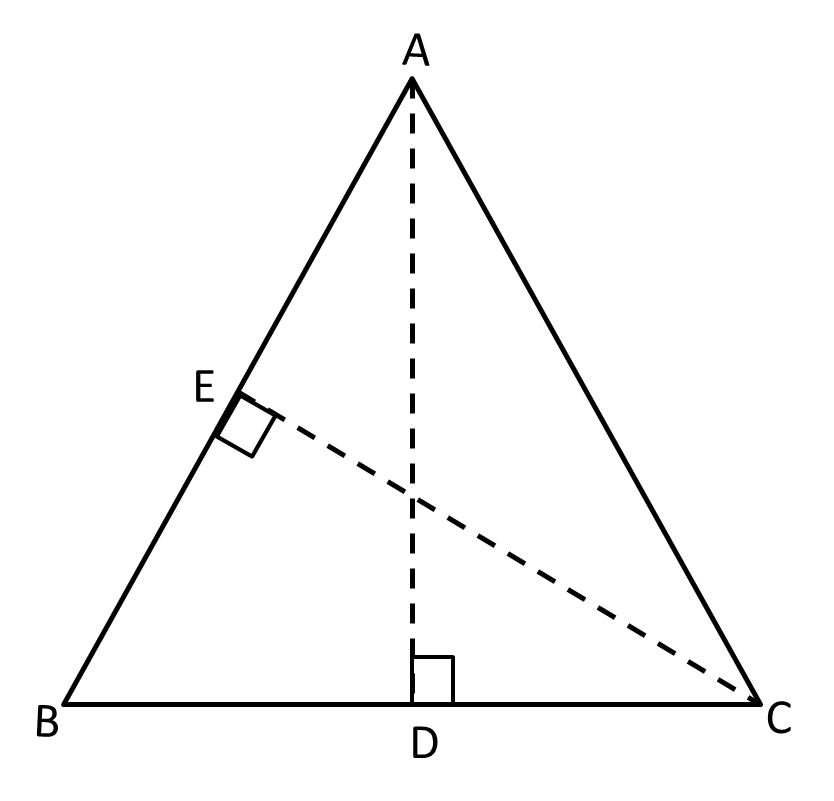
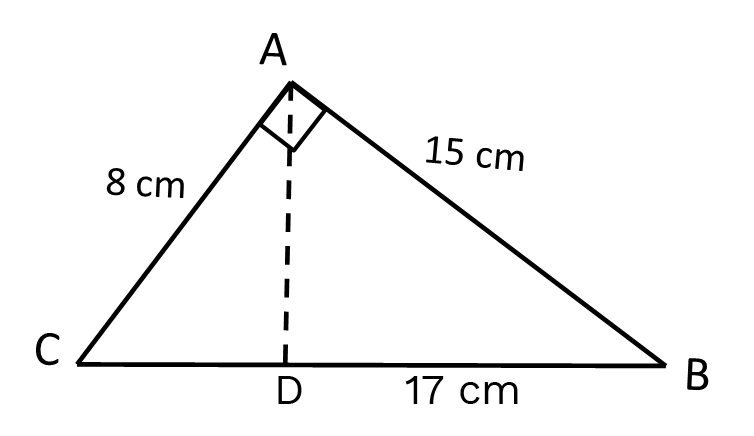
8. What is the formula for finding the area of a triangle?
The area of a triangle is calculated using the formula $\dfrac{1}{2} \times \text{Base} \times \text{Height}$.
9. Why is it important to learn different formulas for area and perimeter?
Each shape has a unique formula for perimeter and area. Learning these helps you solve problems related to different shapes accurately.
10. How can I remember all the formulas for perimeter and area?
Regular practice and using the formulas in solving questions will help you remember them. Writing them down and revising them frequently also helps.
11. How can practising perimeter and area questions improve my maths skills?
Practising these questions improves calculation skills, problem-solving ability, and helps you understand geometric measurements, which are useful in many real-life situations.
