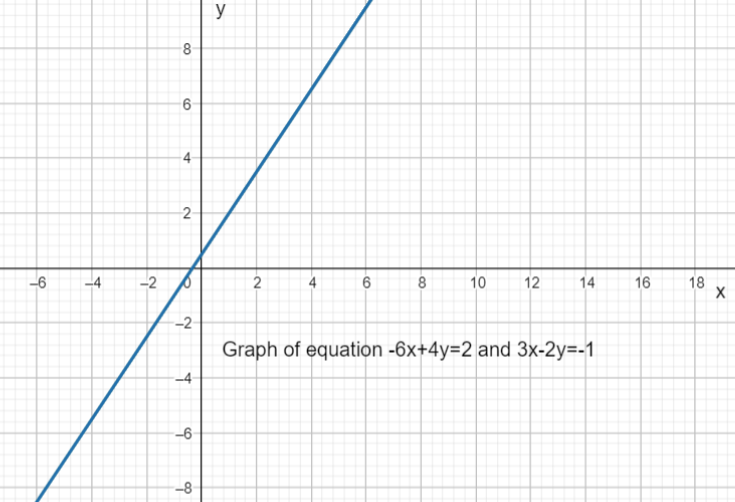
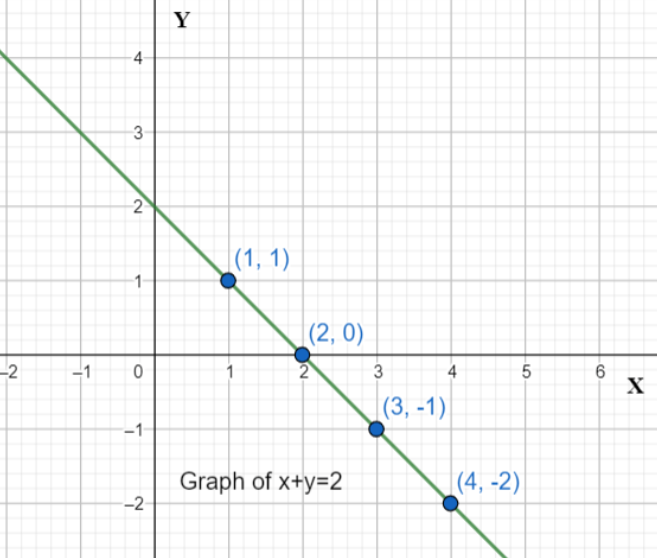
Download Revision Notes for Class 9 Maths Chapter 4 Linear Equations in Two Variables - Free PDF
FAQs on Revision Notes for CBSE Class 9 Maths Chapter 4 - Linear Equations in Two Variables
1. What are the key concepts to revise in Class 9 Maths Chapter 4 – Linear Equations in Two Variables?
The key concepts to focus on during revision are:
- Definition and standard form of a linear equation in two variables (ax + by + c = 0)
- Understanding what constitutes a solution (ordered pair) of a linear equation
- Graphical representation of linear equations in two variables
- Special cases: lines parallel to the x-axis and y-axis
2. How does the solution set of a linear equation in two variables differ from that of a linear equation in one variable?
A linear equation in one variable has exactly one solution, representing a unique value on the number line. In contrast, a linear equation in two variables has infinitely many solutions, represented by all the ordered pairs (x, y) that satisfy the equation, forming a straight line in the coordinate plane.
3. What is the quickest method to verify if a pair of values is a solution to a given linear equation in two variables?
To verify whether (x, y) is a solution to an equation like ax + by + c = 0, substitute the values of x and y into the equation. If both sides become equal, the pair is a solution; if not, it is not a solution. This method helps in quick checking during revision.
4. How can the concepts in this chapter be summarized for rapid last-minute revision?
For fast revision:
- Recall that the general form is ax + by + c = 0.
- Remember that the graph of the equation is always a straight line.
- Two variables allow for infinite solutions forming a line, unlike a single solution in one variable.
- Practice with ordered pairs and plotting points on the graph.
- Identify the special cases: parallel to axes (y = k, x = h).
5. Why is understanding the graphical interpretation of linear equations in two variables important for exams?
Graphical interpretation forms the basis of several higher-level topics in mathematics. Being able to draw and interpret lines on the coordinate plane helps in topics like coordinate geometry, solution of simultaneous equations, and understanding slopes. It also makes solving word problems easier and aligns with CBSE exam trends where graphical questions carry significant weightage.
6. What are the most common mistakes students make when revising this chapter, and how can they be avoided?
Students often:
- Confuse the solution of equations in two variables with that in one variable.
- Make calculation errors when substituting values.
- Misinterpret parallel lines or axes equations.
7. How are the equations of lines parallel to the x-axis or y-axis represented, and what should be remembered for quick recognition?
Lines parallel to the x-axis are of the form y = k, while lines parallel to the y-axis are x = h, where k and h are constants. Remember that the equation y = 0 represents the x-axis and x = 0 represents the y-axis for quick identification in exams.
8. In what order should I revise the topics within Linear Equations in Two Variables for best outcomes?
Start with the definition and standard form, move to finding solutions using substitution, then practice graphical plotting of the solutions. After that, focus on lines parallel to axes and finally do practice questions and past paper problems. This sequence builds understanding step by step.
9. How do concept maps or summary notes help in quick revision for this chapter?
Concept maps visually summarise the relationships among key ideas, such as forms of linear equations, solution methods, and graph interpretation. They enable you to see the 'big picture', making it easier to recall and connect concepts during the exam.
10. What types of questions should I expect in the final exam from Class 9 Linear Equations in Two Variables based on the CBSE 2025–26 syllabus?
You can expect questions such as:
- Definition and identification of linear equations in two variables
- Finding and verifying solutions (ordered pairs)
- Plotting graphs for given equations
- Interpreting lines parallel to axes and their equations
- Scenario-based conceptual questions on the application of linear equations