
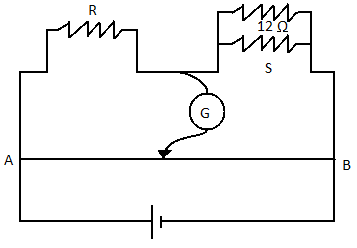
In a metre bridge the null point is found at a distance of 40cm from $A$. If a resistance of $12\Omega $ is connected in parallel with $S$, the null point occurs at $50cm$ from $A$. Determine value of $R$ and $S$.
Answer
180.3k+ views
Hint: Here two conditions are given, in one condition a resistance of $12\Omega $ is connected in parallel to $S$ and in another condition there is no such resistance connected in parallel. Applying the condition of Wheatstone bridge in these two conditions will give two different linear equations in form of variables of $R$ and $S$ . After solving the two equations you will get the answer.
Complete step by step solution:
Here in this question two conditions are given,
First, when a resistance of $12\Omega $ is not connected in parallel to $S$ . In that case we can write,
$\dfrac{R}{S} = \dfrac{{{l_1}}}{{(100 - {l_1})}}$
Putting ${l_1} = 40cm$ as given in the question we have,
$\dfrac{R}{S} = \dfrac{{40}}{{100 - 40}}$
So the relation between $R$ and $S$ is given by,
$R = \dfrac{2}{3}S$
Now case second when $12\Omega $ resistance is connected in parallel to the resistor $S$ .
In this case the effective resistance can be written as,
$\dfrac{1}{{{S_1}}} = \dfrac{1}{S} + \dfrac{1}{{12}}$
On simplifying this expression we have,
$\dfrac{1}{{{S_1}}} = \dfrac{{12 + 5}}{{12S}}$
Taking reciprocals on both sides we have,
${S_1} = \dfrac{{12S}}{{(12 + 5)}}$
Now writing the condition of Wheatstone bridge we have,
$\dfrac{R}{{{S_1}}} = \dfrac{{{l'}}}{{100 - {l'}}}$
Putting the expression for ${S_1}$ we have,
$R = \dfrac{{12S}}{{(12 + S)}} \times \dfrac{{50}}{{50}}$
On simplifying the above expression we have,
$R = \dfrac{{12S}}{{12 + S}}$
Now we have two expressions for $R$ after equating them we have,
$\dfrac{2}{3}S = \dfrac{{12S}}{{(12 + S)}}$
On simplifying the above expression we have,
$12 + S = 18$
So we have, $S = 6\Omega $
So we have $R = \dfrac{2}{3} \times 6 = 4\Omega $
So, the values of $R$ and $S$ are $4\Omega $ and $6\Omega $ respectively.
Note: It is important to note the working principle of a meter bridge. A meter bridge is an instrument that works on the principle of a Wheatstone bridge. A meter bridge is used in finding the unknown resistance of a conductor as that of in a Wheatstone bridge. The null point of a Wheatstone is also known as the balance point of the Wheatstone bridge.
Complete step by step solution:
Here in this question two conditions are given,
First, when a resistance of $12\Omega $ is not connected in parallel to $S$ . In that case we can write,
$\dfrac{R}{S} = \dfrac{{{l_1}}}{{(100 - {l_1})}}$
Putting ${l_1} = 40cm$ as given in the question we have,
$\dfrac{R}{S} = \dfrac{{40}}{{100 - 40}}$
So the relation between $R$ and $S$ is given by,
$R = \dfrac{2}{3}S$
Now case second when $12\Omega $ resistance is connected in parallel to the resistor $S$ .
In this case the effective resistance can be written as,
$\dfrac{1}{{{S_1}}} = \dfrac{1}{S} + \dfrac{1}{{12}}$
On simplifying this expression we have,
$\dfrac{1}{{{S_1}}} = \dfrac{{12 + 5}}{{12S}}$
Taking reciprocals on both sides we have,
${S_1} = \dfrac{{12S}}{{(12 + 5)}}$
Now writing the condition of Wheatstone bridge we have,
$\dfrac{R}{{{S_1}}} = \dfrac{{{l'}}}{{100 - {l'}}}$
Putting the expression for ${S_1}$ we have,
$R = \dfrac{{12S}}{{(12 + S)}} \times \dfrac{{50}}{{50}}$
On simplifying the above expression we have,
$R = \dfrac{{12S}}{{12 + S}}$
Now we have two expressions for $R$ after equating them we have,
$\dfrac{2}{3}S = \dfrac{{12S}}{{(12 + S)}}$
On simplifying the above expression we have,
$12 + S = 18$
So we have, $S = 6\Omega $
So we have $R = \dfrac{2}{3} \times 6 = 4\Omega $
So, the values of $R$ and $S$ are $4\Omega $ and $6\Omega $ respectively.
Note: It is important to note the working principle of a meter bridge. A meter bridge is an instrument that works on the principle of a Wheatstone bridge. A meter bridge is used in finding the unknown resistance of a conductor as that of in a Wheatstone bridge. The null point of a Wheatstone is also known as the balance point of the Wheatstone bridge.
Recently Updated Pages
Uniform Acceleration - Definition, Equation, Examples, and FAQs

Difference Between Mass and Weight

JEE Main 2023 April 13 Shift 2 Question Paper with Answer Key

JEE Main 2023 April 11 Shift 2 Question Paper with Answer Key

JEE Main 2023 April 10 Shift 2 Question Paper with Answer Key

JEE Main 2023 (April 8th Shift 2) Physics Question Paper with Answer Key

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Displacement-Time Graph and Velocity-Time Graph for JEE

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Atomic Structure - Electrons, Protons, Neutrons and Atomic Models

Wheatstone Bridge for JEE Main Physics 2025

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

What is Hybridisation in Chemistry?

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation




