
Explain the formation of standing waves in case of an organ pipe open at one end and closed at the other end. Represent the first three modes of vibration diagrammatically and find their frequencies.
Answer
125.1k+ views
Hint: In this solution, we will use the relation of sound wave speed, distance, and velocity to determine the formation of standing waves and their frequencies. In a closed pipe, there is an antinode at the open end and a node at the closed end.
Complete step by step answer:
When sound waves are incident in a closed tube, they travel in the form of longitudinal waves. The waves that reach the closed bottom end of the tube, they are reflected back as shown in the diagrams below.
The closed wall of the tube acts as a rigid wall. This is because the air at the closed end is not free to vibrate. So, the reflected wave and the incident interfere with each other and form standing waves inside the organ. In this situation, a node is formed at the closed end. The air at the open end is free to vibrate with maximum amplitude and hence an antinode is formed there. This is the simplest mode of vibration of an air column in a closed well and is called the fundamental mode
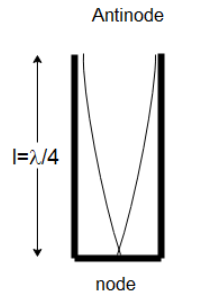
We know that the velocity of a sound wave is the product of its frequency and wavelength i.e.
$v = f.\lambda $
In the standing mode, the wavelength of the wave must be such that
$n\lambda = 4L$ where $n$ is the order of the harmonic. For the fundamental harmonic, $n = 1$. And $L$ is the length of the pipe.
Using the above two relations, we can write
$\dfrac{{nv}}{f} = 4L$
$ \Rightarrow f = \dfrac{v}{{4L}}\,{\text{(for n = 1)}}$
Now for a closed pipe, only antinodes can be formed at the open end of the pipe to form standing waves. So, only odd values of $n$ will be allowed to create standing waves as shown in the diagrams below.
So, the second overtone and the third overtone will have frequencies
${f_2} = \dfrac{{3v}}{{4l}}$
And
${f_3} = \dfrac{{5v}}{{4l}}$
The diagrams of the second and the third overtone respectively are shown below:
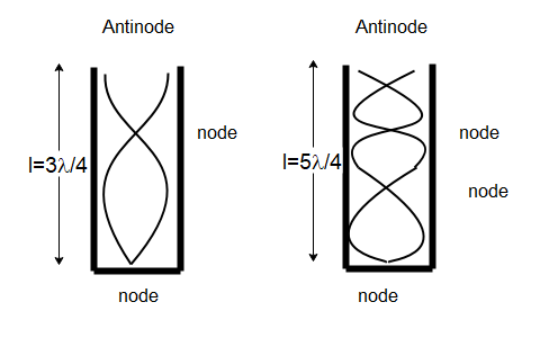
Note: While calculating the frequencies of the harmonic overtones, we must be careful that we select only odd values of \[n\]. This is because, for even values of $n$, an antinode will not be formed at the open end and standing waves won’t be able to form.
Complete step by step answer:
When sound waves are incident in a closed tube, they travel in the form of longitudinal waves. The waves that reach the closed bottom end of the tube, they are reflected back as shown in the diagrams below.
The closed wall of the tube acts as a rigid wall. This is because the air at the closed end is not free to vibrate. So, the reflected wave and the incident interfere with each other and form standing waves inside the organ. In this situation, a node is formed at the closed end. The air at the open end is free to vibrate with maximum amplitude and hence an antinode is formed there. This is the simplest mode of vibration of an air column in a closed well and is called the fundamental mode

We know that the velocity of a sound wave is the product of its frequency and wavelength i.e.
$v = f.\lambda $
In the standing mode, the wavelength of the wave must be such that
$n\lambda = 4L$ where $n$ is the order of the harmonic. For the fundamental harmonic, $n = 1$. And $L$ is the length of the pipe.
Using the above two relations, we can write
$\dfrac{{nv}}{f} = 4L$
$ \Rightarrow f = \dfrac{v}{{4L}}\,{\text{(for n = 1)}}$
Now for a closed pipe, only antinodes can be formed at the open end of the pipe to form standing waves. So, only odd values of $n$ will be allowed to create standing waves as shown in the diagrams below.
So, the second overtone and the third overtone will have frequencies
${f_2} = \dfrac{{3v}}{{4l}}$
And
${f_3} = \dfrac{{5v}}{{4l}}$
The diagrams of the second and the third overtone respectively are shown below:

Note: While calculating the frequencies of the harmonic overtones, we must be careful that we select only odd values of \[n\]. This is because, for even values of $n$, an antinode will not be formed at the open end and standing waves won’t be able to form.
Recently Updated Pages
The ratio of the diameters of two metallic rods of class 11 physics JEE_Main

What is the difference between Conduction and conv class 11 physics JEE_Main

Mark the correct statements about the friction between class 11 physics JEE_Main

Find the acceleration of the wedge towards the right class 11 physics JEE_Main

A standing wave is formed by the superposition of two class 11 physics JEE_Main

Derive an expression for work done by the gas in an class 11 physics JEE_Main

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility & More

Class 11 JEE Main Physics Mock Test 2025

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2023 January 24 Shift 2 Question Paper with Answer Keys & Solutions

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

NCERT Solutions for Class 11 Physics Chapter 1 Units and Measurements

NCERT Solutions for Class 11 Physics Chapter 9 Mechanical Properties of Fluids

Units and Measurements Class 11 Notes: CBSE Physics Chapter 1

NCERT Solutions for Class 11 Physics Chapter 2 Motion In A Straight Line

Important Questions for CBSE Class 11 Physics Chapter 1 - Units and Measurement




