NCERT Solutions for Class 11 Physics Chapter 5 Work Energy and Power - FREE PDF Download
FAQs on NCERT Solutions for Class 11 Physics Chapter 5 Work Energy and Power
1. What does Class 11 Physics Chapter 6 of NCERT book depict?
Class 11 Physics Chapter 6 of NCERT book deals with Work, Energy, and Power. With the help of this chapter, students will smoothly understand the relation between these three physical quantities. Scalar product and its distributive laws have also been taught in this chapter to form the foundation of further complex concepts.
Various other topics related to Work, Energy, and Power have been discussed in this chapter such as concepts of Kinetic energy, work done by variable force, work-energy theorem for a variable force, potential energy, conservation of mechanical energy, potential energy of a spring. Numerical problems have also been explained in a very detailed manner using theoretical and mathematical expressions.
Keeping in mind that energy comes in various forms such as heat, chemical energy, electrical energy, laws of conservation of energy is also explained in a detailed manner. Similarly, the principle of conservation of energy is also included in this chapter.
2. What are the topics/ subtopics covered under this chapter of Class 11 Physics?
There are a total of 12 topics and sub-topics in Chapter 6 of Class 11 Physics titled Work, Energy and Power. Check the topics and sub-topics given below.
Ex 6.1 - Introduction
Ex 6.2 - Notions of work and kinetic energy: The work-energy theorem
Ex 6.3 - Work
Ex 6.4 - Kinetic energy
Ex 6.5 - Work done by a variable force
Ex 6.6 - The work-energy theorem for a variable force
Ex 6.7 - The concept of potential energy
Ex 6.8 - The conservation of mechanical energy
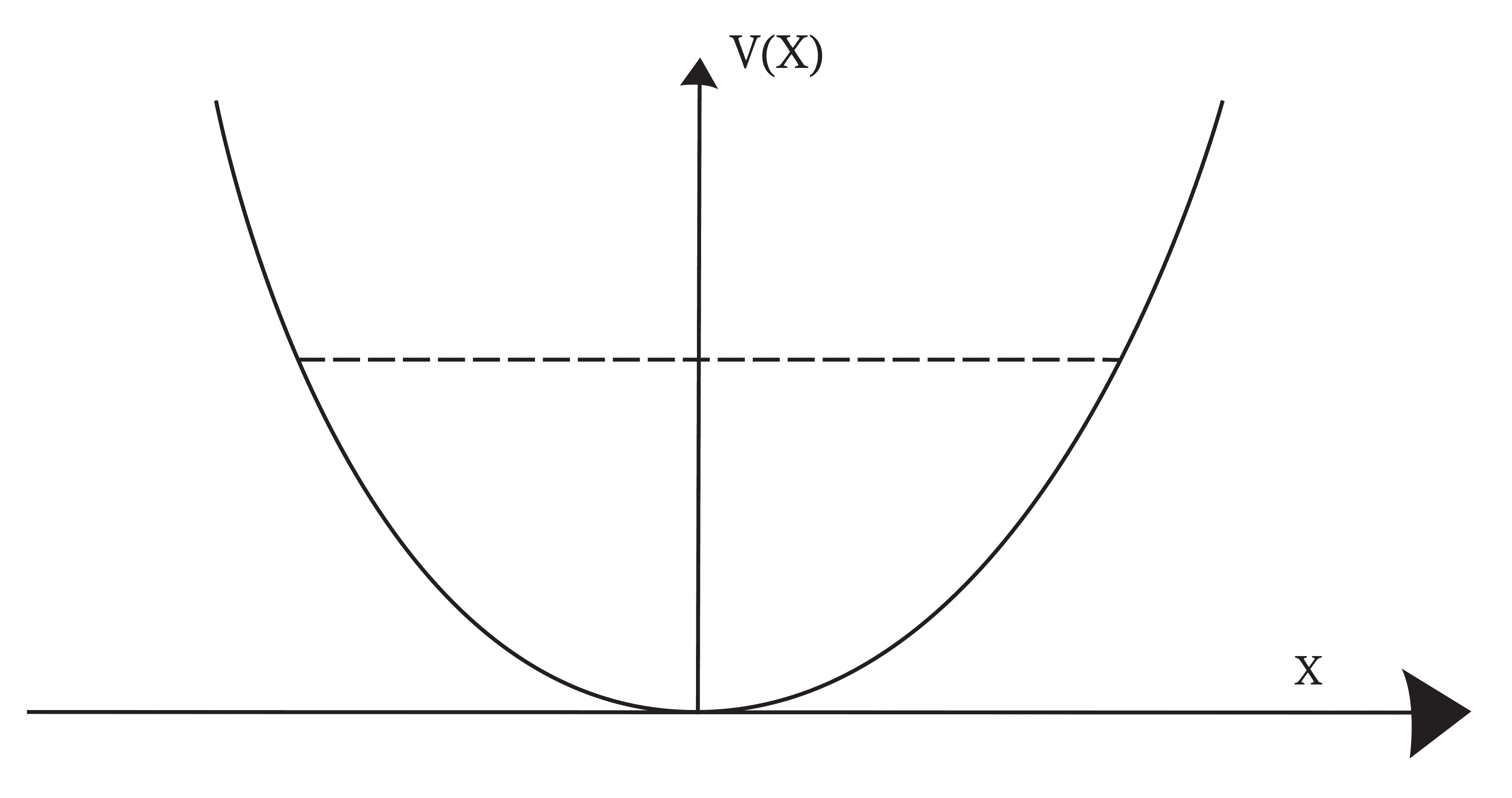
Ex 6.9 - The potential energy of a spring
Ex 6.10 - Various forms of energy: the law of conservation of energy
Ex 6.11 - Power
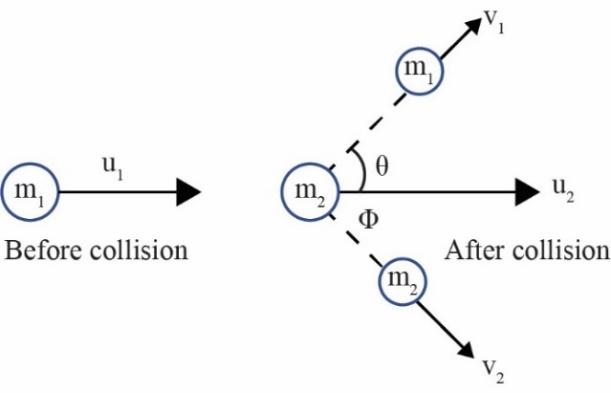
Ex 6.12 - Collisions
3. How many questions are contained in the exercises of Class 11 Physics Chapter 6 of NCERT book?
A total of 30 questions are there in the exercises of Class 11 Physics Chapter 6 of NCERT book. In Addition, answers to all these 30 questions are being provided in the NCERT Solutions by Vedantu. These answers are accumulated by the expert teachers of India's leading ed-tech site in an accurate and logical manner.
Being a part of Unit IV of NCERT textbook, Work, Energy and Power that holds 17 marks of weightage in the final Class 11 Physics exam along with Unit V and VI; Motion of System of Particles and Rigid Body and Gravitation respectively.
4. Why should one opt for the NCERT Solutions for Class 11 Physics Chapter 6 Work, Energy, and Power offered by Vedantu?
There are so many advantages of referring to the NCERT Solutions for Class 11 Physics Chapter 6 Work Energy and power offered by Vedantu. These solutions include all the important topics with in-depth and detailed explanations that aim to help the students of Class 11 understand the concepts in a better way. These solutions help the students to build a strong foundation about Work Energy and power.
The NCERT Solutions for Class 11 Physics Chapter 6 pdf available provided by Vedantu are explained in a logical order so that the students can easily understand all the concepts and hold a strong command over those. Going through the solutions provided here will also help the students to understand how they should approach and solve all the problems that they may face during the actual exam.
5. Where can I find numericals for Class 11 Physics Chapter 6 work, energy and power?
Students can solve all numericals given in Class 11 physics NCERT textbook. Students can find solutions to all numericals given in the textbook on Vedantu’s website. Students can understand the method of solving numericals to score high marks in Class 11 physics. All numericals are solved using simple strategies that can help students to understand the key points for solving numericals easily in the exam. All numericals are solved by expert physics teachers for easy understanding of the students.
6. What is the triple point of water?
The triple point of any substance refers to the combination of temperature and pressure at which a substance can exist in all three states: solid, liquid, and gas. The triple point of water is given a value of 273.16 K and a vapour pressure of 611.66 Pascal. At this point, water can exist in all three states: vapour, liquid, and ice by making small changes in pressure and temperature.
7. Which chapter is Work Energy and Power in Class 11 Physics?
Work, Energy, and Power is Chapter 6 of Class 11 Physics. It is an important Chapter as it covers a wide range of important concepts from the exam point of view. Students can get NCERT Solutions for Chapter 6 Class 11 on the Vedantu website and the app. Vedantu gives all Solutions in PDF format. Students can download the PDF file free of cost and study the notes prepared by expert teachers easily just by visiting the website.
8. How can I download NCERT Solutions Class 11 Physics Chapter 6 Work Energy And Power?
NCERT Solutions Class 11 Physics Chapter 6 Work Energy And Power are given on the internet. Students can download the NCERT Solutions Class 11 Physics Chapter 6 from the Vedantu website.
Visit the page-NCERT Solutions Class 11 Physics Chapter 6.
You download the given PDF file on your computer.
Save the file on your computer.
You can use the saved file anytime for offline study.
NCERT solutions can help students to prepare for their exams and score good marks.
9. What is the formula of Kinetic Energy?
The formula of Kinetic Energy $\dfrac{1}{2}MV^2$. You can find the complete explanation of the chapter on Vedantu. It will help make the chapter easier. Chapter 6 is an interesting chapter as long as you understand the concepts thoroughly.
10. What is the relationship between work, energy, and power explained in Class 11 Physics Chapter 5 NCERT Solutions?
Work, energy, and power are interrelated concepts in physics. Work is done when a force causes displacement. Energy is the capacity to do work, and power is the rate at which work is done. The relationship can be summarized as:
Work: W=F⋅d⋅cosθ
Energy: Energy is the ability to do work.
Power (P): P=W/t
11. From class 11 physics chapter 5 NCERT Solutions, how do Work Energy and Power together?
Work and power are related through the concept of time. Work is the energy transferred by a force acting over a distance, while power is the rate at which this work is done. In essence, power quantifies how quickly work is performed. If a certain amount of work is done in a shorter time, the power involved is greater.
12. What is the formula for work energy discussed in class 11 physics Work Energy and Power NCERT Solutions?
The work-energy theorem states that the work done on an object is equal to the change in its kinetic energy. The formula is:
$W = \Delta KE = \frac{1}{2}mv_f^2 - \frac{1}{2}mv_i^2$
Where,
W is the work done,
m is the mass,
$v_f$ is the final velocity, and
$v_i$ is the initial velocity.
13. What is the SI unit of energy mentioned in class 11 Physics ch 5 NCERT Solutions?
The SI unit of energy is the Joule (J). One joule is defined as the amount of work done when a force of one newton displaces an object by one meter in the direction of the force.
14. What is the full concept of work and energy discussed in class 11 Work Energy and Power NCERT Solutions?
Work involves transferring energy by applying force over a distance. Energy exists in various forms, such as kinetic energy (energy of motion) and potential energy (stored energy due to position). The work-energy theorem links work and energy, stating that the work done on an object results in a change in its kinetic energy. The law of conservation of energy states that energy can neither be created nor destroyed; it can only change forms.
15. What is the difference between work, power and energy mentioned in Work Energy and Power class 11 NCERT Solutions?
Work: Work is the energy transferred by a force over a distance. It is measured in joules (J).
Energy: Energy is the capacity to do work. It can exist in various forms, such as kinetic and potential energy, and is also measured in joules.
Power: Power is the rate at which work is done or energy is transferred. It is measured in watts (W), where one watt equals one joule per second.