Download FREE PDF for NCERT Solutions for Class 11 Maths Chapter 10 Conic Sections: Exercise 10.1
FAQs on NCERT Solutions Class 11 Maths Chapter 10 Conic Sections Exercise 10.1
1. What is the standard equation of a circle discussed in NCERT Solutions for Class 11 Maths Chapter 10?
The standard equation of a circle as explained in NCERT Solutions for Class 11 Maths Chapter 10 (Conic Sections) is (x – h)² + (y – k)² = r², where (h, k) is the centre and r is the radius.
2. How do you find the centre and radius from the general equation of a circle in Class 11 Maths Chapter 10?
For a circle in the form x² + y² + 2gx + 2fy + c = 0:
- The centre is (–g, –f).
- The radius is √(g² + f² – c).
3. Why is understanding conic sections important in Class 11 NCERT Solutions?
Conic sections are fundamental in geometry because they include circles, ellipses, parabolas, and hyperbolas. Mastery of these shapes is essential for solving real-world problems in mathematics, physics, and engineering as per the CBSE 2025–26 syllabus.
4. How do NCERT Solutions for Class 11 Maths Chapter 10 help in stepwise problem solving?
NCERT Solutions offer a stepwise approach for each question, clearly outlining the logic, formulas used, and plugging in values. This methodology helps students understand the reasoning behind each solution rather than just memorising answers.
5. What type of questions can be expected from Exercise 10.1 in NCERT Solutions for Class 11 Maths Chapter 10?
Exercise 10.1 primarily includes:
- Finding equations of circles given centre and radius
- Determining centre and radius from given equations
- Circumstances involving points, intercepts, and lines related to circles
6. What is the distance formula and how is it applied in problems on circles in Chapter 10?
The distance formula is d = √[(x₂ – x₁)² + (y₂ – y₁)²]. It is commonly used to calculate the radius when the centre and a point on the circle are given.
7. How can a student quickly check if a point lies inside, outside, or on a circle?
Calculate the distance between the point and the centre. If:
- Distance < radius, point is inside
- Distance = radius, point is on the circle
- Distance > radius, point is outside
8. What prior knowledge is essential for understanding Exercise 10.1 of Class 11 Maths Chapter 10?
Students should have a good grasp of coordinate geometry basics, including plotting points, understanding equations of lines, and using the distance formula to successfully solve problems in this exercise.
9. Can these NCERT Solutions help for competitive exams like JEE Main?
Yes, the stepwise approach and comprehensive explanations provided in the solutions cover important concepts that regularly appear in JEE Main and other entrance exams, as per the latest syllabus.
10. What are some common misconceptions students have when working with circles in Class 11?
Frequent misunderstandings include:
- Confusing the origin with the centre in the standard equation
- Misapplying the distance formula when the centre is not at (0,0)
- Incorrectly expanding or simplifying circle equations
11. How is the equation of a circle derived if it passes through two points and has its centre on a given line?
By plugging each point into the standard circle equation, you form two equations with variables for the centre. The condition that the centre lies on a specific line gives a third equation. Solving this system yields the centre and radius, allowing you to write the required equation as per NCERT methodology.
12. How can visualising conic sections assist in problem-solving for Class 11 students?
Visualisation helps identify the relative position of points, the orientation of the conic, and the geometric meaning of algebraic results, making it easier to check solutions and understand properties of circles, ellipses, etc.
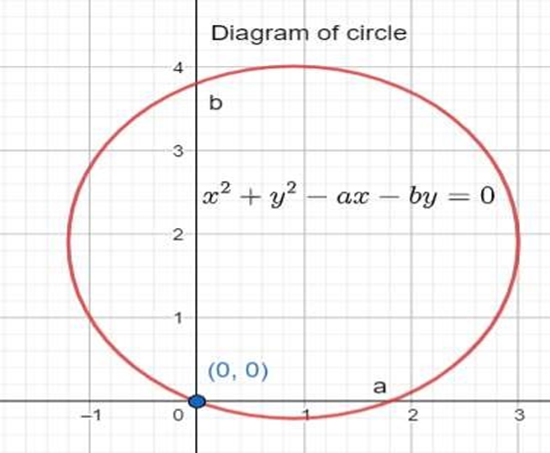
13. In case a circle has intercepts ‘a’ and ‘b’ on the axes and passes through the origin, what is its equation?
The required equation is x² + y² – a·x – b·y = 0 as derived through applying the intercept and origin conditions (as per the NCERT Solutions for Class 11 Maths Chapter 10).
14. What should a student do if their answer differs from the NCERT solution?
Re-examine the steps, check for calculation or conceptual errors (especially in sign, substitution into standard forms), and compare the logic. Refer back to the stepwise method in the NCERT Solutions for clarity.
15. How much weightage does Chapter 10 (Conic Sections) usually carry in Class 11 final exams according to current trends?
Conic Sections typically holds significant weightage in the CBSE Class 11 Maths board exam (about 8–10%) due to its foundational relevance and application across later chapters and higher studies.