
The value of electric potential at any point due to any electric dipole is:-
A. \[k\dfrac{{\overrightarrow P \times \overrightarrow r }}{{{r^2}}}\]
B. \[k\dfrac{{\overrightarrow P \times \overrightarrow r }}{{{r^3}}}\]
C. \[k\dfrac{{\overrightarrow P \cdot \overrightarrow r }}{{{r^2}}}\]
D. \[k\dfrac{{\overrightarrow P \cdot \overrightarrow r }}{{{r^3}}}\]
Answer
173.7k+ views
Hint:The electric dipole is a system of two charges that are separated by a finite distance. The dipole has a property called dipole moment which is equal to the product of the charges and the distance of separation between them. To find the value of electric potential at any point due to any electric dipole we use electric potential due to both charges.
Formula used: >
The potential due to this dipole is given as
\[V = \dfrac{1}{{4\pi {\varepsilon _0}}}\dfrac{{p\cos \theta }}{{{r^2}}}\]
Where p is dipole moment, r is distance from dipole, k is coulomb’s constant,\[k = \dfrac{1}{{4\pi {\varepsilon _0}}} = 9 \times {10^9}N{m^2}\] and \[{\varepsilon _0}\] is permittivity.
Dipole moment is given as,
\[p = q \times 2l\]
Where q is the charge and 2l is the distance of two charges.
Complete step by step solution:
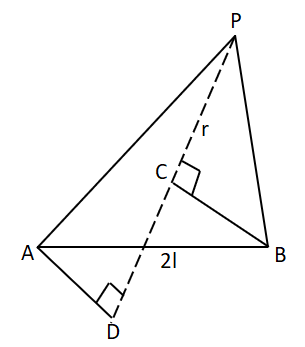
Image: A point P is at some distance r from the dipole.
In figure AB the dipole of distance between them is 2l. Point P is at a distance r from the midpoint of AB.
BP=CP=OP-OC= \[r - l\cos \theta \]
AP=DP=OP-OD=\[r + l\cos \theta \]
Electric potential due to -q,
\[{V_1} = \dfrac{1}{{4\pi {\varepsilon _0}}}\dfrac{{( - q)}}{{AP}}\]
Electric potential due to +q,
\[{V_2} = \dfrac{1}{{4\pi {\varepsilon _0}}}\dfrac{{( + q)}}{{BP}}\]
Now electric potential at P can be:
\[V = {V_1} + {V_2}\]
\[\Rightarrow V = qk\left[ { - \dfrac{1}{{r + l\cos \theta }} + \dfrac{1}{{r - l\cos \theta }}} \right]\] where \[k = \dfrac{1}{{4\pi {\varepsilon _0}}}\]
\[\Rightarrow V = \dfrac{{qk2l\cos \theta }}{{{r^2} - {l^2}{{\cos }^2}\theta }}\]
As distance between the dipoles are less, so we can write;
\[{l^2}{\cos ^2}\theta \approx 0\]
Also we know that \[p = q \times 2l\]
By using these values in above, we get
\[V = k\dfrac{{pr\cos \theta }}{{{r^3}}}\]
\[\therefore V = k\dfrac{{\overrightarrow p \cdot \overrightarrow r }}{{{r^3}}}\]
Therefore the value of electric potential at any point due to any electric dipole is \[V = k\dfrac{{\overrightarrow p \cdot \overrightarrow r }}{{{r^3}}}\]
Hence option D is the correct answer.
Note: As we know electric dipoles consist of two charges equal in magnitude (q) but opposite in nature one is a positive charge and other is a negative charge. These two charges are separated by a distance. Electric potential obeys superposition principle due to electric dipoles as a whole can be sum of potential due to both the charges positive and negative.
Formula used: >
The potential due to this dipole is given as
\[V = \dfrac{1}{{4\pi {\varepsilon _0}}}\dfrac{{p\cos \theta }}{{{r^2}}}\]
Where p is dipole moment, r is distance from dipole, k is coulomb’s constant,\[k = \dfrac{1}{{4\pi {\varepsilon _0}}} = 9 \times {10^9}N{m^2}\] and \[{\varepsilon _0}\] is permittivity.
Dipole moment is given as,
\[p = q \times 2l\]
Where q is the charge and 2l is the distance of two charges.
Complete step by step solution:

Image: A point P is at some distance r from the dipole.
In figure AB the dipole of distance between them is 2l. Point P is at a distance r from the midpoint of AB.
BP=CP=OP-OC= \[r - l\cos \theta \]
AP=DP=OP-OD=\[r + l\cos \theta \]
Electric potential due to -q,
\[{V_1} = \dfrac{1}{{4\pi {\varepsilon _0}}}\dfrac{{( - q)}}{{AP}}\]
Electric potential due to +q,
\[{V_2} = \dfrac{1}{{4\pi {\varepsilon _0}}}\dfrac{{( + q)}}{{BP}}\]
Now electric potential at P can be:
\[V = {V_1} + {V_2}\]
\[\Rightarrow V = qk\left[ { - \dfrac{1}{{r + l\cos \theta }} + \dfrac{1}{{r - l\cos \theta }}} \right]\] where \[k = \dfrac{1}{{4\pi {\varepsilon _0}}}\]
\[\Rightarrow V = \dfrac{{qk2l\cos \theta }}{{{r^2} - {l^2}{{\cos }^2}\theta }}\]
As distance between the dipoles are less, so we can write;
\[{l^2}{\cos ^2}\theta \approx 0\]
Also we know that \[p = q \times 2l\]
By using these values in above, we get
\[V = k\dfrac{{pr\cos \theta }}{{{r^3}}}\]
\[\therefore V = k\dfrac{{\overrightarrow p \cdot \overrightarrow r }}{{{r^3}}}\]
Therefore the value of electric potential at any point due to any electric dipole is \[V = k\dfrac{{\overrightarrow p \cdot \overrightarrow r }}{{{r^3}}}\]
Hence option D is the correct answer.
Note: As we know electric dipoles consist of two charges equal in magnitude (q) but opposite in nature one is a positive charge and other is a negative charge. These two charges are separated by a distance. Electric potential obeys superposition principle due to electric dipoles as a whole can be sum of potential due to both the charges positive and negative.
Recently Updated Pages
JEE Main 2025-26 Atoms and Nuclei Mock Test: Free Practice Online

JEE Main 2025-26: Dual Nature of Matter and Radiation Mock Test

JEE Main 2025-26 Electronic Devices Mock Test – Free Practice

JEE Main Mock Test 2025-26: Experimental Skills Chapter Online Practice

JEE Main 2025-26 Current Electricity Mock Test: Free Practice Online

JEE Main 2025-26 Rotational Motion Mock Test – Free Practice Online

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

Displacement-Time Graph and Velocity-Time Graph for JEE

Uniform Acceleration

Electric field due to uniformly charged sphere class 12 physics JEE_Main

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Instantaneous Velocity - Formula based Examples for JEE

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

Essential Derivations for CBSE Class 12 Physics: Stepwise & PDF Solutions

Electron Gain Enthalpy and Electron Affinity for JEE

What is Hybridisation in Chemistry?




