
The potential difference across $8\Omega $ resistance is $48V$ as shown in the figure. The value of potential difference across $X$ and $Y$ points will be
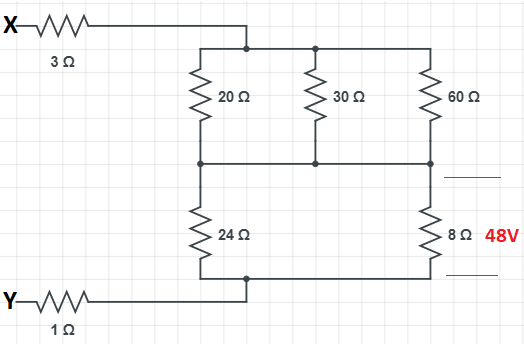
(A) $128V$
(B) $160V$
(C) $80V$
(D) $62V$
Answer
146.7k+ views
Hint: In the given circuit the resistors are connected in both parallel and series connection from the point X to the point Y. In this connection a particular parallel connection has a potential difference of $48V$. We have to find the net potential difference across X and Y. Find the current through the circuit using the given potential difference across the parallel connection and also find the net resistance across X and Y. Now we have the net current and the net resistance across X and Y. Apply ohm’s law to find the net potential.
Complete step by step answer:
Given,
In the given circuit, the below mentioned resistors are connected in parallel
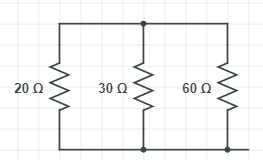
Here,
${R_{P1}} = 20\Omega $
${R_{P2}} = 30\Omega $
${R_{P3}} = 60\Omega $
The total resistance of the above circuit is ${R_p}$
The total resistance of the resistors connected parallel is given by
\[ \Rightarrow \dfrac{1}{{{R_P}}} = \dfrac{1}{{{R_1}}} + \dfrac{1}{{{R_2}}} + \dfrac{1}{{{R_3}}}\]
$ \Rightarrow \dfrac{1}{{{R_P}}} = \dfrac{1}{{20}} + \dfrac{1}{{30}} + \dfrac{1}{{60}}$
$ \Rightarrow \dfrac{1}{{{R_P}}} = \dfrac{3}{{60}} + \dfrac{2}{{60}} + \dfrac{1}{{60}}$
$ \Rightarrow \dfrac{1}{{{R_P}}} = \dfrac{6}{{60}}$
$ \Rightarrow \dfrac{1}{{{R_{P1}}}} = \dfrac{1}{{10}}$
$ \Rightarrow {R_{P1}} = 10$
In the given circuit, the below mentioned resistors are also connected in parallel.
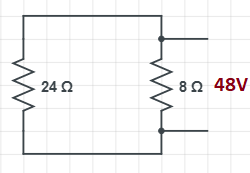
Here,
${R_{P1}} = 24\Omega $
${R_{P2}} = 8\Omega $
$ \Rightarrow \dfrac{1}{{{R_P}}} = \dfrac{1}{{{R_1}}} + \dfrac{1}{{{R_2}}}$
$ \Rightarrow \dfrac{1}{{{R_P}}} = \dfrac{1}{{24}} + \dfrac{1}{8}$
$ \Rightarrow \dfrac{1}{{{R_P}}} = \dfrac{1}{{24}} + \dfrac{3}{{24}}$
$ \Rightarrow \dfrac{1}{{{R_P}}} = \dfrac{4}{{24}}$
$ \Rightarrow \dfrac{1}{{{R_{P2}}}} = \dfrac{1}{6}$
$ \Rightarrow {R_{P2}} = 6$
And also,
Given that the potential difference across $8\Omega $ is $48V$
Since ${R_{P2}} = 8\Omega $ and ${R_{P1}} = 24\Omega $ are connected in parallel the potential difference $48V$ is applied to both the resistors, we found the net resistance of this resistor circuit
$ \Rightarrow {R_{P2}} = 6$
Given, $V = 48V$
According to ohm’s law we know that
$ \Rightarrow V = IR$
$ \Rightarrow I = \dfrac{V}{R}$
Substitute the given values
$ \Rightarrow I = \dfrac{{48}}{6}$
$ \Rightarrow I = 8A$
The current through the circuit is $I = 8A$
And the circuit becomes
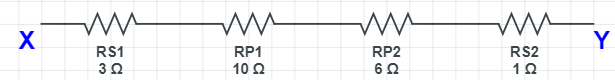
The resistors are in series connection
The total resistance of the resistors connected parallel is given by
\[ \Rightarrow {R_S} = {R_1} + {R_2} + {R_3} + ... + {R_n}\]
\[ \Rightarrow {R_S} = {R_{S1}} + {R_{P1}} + {R_{P2}} + {R_{S2}}\]
\[ \Rightarrow {R_S} = 3 + 10 + 6 + 1\]
\[ \Rightarrow {R_S} = 20\]
The total resistance across X and Y is \[{R_S} = 20\]
The current through the circuit is $I = 8A$
The potential difference across X and Y is given by the ohm’s law
$ \Rightarrow V = IR$
$ \Rightarrow V = 8 \times 20$
$ \Rightarrow V = 160V$
Hence the correct answer is option (B), $160V$.
Note: Ohm’s law states that at constant temperature the potential difference across the conductor is proportional to the steady current flowing through the conductor.
$ \Rightarrow V \propto I$
$ \Rightarrow V = IR$
$V$ is the potential difference
$I$ is the current
$R$ is the resistance
Complete step by step answer:
Given,
In the given circuit, the below mentioned resistors are connected in parallel

Here,
${R_{P1}} = 20\Omega $
${R_{P2}} = 30\Omega $
${R_{P3}} = 60\Omega $
The total resistance of the above circuit is ${R_p}$
The total resistance of the resistors connected parallel is given by
\[ \Rightarrow \dfrac{1}{{{R_P}}} = \dfrac{1}{{{R_1}}} + \dfrac{1}{{{R_2}}} + \dfrac{1}{{{R_3}}}\]
$ \Rightarrow \dfrac{1}{{{R_P}}} = \dfrac{1}{{20}} + \dfrac{1}{{30}} + \dfrac{1}{{60}}$
$ \Rightarrow \dfrac{1}{{{R_P}}} = \dfrac{3}{{60}} + \dfrac{2}{{60}} + \dfrac{1}{{60}}$
$ \Rightarrow \dfrac{1}{{{R_P}}} = \dfrac{6}{{60}}$
$ \Rightarrow \dfrac{1}{{{R_{P1}}}} = \dfrac{1}{{10}}$
$ \Rightarrow {R_{P1}} = 10$
In the given circuit, the below mentioned resistors are also connected in parallel.

Here,
${R_{P1}} = 24\Omega $
${R_{P2}} = 8\Omega $
$ \Rightarrow \dfrac{1}{{{R_P}}} = \dfrac{1}{{{R_1}}} + \dfrac{1}{{{R_2}}}$
$ \Rightarrow \dfrac{1}{{{R_P}}} = \dfrac{1}{{24}} + \dfrac{1}{8}$
$ \Rightarrow \dfrac{1}{{{R_P}}} = \dfrac{1}{{24}} + \dfrac{3}{{24}}$
$ \Rightarrow \dfrac{1}{{{R_P}}} = \dfrac{4}{{24}}$
$ \Rightarrow \dfrac{1}{{{R_{P2}}}} = \dfrac{1}{6}$
$ \Rightarrow {R_{P2}} = 6$
And also,
Given that the potential difference across $8\Omega $ is $48V$
Since ${R_{P2}} = 8\Omega $ and ${R_{P1}} = 24\Omega $ are connected in parallel the potential difference $48V$ is applied to both the resistors, we found the net resistance of this resistor circuit
$ \Rightarrow {R_{P2}} = 6$
Given, $V = 48V$
According to ohm’s law we know that
$ \Rightarrow V = IR$
$ \Rightarrow I = \dfrac{V}{R}$
Substitute the given values
$ \Rightarrow I = \dfrac{{48}}{6}$
$ \Rightarrow I = 8A$
The current through the circuit is $I = 8A$
And the circuit becomes

The resistors are in series connection
The total resistance of the resistors connected parallel is given by
\[ \Rightarrow {R_S} = {R_1} + {R_2} + {R_3} + ... + {R_n}\]
\[ \Rightarrow {R_S} = {R_{S1}} + {R_{P1}} + {R_{P2}} + {R_{S2}}\]
\[ \Rightarrow {R_S} = 3 + 10 + 6 + 1\]
\[ \Rightarrow {R_S} = 20\]
The total resistance across X and Y is \[{R_S} = 20\]
The current through the circuit is $I = 8A$
The potential difference across X and Y is given by the ohm’s law
$ \Rightarrow V = IR$
$ \Rightarrow V = 8 \times 20$
$ \Rightarrow V = 160V$
Hence the correct answer is option (B), $160V$.
Note: Ohm’s law states that at constant temperature the potential difference across the conductor is proportional to the steady current flowing through the conductor.
$ \Rightarrow V \propto I$
$ \Rightarrow V = IR$
$V$ is the potential difference
$I$ is the current
$R$ is the resistance
Recently Updated Pages
How to find Oxidation Number - Important Concepts for JEE

How Electromagnetic Waves are Formed - Important Concepts for JEE

Electrical Resistance - Important Concepts and Tips for JEE

Average Atomic Mass - Important Concepts and Tips for JEE

Chemical Equation - Important Concepts and Tips for JEE

Concept of CP and CV of Gas - Important Concepts and Tips for JEE

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2025: Derivation of Equation of Trajectory in Physics

JEE Main Participating Colleges 2024 - A Complete List of Top Colleges

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

Degree of Dissociation and Its Formula With Solved Example for JEE

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Ideal and Non-Ideal Solutions Raoult's Law - JEE




