
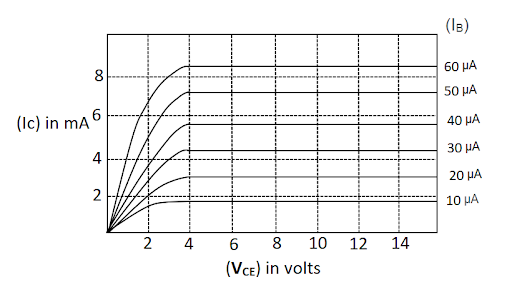
The output characteristics of a transistor is shown in the figure. When \[{V_{CE}}\] is 10V and \[{I_C}\]= 4.0 mA, then the value of \[{\beta _{ac}}\] is __________.
Answer
180k+ views
Hint:A transistor is defined as a semiconductor device used to conduct an electric current or electric voltage. For a transistor, the three terminals are base, collector and emitter. When we combine the expressions for \[\alpha \] and \[\beta \] the mathematical relationship between these parameters then we get the current gain of the transistor. By using the relation, we can find the value for \[\beta \].
Formula used:
The mathematical relationship between these parameters can be given as:
\[\alpha = \dfrac{{{I_C}}}{{{I_E}}}\] and \[\beta = \dfrac{{{I_C}}}{{{I_B}}}\]
\[\Rightarrow {I_C} = \alpha {I_E} = \beta {I_B}\]
As \[\alpha = \dfrac{\beta }{{\beta + 1}}\]
\[\beta = \dfrac{\alpha }{{1 - \alpha }}\]
\[{I_E} = {I_C} + {I_B}\]
Where \[{I_C}\] is the current in the collector terminal, \[{I_B}\] is the current in the base terminal and \[{I_E}\] is the current in the emitter terminal.
Complete step by step solution:
A transistor can also use a small signal which is applied between one pair of its terminals to control a large signal at the other pair of terminals. This property is known as gain. A stronger output signal can produce a voltage as well as a current which is dependable on a weaker input signal.
\[\beta = \dfrac{{\Delta {I_c}}}{{\Delta {I_B}}}\]
Substituting the values
\[\Delta {I_B} = 30 - 20{I_C} = 4\,mA\]
\[\Rightarrow \Delta {I_C} = 4.5 - 3 = 1.5\,mA\]
Now \[\beta = \dfrac{{1.5 \times {{10}^{ - 3}}}}{{10 \times {{10}^{ - 6}}}}\]
\[\therefore \beta = 150\]
Therefore, the value of \[{\beta _{ac}}\] is 150.
Note: In physics, for any transistor the graph shows the relationships between the current(I) and the voltage (V) for any configuration is known as transistor characteristics. The current transfer properties curve can define the variation of output current to the input current by keeping the output voltage constant. There are three transistor circuit configurations. These are Common Emitter Transistor, Common Base Transistor and Common Collector Transistor.
Formula used:
The mathematical relationship between these parameters can be given as:
\[\alpha = \dfrac{{{I_C}}}{{{I_E}}}\] and \[\beta = \dfrac{{{I_C}}}{{{I_B}}}\]
\[\Rightarrow {I_C} = \alpha {I_E} = \beta {I_B}\]
As \[\alpha = \dfrac{\beta }{{\beta + 1}}\]
\[\beta = \dfrac{\alpha }{{1 - \alpha }}\]
\[{I_E} = {I_C} + {I_B}\]
Where \[{I_C}\] is the current in the collector terminal, \[{I_B}\] is the current in the base terminal and \[{I_E}\] is the current in the emitter terminal.
Complete step by step solution:
A transistor can also use a small signal which is applied between one pair of its terminals to control a large signal at the other pair of terminals. This property is known as gain. A stronger output signal can produce a voltage as well as a current which is dependable on a weaker input signal.
\[\beta = \dfrac{{\Delta {I_c}}}{{\Delta {I_B}}}\]
Substituting the values
\[\Delta {I_B} = 30 - 20{I_C} = 4\,mA\]
\[\Rightarrow \Delta {I_C} = 4.5 - 3 = 1.5\,mA\]
Now \[\beta = \dfrac{{1.5 \times {{10}^{ - 3}}}}{{10 \times {{10}^{ - 6}}}}\]
\[\therefore \beta = 150\]
Therefore, the value of \[{\beta _{ac}}\] is 150.
Note: In physics, for any transistor the graph shows the relationships between the current(I) and the voltage (V) for any configuration is known as transistor characteristics. The current transfer properties curve can define the variation of output current to the input current by keeping the output voltage constant. There are three transistor circuit configurations. These are Common Emitter Transistor, Common Base Transistor and Common Collector Transistor.
Recently Updated Pages
Degree of Dissociation Important Concepts and Tips for JEE

JEE Main Chemistry Question Paper PDF Download with Answer Key

JEE Electricity and Magnetism Important Concepts and Tips for Exam Preparation

JEE Energetics Important Concepts and Tips for Exam Preparation

JEE Isolation, Preparation and Properties of Non-metals Important Concepts and Tips for Exam Preparation

States of Matter Chapter For JEE Main Chemistry

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Displacement-Time Graph and Velocity-Time Graph for JEE

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Atomic Structure - Electrons, Protons, Neutrons and Atomic Models

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

What is Hybridisation in Chemistry?

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

Wheatstone Bridge for JEE Main Physics 2025

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation




