
Radius of curvature of spherical mirror is:
A) Half of focal length
B) Double of focal length
C) Equal to focal length.
D) No relation.
Answer
202.2k+ views
Hint: In this as we know that the spherical lens is a part of a spherical mirror. The relation between focal length of a spherical lens and the radius of a spherical lens is calculated by understanding the sign convention.
Complete step by step answer:
As we know that the center of a spherical mirror or the lens is known as the center of curvature of that mirror or lens. The radius of a spherical mirror or lens is defined as the distance between the centre of curvature to the circumference of the curvature.
The figure below represents the sketch diagram of a curved surface (mirror or lens).
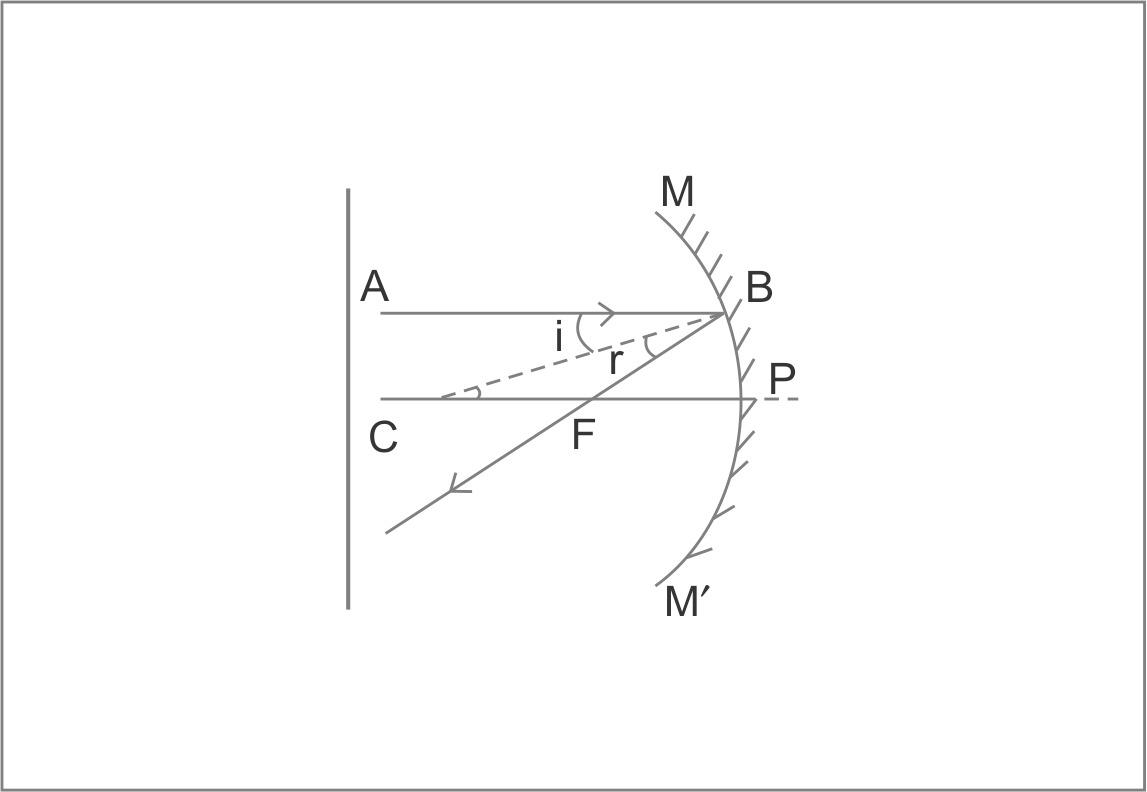
As we can see in the above figure $CP = CB$. These both distances are the radius of the mirror.
From the geometry of the Figure it is clear that,
$ \Rightarrow BF = PF$
Further we can conclude that,
$ \Rightarrow FC = FP$
Also,
$ \Rightarrow FP = PF$
From the diagram we can write that,
$ \Rightarrow PC = PF + FC$
Now we substitute $PF$ for $FC$ in above equation.
$PC = PF + PF$
After simplification we get,
$ \Rightarrow R = 2PF$
Now substitute $f$ for$PF$ in above equation to get
$\therefore R = 2f$
Here, $f$ is the focal length of the spherical lens.
It is clear from the above expression that the radius of curvature of a spherical lens is twice the focal length of the lens.
Therefore, the correct option is (B).
Note: In this question the diagram of the spherical lens should be made clear and the basic knowledge of geometry is applied. The main concept in this question is substitution of various data (distance) from the diagram. If this substitution goes wrong the answer will be wrong.
Complete step by step answer:
As we know that the center of a spherical mirror or the lens is known as the center of curvature of that mirror or lens. The radius of a spherical mirror or lens is defined as the distance between the centre of curvature to the circumference of the curvature.
The figure below represents the sketch diagram of a curved surface (mirror or lens).

As we can see in the above figure $CP = CB$. These both distances are the radius of the mirror.
From the geometry of the Figure it is clear that,
$ \Rightarrow BF = PF$
Further we can conclude that,
$ \Rightarrow FC = FP$
Also,
$ \Rightarrow FP = PF$
From the diagram we can write that,
$ \Rightarrow PC = PF + FC$
Now we substitute $PF$ for $FC$ in above equation.
$PC = PF + PF$
After simplification we get,
$ \Rightarrow R = 2PF$
Now substitute $f$ for$PF$ in above equation to get
$\therefore R = 2f$
Here, $f$ is the focal length of the spherical lens.
It is clear from the above expression that the radius of curvature of a spherical lens is twice the focal length of the lens.
Therefore, the correct option is (B).
Note: In this question the diagram of the spherical lens should be made clear and the basic knowledge of geometry is applied. The main concept in this question is substitution of various data (distance) from the diagram. If this substitution goes wrong the answer will be wrong.
Recently Updated Pages
Chemical Equation - Important Concepts and Tips for JEE

JEE Main 2022 (July 29th Shift 1) Chemistry Question Paper with Answer Key

Conduction, Transfer of Energy Important Concepts and Tips for JEE

JEE Analytical Method of Vector Addition Important Concepts and Tips

Atomic Size - Important Concepts and Tips for JEE

JEE Main 2022 (June 29th Shift 1) Maths Question Paper with Answer Key

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

Equation of Trajectory in Projectile Motion: Derivation & Proof

Atomic Structure: Definition, Models, and Examples

How to Convert a Galvanometer into an Ammeter or Voltmeter

Hybridisation in Chemistry – Concept, Types & Applications

Angle of Deviation in a Prism – Formula, Diagram & Applications

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Collision: Meaning, Types & Examples in Physics

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

Average and RMS Value in Physics: Formula, Comparison & Application

Elastic Collisions in One Dimension: Concepts, Derivation, and Examples

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry




