
What is the physical meaning of cross-product?
Answer
202.5k+ views
Hint: For a resultant vector, the cross product is known as the vector product of the two vectors and can be written as A x B. This resultant vector illustrates a cross-product between two vectors on a plane surface.
Complete step by step solution:
We know that A vector is a quantity with magnitude and direction used to locate one location in space in relation to another. It can be visualized as an arrow or a line segment with the direction in a geometrical representation.
Mathematically, the cross product of two non-zero vectors \[\overrightarrow a \] and \[\overrightarrow b \] , is
\[\overrightarrow a \times \overrightarrow b = |\overrightarrow a ||\overrightarrow b |sin\,\theta \widehat {\,n}\]
Where \[\theta \] is the angle between \[\overrightarrow a \] and \[\overrightarrow b \] , \[0 \leqslant \theta \leqslant \pi \] . Also, \[\widehat n\] is a unit vector perpendicular to both \[\overrightarrow a \] and \[\overrightarrow b \] such that \[\overrightarrow a ,\overrightarrow b \] and \[\widehat {\,n}\] form a right-handed system.
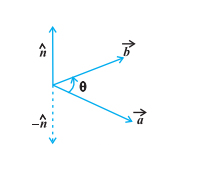
Image: cross product of two vectors
Hence, the physical meaning of cross product is a vector that is perpendicular to the two vectors is the cross product of any two vectors. It has magnitude as well as direction. The resultant vector has the same magnitude as the parallelogram, whose side lengths are the same as the magnitudes of the two provided vectors.
Note: Students should remember the right-hand thumb rule in which you stretch your right hand so that the index finger of the right hand is in the direction of the first vector and the middle finger is in the direction of the second vector. Then, the thumb of the right hand indicates the direction vector.
Complete step by step solution:
We know that A vector is a quantity with magnitude and direction used to locate one location in space in relation to another. It can be visualized as an arrow or a line segment with the direction in a geometrical representation.
Mathematically, the cross product of two non-zero vectors \[\overrightarrow a \] and \[\overrightarrow b \] , is
\[\overrightarrow a \times \overrightarrow b = |\overrightarrow a ||\overrightarrow b |sin\,\theta \widehat {\,n}\]
Where \[\theta \] is the angle between \[\overrightarrow a \] and \[\overrightarrow b \] , \[0 \leqslant \theta \leqslant \pi \] . Also, \[\widehat n\] is a unit vector perpendicular to both \[\overrightarrow a \] and \[\overrightarrow b \] such that \[\overrightarrow a ,\overrightarrow b \] and \[\widehat {\,n}\] form a right-handed system.

Image: cross product of two vectors
Hence, the physical meaning of cross product is a vector that is perpendicular to the two vectors is the cross product of any two vectors. It has magnitude as well as direction. The resultant vector has the same magnitude as the parallelogram, whose side lengths are the same as the magnitudes of the two provided vectors.
Note: Students should remember the right-hand thumb rule in which you stretch your right hand so that the index finger of the right hand is in the direction of the first vector and the middle finger is in the direction of the second vector. Then, the thumb of the right hand indicates the direction vector.
Recently Updated Pages
Chemical Equation - Important Concepts and Tips for JEE

JEE Main 2022 (July 29th Shift 1) Chemistry Question Paper with Answer Key

Conduction, Transfer of Energy Important Concepts and Tips for JEE

JEE Analytical Method of Vector Addition Important Concepts and Tips

Atomic Size - Important Concepts and Tips for JEE

JEE Main 2022 (June 29th Shift 1) Maths Question Paper with Answer Key

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

Atomic Structure: Definition, Models, and Examples

Angle of Deviation in a Prism – Formula, Diagram & Applications

Collision: Meaning, Types & Examples in Physics

Hybridisation in Chemistry – Concept, Types & Applications

Equation of Trajectory in Projectile Motion: Derivation & Proof

Other Pages
Units And Measurements Class 11 Physics Chapter 1 CBSE Notes - 2025-26

NCERT Solutions for Class 11 Physics Chapter 7 Gravitation 2025-26

JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

JEE Advanced 2025: Dates, Registration, Syllabus, Eligibility Criteria and More

Laws of Motion Class 11 Physics Chapter 4 CBSE Notes - 2025-26

NCERT Solutions For Class 11 Physics Chapter 2 Motion In A Straight Line - 2025-26




