
Four particles of masses m, 2m, 3m and 4m are placed at the corners of a square of side of length ‘a’. The gravitational force on a particle of mass m placed at the centre of the square is
A) \[4\sqrt 2 \dfrac{{G{m^2}}}{{{a^2}}}\]
B) $3\sqrt 2 \dfrac{{G{m^2}}}{{{a^2}}}$
C) $2\sqrt 2 \dfrac{{G{m^2}}}{{{a^2}}}$
D) $\sqrt 2 \dfrac{{G{m^2}}}{{{a^2}}}$
Answer
200.7k+ views
Hint: Recall Newton’s law of universal gravitation. According to this law, every object attracts other objects in the universe. The force of attraction points in a straight line between the centre of both the objects. It is directly proportional to the mass of the objects and inversely proportional to the square of distance between them.
Complete step by step solution:
The formula for gravitational force is given by
$F = \dfrac{{G{m_1}{m_2}}}{{{r^2}}}$
Where G is the gravitational force
${m_1}$ is the mass of the first object
${m_2}$ is the mass of the second object
And ‘r’ is the distance between them
Given that the length of each side of the square is = a
The distance of each corner from the centre will be $ = \dfrac{a}{{\sqrt 2 }}$
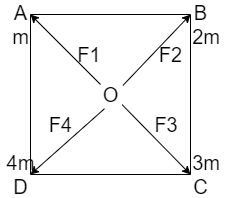
The gravitational force on point O due to point A is given by
\[\Rightarrow {F_1} = \dfrac{{Gmm}}{{{{(\dfrac{a}{{\sqrt 2 }})}^2}}} = \dfrac{{2G{m^2}}}{{{a^2}}}\]
Similarly, the gravitational force on point O due to point B is written as
$\Rightarrow {F_2} = \dfrac{{G(2m)(m)}}{{{{(\dfrac{a}{{\sqrt 2 }})}^2}}} = \dfrac{{4G{m^2}}}{{{a^2}}}$
The gravitational force on point O due to point C will be
$\Rightarrow {F_3} = \dfrac{{G(3m)(m)}}{{{{(\dfrac{a}{{\sqrt 2 }})}^2}}} = \dfrac{{6G{m^2}}}{{{a^2}}}$
The gravitational force on point O due to point D will be
$\Rightarrow {F_4} = \dfrac{{G(4m)(m)}}{{{{(\dfrac{a}{{\sqrt 2 }})}^2}}} = \dfrac{{8G{m^2}}}{{{a^2}}}$
The net force of ${F_1}$and ${F_3}$on point O is given by
$\Rightarrow {F_{13}} = {F_3} - {F_1}$
$\Rightarrow {F_{13}} = \dfrac{{6G{m^2}}}{{{a^2}}} - \dfrac{{2G{m^2}}}{{{a^2}}} = \dfrac{{4G{m^2}}}{{{a^2}}}$
Similarly, the force of ${F_2}$and${F_4}$on point O is given by
$\Rightarrow {F_{24}} = {F_4} - {F_2}$
$\Rightarrow {F_{24}} = \dfrac{{8G{m^2}}}{{{a^2}}} - \dfrac{{4G{m^2}}}{{{a^2}}} = \dfrac{{4G{m^2}}}{{{a^2}}}$
The magnitude of net force on point O is given by
$\Rightarrow F = \sqrt {{{({F_{13}})}^2} + {{({F_{24}})}^2}} $
$\Rightarrow F = \sqrt {{{(\dfrac{{4G{m^2}}}{{{a^2}}})}^2} + {{(\dfrac{{4G{m^2}}}{{{a^2}}})}^2}} $
$\Rightarrow F = \sqrt {2.\dfrac{{4{G^2}{m^4}}}{{{a^4}}}} $
\[\Rightarrow F = 2\sqrt 2 \dfrac{{G{m^2}}}{{{a^2}}}\]
Option C is the right answer.
Note: It is important to note that since the force of gravitation is directly proportional to the mass of the object, so an object with more mass will have a large force of gravitation. But it varies inversely with the square of distance, so if the distance is doubled the force of gravitation will decrease by 4 times and so on.
Complete step by step solution:
The formula for gravitational force is given by
$F = \dfrac{{G{m_1}{m_2}}}{{{r^2}}}$
Where G is the gravitational force
${m_1}$ is the mass of the first object
${m_2}$ is the mass of the second object
And ‘r’ is the distance between them
Given that the length of each side of the square is = a
The distance of each corner from the centre will be $ = \dfrac{a}{{\sqrt 2 }}$

The gravitational force on point O due to point A is given by
\[\Rightarrow {F_1} = \dfrac{{Gmm}}{{{{(\dfrac{a}{{\sqrt 2 }})}^2}}} = \dfrac{{2G{m^2}}}{{{a^2}}}\]
Similarly, the gravitational force on point O due to point B is written as
$\Rightarrow {F_2} = \dfrac{{G(2m)(m)}}{{{{(\dfrac{a}{{\sqrt 2 }})}^2}}} = \dfrac{{4G{m^2}}}{{{a^2}}}$
The gravitational force on point O due to point C will be
$\Rightarrow {F_3} = \dfrac{{G(3m)(m)}}{{{{(\dfrac{a}{{\sqrt 2 }})}^2}}} = \dfrac{{6G{m^2}}}{{{a^2}}}$
The gravitational force on point O due to point D will be
$\Rightarrow {F_4} = \dfrac{{G(4m)(m)}}{{{{(\dfrac{a}{{\sqrt 2 }})}^2}}} = \dfrac{{8G{m^2}}}{{{a^2}}}$
The net force of ${F_1}$and ${F_3}$on point O is given by
$\Rightarrow {F_{13}} = {F_3} - {F_1}$
$\Rightarrow {F_{13}} = \dfrac{{6G{m^2}}}{{{a^2}}} - \dfrac{{2G{m^2}}}{{{a^2}}} = \dfrac{{4G{m^2}}}{{{a^2}}}$
Similarly, the force of ${F_2}$and${F_4}$on point O is given by
$\Rightarrow {F_{24}} = {F_4} - {F_2}$
$\Rightarrow {F_{24}} = \dfrac{{8G{m^2}}}{{{a^2}}} - \dfrac{{4G{m^2}}}{{{a^2}}} = \dfrac{{4G{m^2}}}{{{a^2}}}$
The magnitude of net force on point O is given by
$\Rightarrow F = \sqrt {{{({F_{13}})}^2} + {{({F_{24}})}^2}} $
$\Rightarrow F = \sqrt {{{(\dfrac{{4G{m^2}}}{{{a^2}}})}^2} + {{(\dfrac{{4G{m^2}}}{{{a^2}}})}^2}} $
$\Rightarrow F = \sqrt {2.\dfrac{{4{G^2}{m^4}}}{{{a^4}}}} $
\[\Rightarrow F = 2\sqrt 2 \dfrac{{G{m^2}}}{{{a^2}}}\]
Option C is the right answer.
Note: It is important to note that since the force of gravitation is directly proportional to the mass of the object, so an object with more mass will have a large force of gravitation. But it varies inversely with the square of distance, so if the distance is doubled the force of gravitation will decrease by 4 times and so on.
Recently Updated Pages
Chemical Equation - Important Concepts and Tips for JEE

JEE Main 2022 (July 29th Shift 1) Chemistry Question Paper with Answer Key

Conduction, Transfer of Energy Important Concepts and Tips for JEE

JEE Analytical Method of Vector Addition Important Concepts and Tips

Atomic Size - Important Concepts and Tips for JEE

JEE Main 2022 (June 29th Shift 1) Maths Question Paper with Answer Key

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

Equation of Trajectory in Projectile Motion: Derivation & Proof

Atomic Structure: Definition, Models, and Examples

How to Convert a Galvanometer into an Ammeter or Voltmeter

Hybridisation in Chemistry – Concept, Types & Applications

Angle of Deviation in a Prism – Formula, Diagram & Applications

Other Pages
Units And Measurements Class 11 Physics Chapter 1 CBSE Notes - 2025-26

Motion in a Straight Line Class 11 Physics Chapter 2 CBSE Notes - 2025-26

JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Motion In A Plane Class 11 Physics Chapter 3 CBSE Notes - 2025-26

NCERT Solutions For Class 11 Physics Chapter 1 Units and Measurements - 2025-26

NCERT Solutions for Class 11 Physics Chapter 3 Motion In A Plane 2025-26




