
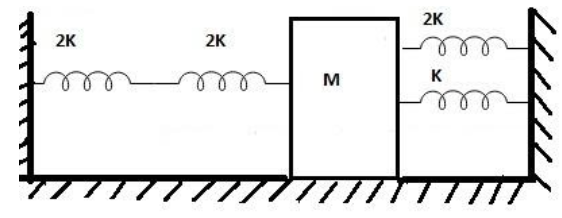
Four massless springs whose force constants are 2k, 2k, k and 2k respectively are attached to a mass M kept on a frictionless plane as shown in the figure. If the mass M is displaced in the horizontal direction, then the frequency of the system is:
A) $\dfrac{1}{{2\pi }}\sqrt {\dfrac{k}{{4M}}} $
B) $\dfrac{1}{{2\pi }}\sqrt {\dfrac{{4k}}{M}} $
C) $\dfrac{1}{{2\pi }}\sqrt {\dfrac{k}{{7M}}} $
D) $\dfrac{1}{{2\pi }}\sqrt {\dfrac{{7k}}{{7M}}} $
Answer
180k+ views
Hint: Formula for frequency is:
$\dfrac{1}{{2\pi }}\sqrt {\dfrac{1}{{LC}}} $ (L is the inductor, C is the capacitor)
As per electrical and mechanical analogy conversion, in force current analogy M is the capacitor (C) and k is the reciprocal of the inductor (1/L).
Let’s find the value of k using series and parallel connections (using 1/L = k, in series connections are added with their direct connections and the parallel connection have reciprocal addition).
Complete step by step solution:
As we are provided with an inductor and capacitor in the system then we will add the reciprocal of the inductor for the series connection.
$K = {K_1} + {K_2}$
First, we will do the calculation for series connection:
$
\Rightarrow {K_1} = \dfrac{1}{{2k}} + \dfrac{1}{{2k}} \\
\Rightarrow {K_1} = \dfrac{{2k \times 2k}}{{2k + 2k}}
$ (Taking LCM)
$ \Rightarrow {K_1} = \dfrac{{2k}}{{2k}} = 1k$
Now, we will calculate for the springs in parallel:
$
\Rightarrow {K_2} = \dfrac{1}{{\dfrac{1}{{2k}}}} + \dfrac{1}{{\dfrac{1}{k}}} \\
\Rightarrow {K_2} = 2k + k = 3k$ (in parallel connection we have to take the reciprocal of the spring constants)
Total value of K comes out to be:
$
\Rightarrow K = {K_1} + {K_2} \\
\Rightarrow K = k + 3k = 4k $
From the equation of frequency:
$
\Rightarrow f = \dfrac{1}{{2\pi }}\sqrt {\dfrac{1}{{M\dfrac{1}{K}}}} \\
\Rightarrow f = \dfrac{1}{{2\pi }}\sqrt {\dfrac{K}{M}} $ (We have substituted the value of LC as per formula of frequency)
$ \Rightarrow f = \dfrac{1}{{2\pi }}\sqrt {\dfrac{{4k}}{M}} $ (We have substituted the values M and K).
Hence, Option B is correct.
Note: In the question above we have used electrical to mechanical equivalent system of force current, where current is acting as the force in a mechanical system, mass as capacitor, frictional coefficient as reciprocal of R resistor, spring constant as reciprocal of L inductor, displacement as magnetic flux and velocity as voltage.
$\dfrac{1}{{2\pi }}\sqrt {\dfrac{1}{{LC}}} $ (L is the inductor, C is the capacitor)
As per electrical and mechanical analogy conversion, in force current analogy M is the capacitor (C) and k is the reciprocal of the inductor (1/L).
Let’s find the value of k using series and parallel connections (using 1/L = k, in series connections are added with their direct connections and the parallel connection have reciprocal addition).
Complete step by step solution:
As we are provided with an inductor and capacitor in the system then we will add the reciprocal of the inductor for the series connection.
$K = {K_1} + {K_2}$
First, we will do the calculation for series connection:
$
\Rightarrow {K_1} = \dfrac{1}{{2k}} + \dfrac{1}{{2k}} \\
\Rightarrow {K_1} = \dfrac{{2k \times 2k}}{{2k + 2k}}
$ (Taking LCM)
$ \Rightarrow {K_1} = \dfrac{{2k}}{{2k}} = 1k$
Now, we will calculate for the springs in parallel:
$
\Rightarrow {K_2} = \dfrac{1}{{\dfrac{1}{{2k}}}} + \dfrac{1}{{\dfrac{1}{k}}} \\
\Rightarrow {K_2} = 2k + k = 3k$ (in parallel connection we have to take the reciprocal of the spring constants)
Total value of K comes out to be:
$
\Rightarrow K = {K_1} + {K_2} \\
\Rightarrow K = k + 3k = 4k $
From the equation of frequency:
$
\Rightarrow f = \dfrac{1}{{2\pi }}\sqrt {\dfrac{1}{{M\dfrac{1}{K}}}} \\
\Rightarrow f = \dfrac{1}{{2\pi }}\sqrt {\dfrac{K}{M}} $ (We have substituted the value of LC as per formula of frequency)
$ \Rightarrow f = \dfrac{1}{{2\pi }}\sqrt {\dfrac{{4k}}{M}} $ (We have substituted the values M and K).
Hence, Option B is correct.
Note: In the question above we have used electrical to mechanical equivalent system of force current, where current is acting as the force in a mechanical system, mass as capacitor, frictional coefficient as reciprocal of R resistor, spring constant as reciprocal of L inductor, displacement as magnetic flux and velocity as voltage.
Recently Updated Pages
Electric Flux and Area Vector - Important Concepts for JEE

JEE Main 2023 (April 12th Shift 1) Physics Question Paper with Answer Key

Clemmensen and Wolff Kishner Reduction Important Concepts and Tips for JEE

All About Relations and Functions Complete Relation for JEE

Chain and Position Isomerism Important Concepts and Tips for JEE

JEE Main 2021 July 20 Shift 2 Question Paper with Answer Key

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Displacement-Time Graph and Velocity-Time Graph for JEE

Atomic Structure - Electrons, Protons, Neutrons and Atomic Models

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

What is Hybridisation in Chemistry?

Other Pages
NCERT Solutions For Class 11 Physics Chapter 2 Motion In A Straight Line - 2025-26

NCERT Solutions For Class 11 Physics Chapter 1 Units and Measurements - 2025-26

NCERT Solutions For Class 11 Physics Chapter 3 Motion In A Plane - 2025-26

JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Units And Measurements Class 11 Physics Chapter 1 CBSE Notes - 2025-26

Motion in a Straight Line Class 11 Physics Chapter 2 CBSE Notes - 2025-26




