
(a) The potential difference applied across a given resistor is altered so that the heat produced per second increases by a factor$9$. By what factor does the applied potential difference change?
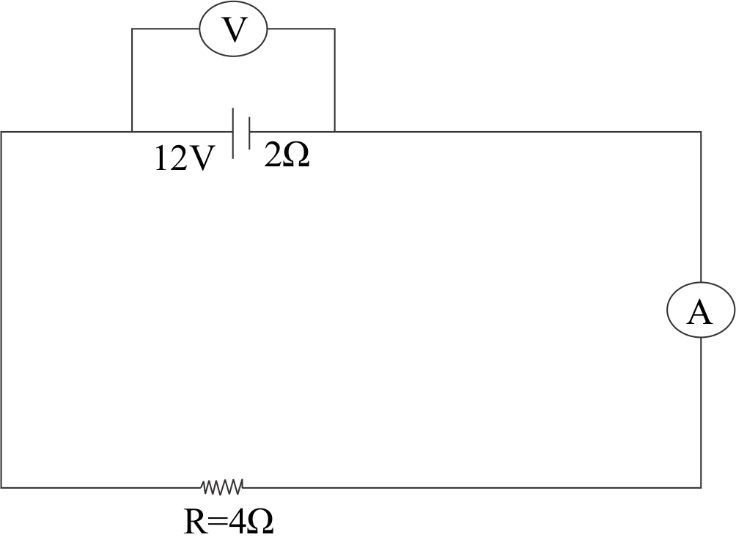
(b) In the figure shown, an ammeter A and a resistor are $4\Omega $ connected to the terminals of the source. The emf of the source is $12V$ having an internal resistance $2\Omega $. Calculate the voltmeter and ammeter reading.
Answer
118.8k+ views
Hint: Electric potential could be a location-dependent amount that expresses the number of potential energy per unit of charge at such location. Once a Coulomb of charge (or any given quantity of charge) possesses a comparatively great quantity of potential energy at a given location, then that location is alleged to be a location of high electric potential. And equally, if a Coulomb of charge (or any given quantity of charge) possesses a comparatively tiny amount of potential energy at a given location, then that location is alleged to be a location of low electric potential.
Formula used:
Heat produced,
$ \Rightarrow H = \dfrac{{{V^2}t}}{R}$
Where, $H$is the heat produced, $V$is the voltage, $t$is the time, and $R$is the resistance.
Voltmeter,
$ \Rightarrow V = R - IR$
Where, $R$ is the resistance, and $I$ is the ammeter.
Complete step by Step Solution In this question, the heat is altered and that causes the heat produced by is $9$ times its initial.
So they are asking the factor for which the applied potential difference changes.
$\left( a \right)$. Suppose the original potential difference applied to be $V$ and the original heat produced will be $H$.
Then,
$ \Rightarrow H = \dfrac{{{V^2}t}}{R}$
Now the new potential difference will be $V'$ and the heat produced after the change is $H'$
Then, we can write the equation as
$ \Rightarrow H' = \dfrac{{{{V'}^2}t}}{R}$
According to the question statement,
$ \Rightarrow H' = 9H$
Now we will put the values of both the heat,
We get
$ \Rightarrow \dfrac{{{{V'}^2}t}}{R} = 9 \times \dfrac{{{V^2}t}}{R}$
On further solving this equation, we get
$ \Rightarrow {V'^2} = 9{V^2}$
Which implies,
$ \Rightarrow V' = 3V$
Therefore we can say that the potential difference is increased by a factor $3$.
$\left( b \right)$. Let us consider the voltmeter and the ammeter to be in an ideal state.
Here the total resistance $R$ will be equal to
$ \Rightarrow R = 4 + 2$
$ \Rightarrow 6\Omega $
Then the ammeter reading will be,
$ \Rightarrow I = \dfrac{V}{R}$
$ \Rightarrow I = \dfrac{{12}}{6}$
$ \Rightarrow 2A$
Hence the ammeter reading will be$2A$.
Now we will calculate the voltmeter required,
$ \Rightarrow V = R - IR$
Substituting the values, we get
$ \Rightarrow 12 - 2 \times 2$
$ \Rightarrow 12 - 4$
$ \Rightarrow 8V$
Therefore the voltmeter reading will be $8V$.
Note: Voltmeters and ammeters measure the voltage and current, severally, of a circuit. Some meters in automobile dashboards, digital cameras, cell phones, and tuner-amplifiers are voltmeters or ammeters.
Formula used:
Heat produced,
$ \Rightarrow H = \dfrac{{{V^2}t}}{R}$
Where, $H$is the heat produced, $V$is the voltage, $t$is the time, and $R$is the resistance.
Voltmeter,
$ \Rightarrow V = R - IR$
Where, $R$ is the resistance, and $I$ is the ammeter.
Complete step by Step Solution In this question, the heat is altered and that causes the heat produced by is $9$ times its initial.
So they are asking the factor for which the applied potential difference changes.
$\left( a \right)$. Suppose the original potential difference applied to be $V$ and the original heat produced will be $H$.
Then,
$ \Rightarrow H = \dfrac{{{V^2}t}}{R}$
Now the new potential difference will be $V'$ and the heat produced after the change is $H'$
Then, we can write the equation as
$ \Rightarrow H' = \dfrac{{{{V'}^2}t}}{R}$
According to the question statement,
$ \Rightarrow H' = 9H$
Now we will put the values of both the heat,
We get
$ \Rightarrow \dfrac{{{{V'}^2}t}}{R} = 9 \times \dfrac{{{V^2}t}}{R}$
On further solving this equation, we get
$ \Rightarrow {V'^2} = 9{V^2}$
Which implies,
$ \Rightarrow V' = 3V$
Therefore we can say that the potential difference is increased by a factor $3$.
$\left( b \right)$. Let us consider the voltmeter and the ammeter to be in an ideal state.
Here the total resistance $R$ will be equal to
$ \Rightarrow R = 4 + 2$
$ \Rightarrow 6\Omega $
Then the ammeter reading will be,
$ \Rightarrow I = \dfrac{V}{R}$
$ \Rightarrow I = \dfrac{{12}}{6}$
$ \Rightarrow 2A$
Hence the ammeter reading will be$2A$.
Now we will calculate the voltmeter required,
$ \Rightarrow V = R - IR$
Substituting the values, we get
$ \Rightarrow 12 - 2 \times 2$
$ \Rightarrow 12 - 4$
$ \Rightarrow 8V$
Therefore the voltmeter reading will be $8V$.
Note: Voltmeters and ammeters measure the voltage and current, severally, of a circuit. Some meters in automobile dashboards, digital cameras, cell phones, and tuner-amplifiers are voltmeters or ammeters.
Recently Updated Pages
JEE Main 2023 January 25 Shift 1 Question Paper with Answer Key

Geostationary Satellites and Geosynchronous Satellites for JEE

Complex Numbers - Important Concepts and Tips for JEE

JEE Main 2023 (February 1st Shift 2) Maths Question Paper with Answer Key

JEE Main 2022 (July 25th Shift 2) Physics Question Paper with Answer Key

Inertial and Non-Inertial Frame of Reference for JEE

Trending doubts
JEE Main 2025: Application Form (Out), Exam Dates (Released), Eligibility & More

JEE Main Login 2045: Step-by-Step Instructions and Details

JEE Main Chemistry Question Paper with Answer Keys and Solutions

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

JEE Main Exam Marking Scheme: Detailed Breakdown of Marks and Negative Marking

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Dual Nature of Radiation and Matter Class 12 Notes CBSE Physics Chapter 11 (Free PDF Download)

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Degree of Dissociation and Its Formula With Solved Example for JEE

Diffraction of Light - Young’s Single Slit Experiment

JEE Main 2025: Derivation of Equation of Trajectory in Physics




