
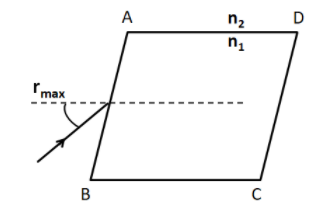
A rectangular glass slab ABCD of refractive index \[{n_1}\] is immersed in water of refractive index ${n_2}$ $\left( {{n_1} > {n_2}} \right)$. A ray of light is incident to the surface AB of the slab as shown. The maximum value of the angle of incidence ${r_{\max }}$ such that the ray comes out only from the another surface CD is given by
(A) ${\sin ^{ - 1}}\left[ {\dfrac{{{n_1}}}{{{n_2}}}\cos \left( {{{\sin }^{ - 1}}\left( {\dfrac{{{n_1}}}{{{n_2}}}} \right)} \right)} \right]$
(B) ${\sin ^{ - 1}}\left[ {{n_1}\cos \left( {{{\sin }^{ - 1}}\left( {\dfrac{1}{2}} \right)} \right)} \right]$
(C) \[{\sin ^{ - 1}}\left( {\dfrac{{{n_1}}}{{{n_2}}}} \right)\]
(D) \[{\sin ^{ - 1}}\left( {\dfrac{{{n_2}}}{{{n_1}}}} \right)\]
Answer
180k+ views
Hint: To solve this question, we need to apply the concept of total internal reflection within the glass slab. Then applying the Snell’s law at the interface AB we will get the answer.
Complete step-by-step solution:
For emerging out of the face CD of the slab, the angle of incidence should be such that the refracted ray suffers total internal reflection within the rectangular slab. As it will suffer multiple total internal reflections within the slab, it will reach the face CD and get refracted out of the slab.
We know that for total internal reflection to occur, the light ray must be incident at an angle greater or equal to the critical angle of incidence.
For the maximum angle ${r_{\max }}$, the ray will be incident at the critical angle of incidence.
Consider the following figure
In the triangle FED we have
$r + {i_c} = {90^ \circ }$
$ \Rightarrow r = {90^ \circ } - {i_c}$.................(1)
Applying Snell’s law at the interface AB, we have
\[{n_2}\sin {r_{\max }} = {n_1}\sin r\]
Putting (1) in above equation, we get
\[{n_2}\sin {r_{\max }} = {n_1}\sin \left( {{{90}^ \circ } - {i_c}} \right)\]
\[ \Rightarrow {n_2}\sin {r_{\max }} = {n_1}\cos {i_c}\].................(2)
Now, we know that the critical angle of incidence is equal to the sine inverse of the reciprocal of the refractive index of denser medium with respect to the rarer medium. So we have
${i_c} = {\sin ^{ - 1}}\left( {\dfrac{{{n_2}}}{{{n_1}}}} \right)$...........(3)
Putting (3) in (2) we get
\[{n_2}\sin {r_{\max }} = {n_1}\cos \left[ {{{\sin }^{ - 1}}\left( {\dfrac{{{n_2}}}{{{n_1}}}} \right)} \right]\]
\[ \Rightarrow \sin {r_{\max }} = \dfrac{{{n_1}}}{{{n_2}}}\cos \left[ {{{\sin }^{ - 1}}\left( {\dfrac{{{n_2}}}{{{n_1}}}} \right)} \right]\]
Taking sine inverse both the sides, we get
\[{r_{\max }} = {\sin ^{ - 1}}\left[ {\dfrac{{{n_1}}}{{{n_2}}}\cos \left( {{{\sin }^{ - 1}}\left( {\dfrac{{{n_2}}}{{{n_1}}}} \right)} \right)} \right]\]
Hence, the correct answer is option A.
Note: The phenomena of total internal reflection to get the light ray, which was incident on one face, transmitted to the opposite face is used in the optical fibre cables. The angle of incidence, which was ${r_{\max }}$ here, is known as the acceptance angle.
Complete step-by-step solution:
For emerging out of the face CD of the slab, the angle of incidence should be such that the refracted ray suffers total internal reflection within the rectangular slab. As it will suffer multiple total internal reflections within the slab, it will reach the face CD and get refracted out of the slab.
We know that for total internal reflection to occur, the light ray must be incident at an angle greater or equal to the critical angle of incidence.
For the maximum angle ${r_{\max }}$, the ray will be incident at the critical angle of incidence.
Consider the following figure
In the triangle FED we have
$r + {i_c} = {90^ \circ }$
$ \Rightarrow r = {90^ \circ } - {i_c}$.................(1)
Applying Snell’s law at the interface AB, we have
\[{n_2}\sin {r_{\max }} = {n_1}\sin r\]
Putting (1) in above equation, we get
\[{n_2}\sin {r_{\max }} = {n_1}\sin \left( {{{90}^ \circ } - {i_c}} \right)\]
\[ \Rightarrow {n_2}\sin {r_{\max }} = {n_1}\cos {i_c}\].................(2)
Now, we know that the critical angle of incidence is equal to the sine inverse of the reciprocal of the refractive index of denser medium with respect to the rarer medium. So we have
${i_c} = {\sin ^{ - 1}}\left( {\dfrac{{{n_2}}}{{{n_1}}}} \right)$...........(3)
Putting (3) in (2) we get
\[{n_2}\sin {r_{\max }} = {n_1}\cos \left[ {{{\sin }^{ - 1}}\left( {\dfrac{{{n_2}}}{{{n_1}}}} \right)} \right]\]
\[ \Rightarrow \sin {r_{\max }} = \dfrac{{{n_1}}}{{{n_2}}}\cos \left[ {{{\sin }^{ - 1}}\left( {\dfrac{{{n_2}}}{{{n_1}}}} \right)} \right]\]
Taking sine inverse both the sides, we get
\[{r_{\max }} = {\sin ^{ - 1}}\left[ {\dfrac{{{n_1}}}{{{n_2}}}\cos \left( {{{\sin }^{ - 1}}\left( {\dfrac{{{n_2}}}{{{n_1}}}} \right)} \right)} \right]\]
Hence, the correct answer is option A.
Note: The phenomena of total internal reflection to get the light ray, which was incident on one face, transmitted to the opposite face is used in the optical fibre cables. The angle of incidence, which was ${r_{\max }}$ here, is known as the acceptance angle.
Recently Updated Pages
Degree of Dissociation Important Concepts and Tips for JEE

JEE Main Chemistry Question Paper PDF Download with Answer Key

JEE Electricity and Magnetism Important Concepts and Tips for Exam Preparation

JEE Energetics Important Concepts and Tips for Exam Preparation

JEE Isolation, Preparation and Properties of Non-metals Important Concepts and Tips for Exam Preparation

States of Matter Chapter For JEE Main Chemistry

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Displacement-Time Graph and Velocity-Time Graph for JEE

Electric field due to uniformly charged sphere class 12 physics JEE_Main

Atomic Structure - Electrons, Protons, Neutrons and Atomic Models

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

What is Hybridisation in Chemistry?

JEE Main 2025: Conversion of Galvanometer Into Ammeter And Voltmeter in Physics

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

Wheatstone Bridge for JEE Main Physics 2025

Electric Field Due to Uniformly Charged Ring for JEE Main 2025 - Formula and Derivation




