
A capacitor is charged by a battery. The battery is removed and another identical uncharged capacitor is connected in parallel. The total electrostatic energy of the resulting system:
$\left( a \right)$ Increase by a factor of $2$
$\left( b \right)$ Increase by a factor of $4$
$\left( c \right)$ Decrease by a factor of $2$
$\left( d \right)$ Remains the same
Answer
198.6k+ views
Hint: Kirchhoff’s Voltage Law is that the second of his elementary laws we will use for circuit analysis. His voltage law states that for a closed-loop system series path the algebraically total of all the voltages around any closed-loop system in an exceeding circuit is adequate zero. This is often as a result of a circuit loop that could be a closed conducting path thus no energy is lost.
Complete step by step solution: Here in the question it is given that the capacitor is charged initially with the battery. For this, the diagram is shown below.
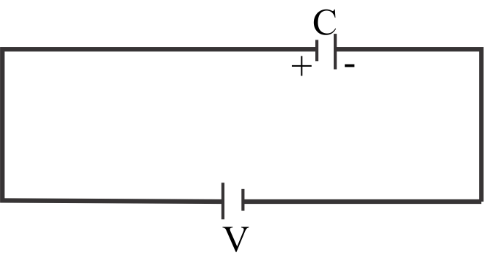
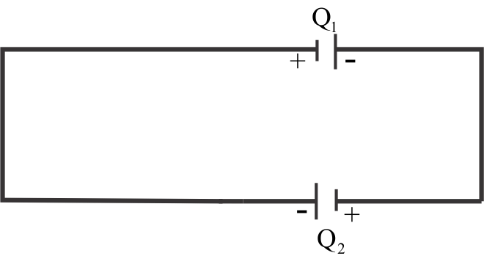
The total charge is equal to ${Q_0}$;
Therefore from the figure, we can write,
$ \Rightarrow {Q_1} + {Q_2} = {Q_0}$
Now applying KVL,
And as we know in KVL for a closed-loop system series path the algebraically total of all the voltages around any closed-loop system in an exceeding circuit is adequate zero.
Therefore,
\[ \Rightarrow \dfrac{{{Q_1}}}{C} = \dfrac{{{Q_2}}}{C} = 0\]
On further solving the equation, we can write it as;
$ \Rightarrow {Q_1} = {Q_2}$
And since ${Q_1} + {Q_2} = {Q_0}$
Therefore
$ \Rightarrow {Q_1} = {Q_2} = \dfrac{{{Q_0}}}{2}$
Since the initial electrostatic charge is,
\[ \Rightarrow {E_i} = \dfrac{1}{2}C{V^2} = \dfrac{{Q_0^2}}{{2C}}\]
Therefore, the final electrostatic charge will be
\[ \Rightarrow {E_f} = \dfrac{1}{2}C{V^2} + \dfrac{1}{2}C{V^2}\]
Now putting the values which we have calculated initially in the above equation, we get
\[ \Rightarrow \dfrac{{Q_1^2}}{{2C}} + \dfrac{{Q_2^2}}{{2C}}\]
On further solving,
\[ \Rightarrow \dfrac{{Q_0^2}}{{8C}} + \dfrac{{Q_0^2}}{{8C}}\]
Adding both the terms, we get
\[ \Rightarrow \dfrac{{Q_0^2}}{{4C}}\]
So now we will calculate the ratio of ${E_f}$and ${E_i}$
We get,
$\dfrac{{{E_f}}}{{{E_i}}} = \dfrac{{\dfrac{{Q_0^2}}{{4C}}}}{{\dfrac{{Q_0^2}}{{2C}}}}$
On solving this, we get
$\dfrac{{{E_f}}}{{{E_i}}} = \dfrac{1}{2}$
Therefore, the total electrostatic energy of the resulting system decrease by a factor $2$.
Note: The theory behind Kirchhoff’s second law is additionally called the law of conservation of voltage, and this is often notably helpful for us once managing series circuits, as series circuits conjointly act as voltage dividers and therefore the voltage divider circuit is a very important application of the many series circuits.
Complete step by step solution: Here in the question it is given that the capacitor is charged initially with the battery. For this, the diagram is shown below.


The total charge is equal to ${Q_0}$;
Therefore from the figure, we can write,
$ \Rightarrow {Q_1} + {Q_2} = {Q_0}$
Now applying KVL,
And as we know in KVL for a closed-loop system series path the algebraically total of all the voltages around any closed-loop system in an exceeding circuit is adequate zero.
Therefore,
\[ \Rightarrow \dfrac{{{Q_1}}}{C} = \dfrac{{{Q_2}}}{C} = 0\]
On further solving the equation, we can write it as;
$ \Rightarrow {Q_1} = {Q_2}$
And since ${Q_1} + {Q_2} = {Q_0}$
Therefore
$ \Rightarrow {Q_1} = {Q_2} = \dfrac{{{Q_0}}}{2}$
Since the initial electrostatic charge is,
\[ \Rightarrow {E_i} = \dfrac{1}{2}C{V^2} = \dfrac{{Q_0^2}}{{2C}}\]
Therefore, the final electrostatic charge will be
\[ \Rightarrow {E_f} = \dfrac{1}{2}C{V^2} + \dfrac{1}{2}C{V^2}\]
Now putting the values which we have calculated initially in the above equation, we get
\[ \Rightarrow \dfrac{{Q_1^2}}{{2C}} + \dfrac{{Q_2^2}}{{2C}}\]
On further solving,
\[ \Rightarrow \dfrac{{Q_0^2}}{{8C}} + \dfrac{{Q_0^2}}{{8C}}\]
Adding both the terms, we get
\[ \Rightarrow \dfrac{{Q_0^2}}{{4C}}\]
So now we will calculate the ratio of ${E_f}$and ${E_i}$
We get,
$\dfrac{{{E_f}}}{{{E_i}}} = \dfrac{{\dfrac{{Q_0^2}}{{4C}}}}{{\dfrac{{Q_0^2}}{{2C}}}}$
On solving this, we get
$\dfrac{{{E_f}}}{{{E_i}}} = \dfrac{1}{2}$
Therefore, the total electrostatic energy of the resulting system decrease by a factor $2$.
Note: The theory behind Kirchhoff’s second law is additionally called the law of conservation of voltage, and this is often notably helpful for us once managing series circuits, as series circuits conjointly act as voltage dividers and therefore the voltage divider circuit is a very important application of the many series circuits.
Recently Updated Pages
Chemical Equation - Important Concepts and Tips for JEE

JEE Main 2022 (July 29th Shift 1) Chemistry Question Paper with Answer Key

Conduction, Transfer of Energy Important Concepts and Tips for JEE

JEE Analytical Method of Vector Addition Important Concepts and Tips

Atomic Size - Important Concepts and Tips for JEE

JEE Main 2022 (June 29th Shift 1) Maths Question Paper with Answer Key

Trending doubts
Atomic Structure: Definition, Models, and Examples

Degree of Dissociation: Meaning, Formula, Calculation & Uses

Centre of Mass of a Semicircular Ring: Concept, Derivation & Uses

A passenger in an aeroplane shall A Never see a rainbow class 12 physics JEE_Main

Top 10 NIT Colleges in India 2025: Rankings, Courses, Eligibility and More

Exothermic Reactions: Real-Life Examples, Equations, and Uses

Other Pages
JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

Electric field due to uniformly charged sphere class 12 physics JEE_Main

JEE Advanced 2026 Notes

Navratri 2025 Colours 9 Days Significance of 9 Colours of Navratri

Important Days and Dates in October 2025 - Full List and Significance

Dhanteras 2025 Date Muhurat Meaning Puja Vidhi and Rangoli Ideas




