
The unit of angular displacement in SI units is
(a). Radians
(b). Degrees
(c). Degree Celsius
(d). Radians/sec
Answer
545.7k+ views
- Hint: To find the SI unit of angular displacement, understand its formula. With this formula, find the dimensional formula of angular displacement. Then find its units with help of its dimensional formula.
Complete step-by-step solution -
We all know about normal displacement, which tells you about the change in position of a body along a straight line. For example, if a body moves from point A to point B, then the displacement of that is the line joining the points A and B.
There is one more type of displacement called angular displacement. Sometimes, we tend to analyse certain cases with respect to a fixed point or fixed line (axis).
Then the moving body appears to be resolving around this fixed point/axis. This is where angular displacement comes into play. Now, we measure the change in the position of the body in terms of the distance of the body from the fixed point/axis and the angle the body makes with respect to the fixed point/axis while moving. This angle is called angular displacement. The SI unit of angular displacement is radians.
Now, let us consider a simple case. A body is revolving around a fixed point in a circular path with a fixed point as the centre. Suppose it moves from point A to point B, along the circular path, which makes arc AB. An angle is defined as the ratio of the length of the arc to the radius of the circle.
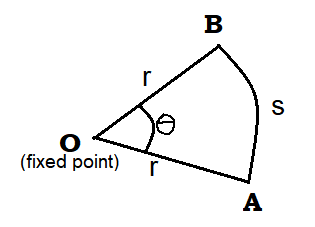
Therefore, $\theta =\dfrac{s}{r}$
The dimensional formula of angular displacement is $\dfrac{[L]}{[L]}=1$. Hence, it does not have any dimension and it is true that it will not have any units, but for some reason it has been given a SI unit i.e. radians. Other unit is degree.
Hence, the correct answer is (a) radians.
Note: It is important to note that in reality, the angular displacement has no unit but just to avoid confusion with other numbers it is represented with radians/degrees.
Complete step-by-step solution -
We all know about normal displacement, which tells you about the change in position of a body along a straight line. For example, if a body moves from point A to point B, then the displacement of that is the line joining the points A and B.
There is one more type of displacement called angular displacement. Sometimes, we tend to analyse certain cases with respect to a fixed point or fixed line (axis).
Then the moving body appears to be resolving around this fixed point/axis. This is where angular displacement comes into play. Now, we measure the change in the position of the body in terms of the distance of the body from the fixed point/axis and the angle the body makes with respect to the fixed point/axis while moving. This angle is called angular displacement. The SI unit of angular displacement is radians.
Now, let us consider a simple case. A body is revolving around a fixed point in a circular path with a fixed point as the centre. Suppose it moves from point A to point B, along the circular path, which makes arc AB. An angle is defined as the ratio of the length of the arc to the radius of the circle.

Therefore, $\theta =\dfrac{s}{r}$
The dimensional formula of angular displacement is $\dfrac{[L]}{[L]}=1$. Hence, it does not have any dimension and it is true that it will not have any units, but for some reason it has been given a SI unit i.e. radians. Other unit is degree.
Hence, the correct answer is (a) radians.
Note: It is important to note that in reality, the angular displacement has no unit but just to avoid confusion with other numbers it is represented with radians/degrees.
Recently Updated Pages
Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Trending doubts
1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

What is the opposite of entropy class 11 chemistry CBSE

Proton was discovered by A Thomson B Rutherford C Chadwick class 11 chemistry CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




