
The radial component of centripetal acceleration at poles of earth is?
Where $R$ is the radius of earth, $\omega $ is the angular velocity of earth)
$\begin{align}
& A.zero \\
& B.R{{\omega }^{2}} \\
& C.{{R}^{2}}\omega \\
& D.\infty \\
\end{align}$
Answer
553.5k+ views
Hint: The equation of centripetal acceleration should be found first. Then the radial components can be substituted in that equation. As we know the y components will be zero at the poles because the angle being there is zero.
Complete step-by-step answer:
The centripetal acceleration is given by the formula,
${{a}_{c}}=\dfrac{{{v}^{2}}}{r}$
Where $v$ be the velocity of the rotation of earth and $r$be the radius of earth.
When we take radial components into account, the above equation becomes,
\[{{a}_{c}}=\dfrac{-{{v}^{2}}}{r}\times \sin \theta \]
And also we know that, the velocity can be written in the form of angular velocity as,
\[v=r\omega \]
Substituting this in the equation of centripetal acceleration will give,
\[{{a}_{c}}=\dfrac{-{{\left( r\omega \right)}^{2}}}{r}\sin \theta \]
Simplifying this will give,
\[{{a}_{c}}=-r{{\omega }^{2}}\sin \theta \]
As we know that the angle at the poles in the y component is found to be zero.
Substituting this in the equation will give,
\[\begin{align}
& {{a}_{c}}=\dfrac{-{{\left( r\omega \right)}^{2}}}{r}\sin 0 \\
& {{a}_{c}}=0 \\
\end{align}\]
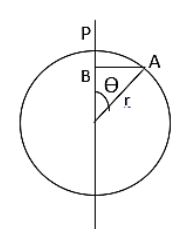
So, the correct answer is “Option A”.
Note: Centripetal acceleration is defined as the characteristics of the motion of a particle which is travelling in a circular path. The acceleration has been directed radially towards the centre of the circular path. Centripetal forces are the reason for the centripetal accelerations. In the circular motion of earth around the Sun or any satellite having circular motion around any celestial body, the centripetal force is there as a result of the gravitational attraction between them. At the equator there is always a subtractive force present because of the gravity opposite to this, towards the centre. At the poles, as there is no distance from the centre of rotation, no centrifugal force is there.
Complete step-by-step answer:
The centripetal acceleration is given by the formula,
${{a}_{c}}=\dfrac{{{v}^{2}}}{r}$
Where $v$ be the velocity of the rotation of earth and $r$be the radius of earth.
When we take radial components into account, the above equation becomes,
\[{{a}_{c}}=\dfrac{-{{v}^{2}}}{r}\times \sin \theta \]
And also we know that, the velocity can be written in the form of angular velocity as,
\[v=r\omega \]
Substituting this in the equation of centripetal acceleration will give,
\[{{a}_{c}}=\dfrac{-{{\left( r\omega \right)}^{2}}}{r}\sin \theta \]
Simplifying this will give,
\[{{a}_{c}}=-r{{\omega }^{2}}\sin \theta \]
As we know that the angle at the poles in the y component is found to be zero.
Substituting this in the equation will give,
\[\begin{align}
& {{a}_{c}}=\dfrac{-{{\left( r\omega \right)}^{2}}}{r}\sin 0 \\
& {{a}_{c}}=0 \\
\end{align}\]

So, the correct answer is “Option A”.
Note: Centripetal acceleration is defined as the characteristics of the motion of a particle which is travelling in a circular path. The acceleration has been directed radially towards the centre of the circular path. Centripetal forces are the reason for the centripetal accelerations. In the circular motion of earth around the Sun or any satellite having circular motion around any celestial body, the centripetal force is there as a result of the gravitational attraction between them. At the equator there is always a subtractive force present because of the gravity opposite to this, towards the centre. At the poles, as there is no distance from the centre of rotation, no centrifugal force is there.
Recently Updated Pages
Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Trending doubts
1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

The term brown air is used for A Acid fumes B Photochemical class 11 chemistry CBSE

Which of the following is not the state of the matter class 11 chemistry CBSE

Which of the following is not the state of matter ASolid class 11 chemistry CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




