Question

State Snell’s first and second law of refraction.

Answer 1

Hint: Refraction deals with bending of light at the interface of two mediums of different refractive index. So the angle associated with the incidence and refraction can have a relation to the refractive index of the two mediums.

Complete step by step answer:

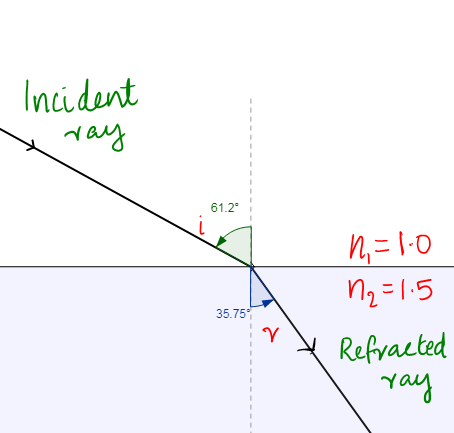
Snell’s First Law: It states that the incident ray, the refracted ray and the normal lie on the same plane.
Snell’s second law: It states that the ratio of the sines of the angles of incidence and refraction is equivalent to the ratio of phase velocities in the two media, or equivalent to the reciprocal of the ratio of the indices of refraction.
$\dfrac{\sin r}{\sin i}=\dfrac{{{\text{n}}_{\text{1}}}}{{{\text{n}}_{\text{2}}}}=\dfrac{{{\text{v}}_{\text{2}}}}{{{\text{v}}_{\text{1}}}}$
Where,
${{\text{v}}_{2}}$ is the phase velocity of light in medium 2.
${{\text{v}}_{1}}$ is the phase velocity of light in medium 1.
${{\text{n}}_{\text{1}}}$ is the refractive index of medium 1.
${{\text{n}}_{2}}$ is the refractive index of medium 2.
‘r’ is the angle of refraction.
‘i’ is the angle of incidence.
Additional Information:
Snell’s law is responsible for many physical phenomena like mirages, dispersion, apparent depth etc..
In optics, the refractive index of a material is a dimensionless number that describes how fast light travels through the material.

Note: Optical prisms and lenses use refraction to redirect light, as does the human eye. The refractive index of materials varies with the wavelength of light, and thus the angle of the refraction also varies correspondingly. This is called dispersion and causes prisms and rainbows to divide white light into its constituent spectral colors.