
Relation between coefficient of static friction ${{\text{ }\!\!\mu\!\!\text{ }}_{\text{S}}}$ and the angle of friction is
$\begin{align}
& \text{a) }{{\text{ }\!\!\mu\!\!\text{ }}_{\text{S}}}\text{=sin }\!\!\theta\!\!\text{ } \\
& \text{b) }{{\text{ }\!\!\mu\!\!\text{ }}_{\text{S}}}\text{=cos }\!\!\theta\!\!\text{ } \\
& \text{c) }{{\text{ }\!\!\mu\!\!\text{ }}_{\text{S}}}\text{=tan }\!\!\theta\!\!\text{ } \\
& \text{d) None of these} \\
\end{align}$
Answer
525k+ views
Hint: To obtain the relation between coefficient of friction and the angle of friction we need to know how we define the following. The coefficient to friction is basically responsible for frictional force that holds the body at rest. The angle of friction is defined as the angle between the resultant of the normal and the frictional force and the normal force of a body on ground. Now to obtain the relation, first we have drawn the diagram and using trigonometric definition we can obtain the required result.
Complete step-by-step answer:
To begin with let us consider a body of mass m resting on the ground as shown in the fig below.
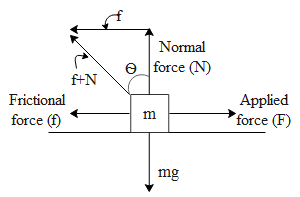
Let us say we applied a force F on the body at rest. The body will still be at rest due to the frictional force. At one point the frictional force will be equal to the applied force at equilibrium. The frictional force at this point is equal to ${{\text{ }\!\!\mu\!\!\text{ }}_{\text{S}}}$ times the normal force. This can mathematically be represented as,
$\begin{align}
& \text{f =}{{\text{ }\!\!\mu\!\!\text{ }}_{\text{S}}}\text{N} \;\;\;\;\;\;\;\text{Since N=mg} \\
& \text{f =}{{\text{ }\!\!\mu\!\!\text{ }}_{\text{S}}}\text{mg} \\
\end{align}$
If we see in the above diagram the angle between the resultant i.e. of( frictional force and the normal force)and the normal force is denoted by $\text{ }\!\!\theta\!\!\text{ }$. Now as we wish to calculate this angle let us write the parallel vector of frictional force to the head of the normal vector. Since the normal and the frictional force are perpendicular to each other by trigonometric definition of $\text{tan }\!\!\theta\!\!\text{ }$
We get,
$\text{tan }\!\!\theta\!\!\text{ }=\dfrac{\text{f}}{\text{N}}$ Since, $\text{f =}{{\text{ }\!\!\mu\!\!\text{ }}_{\text{S}}}\text{mg}$ and N= mg,
$\text{tan }\!\!\theta\!\!\text{ }=\dfrac{{{\text{ }\!\!\mu\!\!\text{ }}_{\text{S}}}\text{mg}}{\text{mg}}={{\text{ }\!\!\mu\!\!\text{ }}_{\text{S}}}$.
So, the correct answer is “Option C”.
Note:
It is to be noted the numerical value of angle of friction and the angle of repose is the same. The angle of repose is the angle between the resultant of the normal and the frictional force, and the normal force of a body on ground. If we observe this definition it is the same as the angle of friction but the difference is that the body itself is on an inclined surface and not horizontal surface.
Complete step-by-step answer:
To begin with let us consider a body of mass m resting on the ground as shown in the fig below.

Let us say we applied a force F on the body at rest. The body will still be at rest due to the frictional force. At one point the frictional force will be equal to the applied force at equilibrium. The frictional force at this point is equal to ${{\text{ }\!\!\mu\!\!\text{ }}_{\text{S}}}$ times the normal force. This can mathematically be represented as,
$\begin{align}
& \text{f =}{{\text{ }\!\!\mu\!\!\text{ }}_{\text{S}}}\text{N} \;\;\;\;\;\;\;\text{Since N=mg} \\
& \text{f =}{{\text{ }\!\!\mu\!\!\text{ }}_{\text{S}}}\text{mg} \\
\end{align}$
If we see in the above diagram the angle between the resultant i.e. of( frictional force and the normal force)and the normal force is denoted by $\text{ }\!\!\theta\!\!\text{ }$. Now as we wish to calculate this angle let us write the parallel vector of frictional force to the head of the normal vector. Since the normal and the frictional force are perpendicular to each other by trigonometric definition of $\text{tan }\!\!\theta\!\!\text{ }$
We get,
$\text{tan }\!\!\theta\!\!\text{ }=\dfrac{\text{f}}{\text{N}}$ Since, $\text{f =}{{\text{ }\!\!\mu\!\!\text{ }}_{\text{S}}}\text{mg}$ and N= mg,
$\text{tan }\!\!\theta\!\!\text{ }=\dfrac{{{\text{ }\!\!\mu\!\!\text{ }}_{\text{S}}}\text{mg}}{\text{mg}}={{\text{ }\!\!\mu\!\!\text{ }}_{\text{S}}}$.
So, the correct answer is “Option C”.
Note:
It is to be noted the numerical value of angle of friction and the angle of repose is the same. The angle of repose is the angle between the resultant of the normal and the frictional force, and the normal force of a body on ground. If we observe this definition it is the same as the angle of friction but the difference is that the body itself is on an inclined surface and not horizontal surface.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Trending doubts
1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

Whales are warmblooded animals which live in cold seas class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

How much is 23 kg in pounds class 11 chemistry CBSE

Explain zero factorial class 11 maths CBSE




