
Obtain the relation between linear velocity and angular velocity.
Answer
477.7k+ views
Hint: At first we need to find the formula for angular velocity. We need to draw figures that satisfy the question and help us in solving the question. After that we need to find the angular velocity for one complete rotation. Then we need to find the formula for linear velocity. Then need to replace some part of the formula with other relations that we will get while solving to get the required result.
Formulas used:
ds = v dt
$\omega =\dfrac{d\theta }{dt}$
$\omega =\dfrac{\theta }{t}=\dfrac{2\pi }{T}$
$d\theta =\dfrac{vdt}{r}$
$v=\omega r$
Complete step by step answer:
At first we need to know the definition of angular velocity,
The rate of change of angular displacement is known as the angular velocity of the particle.
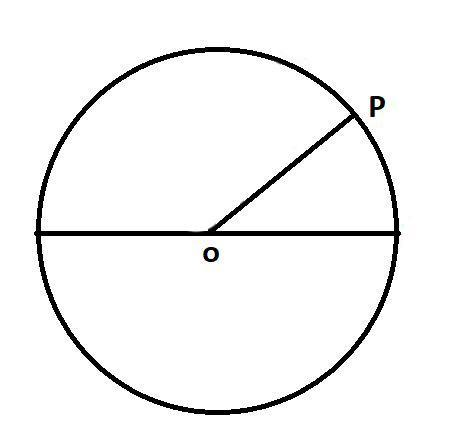
In the above diagram, P is the particle which is moving in a fixed circular path, now let us consider $d\theta $ , as the angular displacement made by the particle in a fixed time, let us consider that time as $dt$.
Then we know that, we can represent angular velocity the particle as,
$\omega =\dfrac{d\theta }{dt}$.
The dimensional formula for angular velocity of the particle is ${{T}^{-1}}$ and its SI unit is $rad\text{ }{{s}^{-1}}$.
Now if we consider that the particle has gone through a complete revolution, then the angle covered by the radius vector is $2\pi \text{ }radians$ or ${{360}^{\circ }}$. Now if time taken for one complete revolution is ‘T’ seconds, then the angular velocity of the particle is
$\omega =\dfrac{\theta }{t}=\dfrac{2\pi }{T}$
Now, if we say that the particle is making n revolutions per seconds, then the angular velocity will be,
$\omega =2\pi \left( \dfrac{1}{T} \right)=2\pi n$
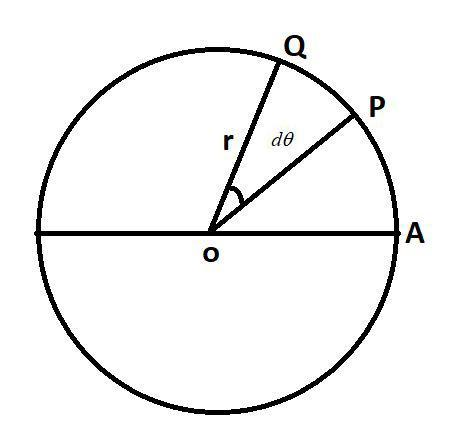
Now, in the above diagram, we see the particle is moving from point P to point Q, let us consider the distance as PQ=ds, and the angle covered by the particle is $d\theta $, so we can represent angular displacement $d\theta =\dfrac{ds}{r}$, but we know that ds = v dt.
$\dfrac{d\theta }{dt}=\dfrac{v}{r}$,
So, angular velocity is $\omega =\dfrac{v}{r}$ or $v=\omega r$.
Now, in vector notation, $\vec{v}=\vec{\omega }\times \vec{r}$
We already know that ds = v dt.
Therefore, $d\theta =\dfrac{vdt}{r}$ , or we can say
$\dfrac{d\theta }{dt}=\dfrac{v}{r}$ ,
So we can further solve it as,
Angular velocity, $\omega =\dfrac{v}{r}$or $v=\omega r$
In vector notation, $\vec{v}=\vec{\omega }\times \vec{r}$.
Therefore we can see that this is the relation between linear velocity and angular velocity.
Note:
We can say that for a given angular velocity, the linear velocity of the particle is directly proportional to the distance of the particle with respect to the centre of the circular path, that is we can write that for a body in a uniform circular motion, the angular velocity is different for different points of the body.
Formulas used:
ds = v dt
$\omega =\dfrac{d\theta }{dt}$
$\omega =\dfrac{\theta }{t}=\dfrac{2\pi }{T}$
$d\theta =\dfrac{vdt}{r}$
$v=\omega r$
Complete step by step answer:
At first we need to know the definition of angular velocity,
The rate of change of angular displacement is known as the angular velocity of the particle.

In the above diagram, P is the particle which is moving in a fixed circular path, now let us consider $d\theta $ , as the angular displacement made by the particle in a fixed time, let us consider that time as $dt$.
Then we know that, we can represent angular velocity the particle as,
$\omega =\dfrac{d\theta }{dt}$.
The dimensional formula for angular velocity of the particle is ${{T}^{-1}}$ and its SI unit is $rad\text{ }{{s}^{-1}}$.
Now if we consider that the particle has gone through a complete revolution, then the angle covered by the radius vector is $2\pi \text{ }radians$ or ${{360}^{\circ }}$. Now if time taken for one complete revolution is ‘T’ seconds, then the angular velocity of the particle is
$\omega =\dfrac{\theta }{t}=\dfrac{2\pi }{T}$
Now, if we say that the particle is making n revolutions per seconds, then the angular velocity will be,
$\omega =2\pi \left( \dfrac{1}{T} \right)=2\pi n$

Now, in the above diagram, we see the particle is moving from point P to point Q, let us consider the distance as PQ=ds, and the angle covered by the particle is $d\theta $, so we can represent angular displacement $d\theta =\dfrac{ds}{r}$, but we know that ds = v dt.
$\dfrac{d\theta }{dt}=\dfrac{v}{r}$,
So, angular velocity is $\omega =\dfrac{v}{r}$ or $v=\omega r$.
Now, in vector notation, $\vec{v}=\vec{\omega }\times \vec{r}$
We already know that ds = v dt.
Therefore, $d\theta =\dfrac{vdt}{r}$ , or we can say
$\dfrac{d\theta }{dt}=\dfrac{v}{r}$ ,
So we can further solve it as,
Angular velocity, $\omega =\dfrac{v}{r}$or $v=\omega r$
In vector notation, $\vec{v}=\vec{\omega }\times \vec{r}$.
Therefore we can see that this is the relation between linear velocity and angular velocity.
Note:
We can say that for a given angular velocity, the linear velocity of the particle is directly proportional to the distance of the particle with respect to the centre of the circular path, that is we can write that for a body in a uniform circular motion, the angular velocity is different for different points of the body.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Trending doubts
One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

How many whole numbers are between 437 and 487 left class 11 maths CBSE

In which place the first ozone hole was discovered class 11 social science CBSE

Why should you not see the solar eclipse directly A class 11 physics CBSE




