
In an \[LCR\] series a.c. circuit, the voltage across each of the components \[L,{\text{ }}C\] and \[R\] is \[50{\text{ }}V\]. The voltage across the \[LC\] combination will be:
A) $50 V$
B) $50\sqrt 2 V$
C) $100 V$
D) $0 V$ (zero)
Answer
532.2k+ views
Hint: In \[LCR\] series a.c circuit, the components \[L,{\text{ }}C\] and \[R\] are related to each other in a way that the voltage across the inductor \[L\](${V_L}$) and voltage across the capacitor \[C\](${V_C}$) has a constant phase difference. The voltage across the resistance \[R\](${V_R}$) and current($i$) does not have any phase difference.
Complete step by step solution:
According to the question, a \[LCR\] series a.c. circuit is given. The voltage across \[L,{\text{ }}C\] and \[R\] is \[50{\text{ }}V\].
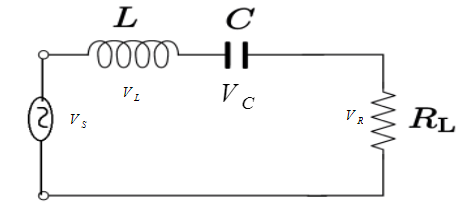
We know that in an \[LCR\] series circuit, the voltage across the inductor \[L\](${V_L}$) leads the current($i$) by \[{90^ \circ }\] and voltage across the capacitor \[C\](${V_C}$) lags the current($i$) by \[{90^ \circ }\]. So, the inductance and the capacitance are in opposite phases. In an \[LCR\] series circuit, the voltage across the resistance \[R\](${V_R}$) is in the same phase with current($i$).
So, the voltage across the \[LC\] combination will be given as:
$
{V_{LC}} = {V_L} - {V_C} \\
\Rightarrow {V_{LC}} = 50 - 50 \\
\Rightarrow {V_{LC}} = 0 \\
$
To understand the phase difference in different voltages, we can make a graph which shows the phase difference between voltages.
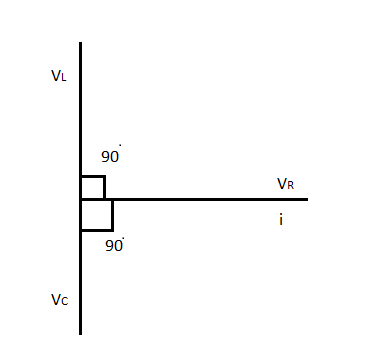
According to the above graph, ${V_R}$ and $i$ are in the same phase. ${V_L}$leads current $i$ by \[{90^ \circ }\] and ${V_C}$ lags current $i$ by \[{90^ \circ }\]. So, the voltage across \[LC\] combination is zero.
Hence, option (D) is correct.
Note: Voltage across the inductor ${V_L}$ and current $i$ has a phase difference. Voltage across the capacitor ${V_C}$ and current $i$ has a phase difference. The voltage across the resistance ${V_R}$ and current $i$ has zero phase difference. According to the graph, current $i$ and voltage across the resistance ${V_R}$ are plotted on X-axis.
Complete step by step solution:
According to the question, a \[LCR\] series a.c. circuit is given. The voltage across \[L,{\text{ }}C\] and \[R\] is \[50{\text{ }}V\].
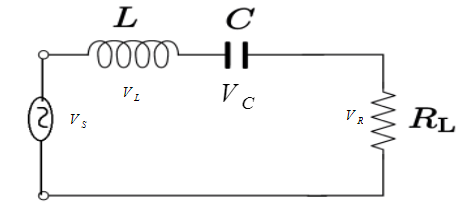
We know that in an \[LCR\] series circuit, the voltage across the inductor \[L\](${V_L}$) leads the current($i$) by \[{90^ \circ }\] and voltage across the capacitor \[C\](${V_C}$) lags the current($i$) by \[{90^ \circ }\]. So, the inductance and the capacitance are in opposite phases. In an \[LCR\] series circuit, the voltage across the resistance \[R\](${V_R}$) is in the same phase with current($i$).
So, the voltage across the \[LC\] combination will be given as:
$
{V_{LC}} = {V_L} - {V_C} \\
\Rightarrow {V_{LC}} = 50 - 50 \\
\Rightarrow {V_{LC}} = 0 \\
$
To understand the phase difference in different voltages, we can make a graph which shows the phase difference between voltages.

According to the above graph, ${V_R}$ and $i$ are in the same phase. ${V_L}$leads current $i$ by \[{90^ \circ }\] and ${V_C}$ lags current $i$ by \[{90^ \circ }\]. So, the voltage across \[LC\] combination is zero.
Hence, option (D) is correct.
Note: Voltage across the inductor ${V_L}$ and current $i$ has a phase difference. Voltage across the capacitor ${V_C}$ and current $i$ has a phase difference. The voltage across the resistance ${V_R}$ and current $i$ has zero phase difference. According to the graph, current $i$ and voltage across the resistance ${V_R}$ are plotted on X-axis.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

What is transplantation in agriculture class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Hydrological cycle is controlled by A Grasslands B class 12 biology CBSE

Who discovered the cell and how class 12 biology CBSE




