
Derive lens maker formula for a convex lens.
Answer
496.2k+ views
Hint: For making a lens of desired focal length, the lens maker formula is used. It has to be specified, the refractive index of the lens material, refractive index of the surroundings, and the radii of curvatures of two spheres of which the lens is a part of. We will use the expression for the refraction of lightwave on a spherical surface to find the required lens maker formula.
Formula used:
Refraction at first surface, $\dfrac{{{n}_{2}}}{{{v}_{1}}}-\dfrac{{{n}_{1}}}{u}=\dfrac{{{n}_{2}}-{{n}_{1}}}{{{R}_{1}}}$
Refraction at second surface, $\dfrac{{{n}_{1}}}{v}-\dfrac{{{n}_{2}}}{{{v}_{1}}}=\dfrac{{{n}_{1}}-{{n}_{2}}}{{{R}_{2}}}$
Complete step by step answer:
Lenses having different values of focal lengths are used for making various optical instruments. The focal length of an optical lens depends upon the refractive index of the material which was used to make the lens and the radii of curvatures of the two surfaces, of which the lens is a part of.
Assumptions for lens maker formula,
Let us consider a thin lens with two refracting surfaces having radii of curvatures ${{R}_{1}}$ and ${{R}_{2}}$. Let the refractive index of the surrounding be ${{n}_{1}}$ and that of the lens be ${{n}_{2}}$.
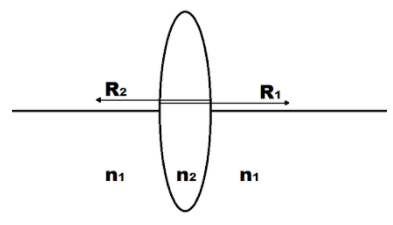
With the help of formula for refraction at a single spherical surface,
For the first surface, we have,
$\dfrac{{{n}_{2}}}{{{v}_{1}}}-\dfrac{{{n}_{1}}}{u}=\dfrac{{{n}_{2}}-{{n}_{1}}}{{{R}_{1}}}$
For the second surface, we have,
$\dfrac{{{n}_{1}}}{v}-\dfrac{{{n}_{2}}}{{{v}_{1}}}=\dfrac{{{n}_{1}}-{{n}_{2}}}{{{R}_{2}}}$
Adding the above two equations,
$\dfrac{{{n}_{1}}}{v}-\dfrac{{{n}_{1}}}{u}=\left( {{n}_{2}}-{{n}_{1}} \right)\left( \dfrac{1}{{{R}_{1}}}-\dfrac{1}{{{R}_{2}}} \right)$
$\dfrac{1}{v}-\dfrac{1}{u}=\left( \dfrac{{{n}_{2}}}{{{n}_{1}}}-1 \right)\left[ \dfrac{1}{{{R}_{1}}}-\dfrac{1}{{{R}_{2}}} \right]$
When $u=\infty $ and $v=f$
We get,
\[\dfrac{1}{f}=\left( \dfrac{{{n}_{2}}}{{{n}_{1}}}-1 \right)\left[ \dfrac{1}{{{R}_{1}}}-\dfrac{1}{{{R}_{2}}} \right]\]
Therefore,
\[\dfrac{1}{f}=\left( \mu -1 \right)\left( \dfrac{1}{{{R}_{1}}}-\dfrac{1}{{{R}_{2}}} \right)\]
Where, $\mu $ is the refractive index of the material.
The above equation is known as the Lens maker formula.
Lens manufacturers commonly take the help of the lens maker formula for manufacturing the lenses of the desired focal length.
Additional information:
In optics, the relation between the distance of an image $(v)$, the distance of an object $(u)$, and the focal length of the lens is given by the formula, called Lens maker formula. Lens formula is applicable for all types of lenses, Plano and Bi convex and Plano and Bi concave lenses. These lenses are assumed to have negligible thickness.
Expression for Lens maker formula:
$\dfrac{1}{v}-\dfrac{1}{u}=\dfrac{1}{f}$
Derivation for lens maker formula:
Consider a convex lens having an optical centre at $\text{O}$. Let $\text{F}$ be the principal focus of the lens and $f$ is the focal length of the lens. An object $\text{AB}$ is placed perpendicular to the principal axis of the lens at a distance beyond the focal length $f$ of the lens.
A real, inverted, and magnified image $\text{A }\!\!'\!\!\text{ B }\!\!'\!\!\text{ }$ of the object $\text{AB}$ is formed.
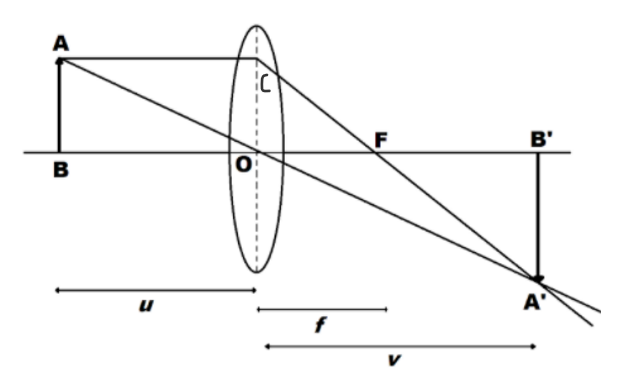
From the above figure,
$\vartriangle \text{ABO}$ is similar to $\vartriangle \text{A }\!\!'\!\!\text{ B }\!\!'\!\!\text{ O}$
Therefore,
$\dfrac{\text{A }\!\!'\!\!\text{ B }\!\!'\!\!\text{ }}{\text{AB}}\text{=}\dfrac{\text{OB }\!\!'\!\!\text{ }}{\text{OB}}$
Similarly,
$\vartriangle \text{A }\!\!'\!\!\text{ B }\!\!'\!\!\text{ F}$ is similar to $\vartriangle \text{OCF}$
Therefore,
$\dfrac{\text{A }\!\!'\!\!\text{ B }\!\!'\!\!\text{ }}{\text{OC}}\text{=}\dfrac{\text{FB}}{\text{OF}}$
Also, $\text{OC=AB}$
Thus,
$\dfrac{\text{A }\!\!'\!\!\text{ B }\!\!'\!\!\text{ }}{\text{OC}}\text{=}\dfrac{\text{FB }\!\!'\!\!\text{ }}{\text{OF}}$
Equating the above equations, we get,
$\dfrac{\text{OB }\!\!'\!\!\text{ }}{\text{OB}}\text{=}\dfrac{\text{FB }\!\!'\!\!\text{ }}{\text{OF}}$
$\text{FB }\!\!'\!\!\text{ = OB }\!\!'\!\!\text{ - OF}$
Therefore,
$\dfrac{\text{OB }\!\!'\!\!\text{ }}{\text{OB}}\text{=}\dfrac{\text{OB }\!\!'\!\!\text{ -OF}}{\text{OF}}$
Substituting the sign convention, we get,
$\begin{align}
& \text{OB}=-u \\
& \text{OB }\!\!'\!\!\text{ }=v \\
& \text{OF}=f \\
\end{align}$
$\dfrac{-v}{u}=\dfrac{v-f}{f}$
$\begin{align}
& vf=-uv+uf \\
& uv=uf-vf \\
\end{align}$
Diving both the sides by$uvf$,
$\dfrac{uv}{uvf}=\dfrac{uf}{uvf}-\dfrac{vf}{uvf}$
$\dfrac{1}{f}=\dfrac{1}{v}-\dfrac{1}{u}$
The above equation is known as Lens formula.
Note: While deriving the lens formula, it should be remembered that the image distance from the refraction at the first surface becomes the object distance for the refraction at the second surface since the light ray is passing undisturbed from the lens. Also, the refractive indices of the two spherical materials, of which the lens is a part of, can also be different. In the above derivation, we have taken the refractive indices of both the material as the same.
Formula used:
Refraction at first surface, $\dfrac{{{n}_{2}}}{{{v}_{1}}}-\dfrac{{{n}_{1}}}{u}=\dfrac{{{n}_{2}}-{{n}_{1}}}{{{R}_{1}}}$
Refraction at second surface, $\dfrac{{{n}_{1}}}{v}-\dfrac{{{n}_{2}}}{{{v}_{1}}}=\dfrac{{{n}_{1}}-{{n}_{2}}}{{{R}_{2}}}$
Complete step by step answer:
Lenses having different values of focal lengths are used for making various optical instruments. The focal length of an optical lens depends upon the refractive index of the material which was used to make the lens and the radii of curvatures of the two surfaces, of which the lens is a part of.
Assumptions for lens maker formula,
Let us consider a thin lens with two refracting surfaces having radii of curvatures ${{R}_{1}}$ and ${{R}_{2}}$. Let the refractive index of the surrounding be ${{n}_{1}}$ and that of the lens be ${{n}_{2}}$.

With the help of formula for refraction at a single spherical surface,
For the first surface, we have,
$\dfrac{{{n}_{2}}}{{{v}_{1}}}-\dfrac{{{n}_{1}}}{u}=\dfrac{{{n}_{2}}-{{n}_{1}}}{{{R}_{1}}}$
For the second surface, we have,
$\dfrac{{{n}_{1}}}{v}-\dfrac{{{n}_{2}}}{{{v}_{1}}}=\dfrac{{{n}_{1}}-{{n}_{2}}}{{{R}_{2}}}$
Adding the above two equations,
$\dfrac{{{n}_{1}}}{v}-\dfrac{{{n}_{1}}}{u}=\left( {{n}_{2}}-{{n}_{1}} \right)\left( \dfrac{1}{{{R}_{1}}}-\dfrac{1}{{{R}_{2}}} \right)$
$\dfrac{1}{v}-\dfrac{1}{u}=\left( \dfrac{{{n}_{2}}}{{{n}_{1}}}-1 \right)\left[ \dfrac{1}{{{R}_{1}}}-\dfrac{1}{{{R}_{2}}} \right]$
When $u=\infty $ and $v=f$
We get,
\[\dfrac{1}{f}=\left( \dfrac{{{n}_{2}}}{{{n}_{1}}}-1 \right)\left[ \dfrac{1}{{{R}_{1}}}-\dfrac{1}{{{R}_{2}}} \right]\]
Therefore,
\[\dfrac{1}{f}=\left( \mu -1 \right)\left( \dfrac{1}{{{R}_{1}}}-\dfrac{1}{{{R}_{2}}} \right)\]
Where, $\mu $ is the refractive index of the material.
The above equation is known as the Lens maker formula.
Lens manufacturers commonly take the help of the lens maker formula for manufacturing the lenses of the desired focal length.
Additional information:
In optics, the relation between the distance of an image $(v)$, the distance of an object $(u)$, and the focal length of the lens is given by the formula, called Lens maker formula. Lens formula is applicable for all types of lenses, Plano and Bi convex and Plano and Bi concave lenses. These lenses are assumed to have negligible thickness.
Expression for Lens maker formula:
$\dfrac{1}{v}-\dfrac{1}{u}=\dfrac{1}{f}$
Derivation for lens maker formula:
Consider a convex lens having an optical centre at $\text{O}$. Let $\text{F}$ be the principal focus of the lens and $f$ is the focal length of the lens. An object $\text{AB}$ is placed perpendicular to the principal axis of the lens at a distance beyond the focal length $f$ of the lens.
A real, inverted, and magnified image $\text{A }\!\!'\!\!\text{ B }\!\!'\!\!\text{ }$ of the object $\text{AB}$ is formed.

From the above figure,
$\vartriangle \text{ABO}$ is similar to $\vartriangle \text{A }\!\!'\!\!\text{ B }\!\!'\!\!\text{ O}$
Therefore,
$\dfrac{\text{A }\!\!'\!\!\text{ B }\!\!'\!\!\text{ }}{\text{AB}}\text{=}\dfrac{\text{OB }\!\!'\!\!\text{ }}{\text{OB}}$
Similarly,
$\vartriangle \text{A }\!\!'\!\!\text{ B }\!\!'\!\!\text{ F}$ is similar to $\vartriangle \text{OCF}$
Therefore,
$\dfrac{\text{A }\!\!'\!\!\text{ B }\!\!'\!\!\text{ }}{\text{OC}}\text{=}\dfrac{\text{FB}}{\text{OF}}$
Also, $\text{OC=AB}$
Thus,
$\dfrac{\text{A }\!\!'\!\!\text{ B }\!\!'\!\!\text{ }}{\text{OC}}\text{=}\dfrac{\text{FB }\!\!'\!\!\text{ }}{\text{OF}}$
Equating the above equations, we get,
$\dfrac{\text{OB }\!\!'\!\!\text{ }}{\text{OB}}\text{=}\dfrac{\text{FB }\!\!'\!\!\text{ }}{\text{OF}}$
$\text{FB }\!\!'\!\!\text{ = OB }\!\!'\!\!\text{ - OF}$
Therefore,
$\dfrac{\text{OB }\!\!'\!\!\text{ }}{\text{OB}}\text{=}\dfrac{\text{OB }\!\!'\!\!\text{ -OF}}{\text{OF}}$
Substituting the sign convention, we get,
$\begin{align}
& \text{OB}=-u \\
& \text{OB }\!\!'\!\!\text{ }=v \\
& \text{OF}=f \\
\end{align}$
$\dfrac{-v}{u}=\dfrac{v-f}{f}$
$\begin{align}
& vf=-uv+uf \\
& uv=uf-vf \\
\end{align}$
Diving both the sides by$uvf$,
$\dfrac{uv}{uvf}=\dfrac{uf}{uvf}-\dfrac{vf}{uvf}$
$\dfrac{1}{f}=\dfrac{1}{v}-\dfrac{1}{u}$
The above equation is known as Lens formula.
Note: While deriving the lens formula, it should be remembered that the image distance from the refraction at the first surface becomes the object distance for the refraction at the second surface since the light ray is passing undisturbed from the lens. Also, the refractive indices of the two spherical materials, of which the lens is a part of, can also be different. In the above derivation, we have taken the refractive indices of both the material as the same.
Recently Updated Pages
Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Draw a labelled sketch of the human eye class 12 physics CBSE

Which animal never drinks water in its entire life class 12 biology CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?

What are the major means of transport Explain each class 12 social science CBSE




