
Define centripetal acceleration. Derive an expression for the centripetal acceleration of a particle moving with uniform speed $v$ along a circular path of radius $r$. Give the direction of this acceleration.
Answer
549.6k+ views
Hint
The centripetal acceleration will occur due to the centripetal force, which mostly occurs in the circular motion of the object. The centripetal acceleration is also called radial acceleration. The centripetal acceleration acts towards the centre of the circular path.
Complete step by step answer
The centripetal acceleration is the type of acceleration which is due to the centripetal force of the body which is moving in the circular path. This centripetal acceleration will act towards the centre of the circular path. The centripetal acceleration is denoted by ${a_c}$.
Now, the centripetal force is given by,
$\Rightarrow F = \dfrac{{m{v^2}}}{r}$
Where, $F$ is the centripetal force, $m$ is the mass of the object, $v$ is the velocity or speed of the object and $r$ is the radius of the circular path.
The acceleration is given by,
$\Rightarrow {a_c} = \dfrac{F}{m}$
By substituting the centripetal force in the centripetal acceleration equation, then the centripetal acceleration is written as,
$\Rightarrow {a_c} = \dfrac{{\left( {\dfrac{{m{v^2}}}{r}} \right)}}{m}$
By cancelling the same terms in the above equation, then the above equation of the centripetal acceleration is given as,
$\Rightarrow {a_c} = \dfrac{{{v^2}}}{r}$
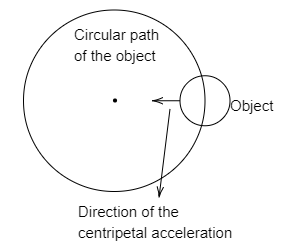
Thus, the above equation shows the centripetal acceleration due to the centripetal force acting on the object which is moving in the circular path.
And when the object is moving in the circular path the velocity of the object always acts tangent to the circular path and the centripetal force acts towards the centre of the circular path.
Note
When a body is in uniform circular motion, its direction is changed continuously when the speed of the object is constant. When the velocity of the object changes continuously, there is an acceleration in the circular motion, that acceleration called centripetal acceleration.
The centripetal acceleration will occur due to the centripetal force, which mostly occurs in the circular motion of the object. The centripetal acceleration is also called radial acceleration. The centripetal acceleration acts towards the centre of the circular path.
Complete step by step answer
The centripetal acceleration is the type of acceleration which is due to the centripetal force of the body which is moving in the circular path. This centripetal acceleration will act towards the centre of the circular path. The centripetal acceleration is denoted by ${a_c}$.
Now, the centripetal force is given by,
$\Rightarrow F = \dfrac{{m{v^2}}}{r}$
Where, $F$ is the centripetal force, $m$ is the mass of the object, $v$ is the velocity or speed of the object and $r$ is the radius of the circular path.
The acceleration is given by,
$\Rightarrow {a_c} = \dfrac{F}{m}$
By substituting the centripetal force in the centripetal acceleration equation, then the centripetal acceleration is written as,
$\Rightarrow {a_c} = \dfrac{{\left( {\dfrac{{m{v^2}}}{r}} \right)}}{m}$
By cancelling the same terms in the above equation, then the above equation of the centripetal acceleration is given as,
$\Rightarrow {a_c} = \dfrac{{{v^2}}}{r}$
Thus, the above equation shows the centripetal acceleration due to the centripetal force acting on the object which is moving in the circular path.
And when the object is moving in the circular path the velocity of the object always acts tangent to the circular path and the centripetal force acts towards the centre of the circular path.

Note
When a body is in uniform circular motion, its direction is changed continuously when the speed of the object is constant. When the velocity of the object changes continuously, there is an acceleration in the circular motion, that acceleration called centripetal acceleration.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
What is the difference between lightdependent and lightindependent class 11 biology CBSE

Which of the following is not the state of the matter class 11 chemistry CBSE

Which of the following is not the state of matter ASolid class 11 chemistry CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

How much is 23 kg in pounds class 11 chemistry CBSE

Draw a diagram of a plant cell and label at least eight class 11 biology CBSE




