Question

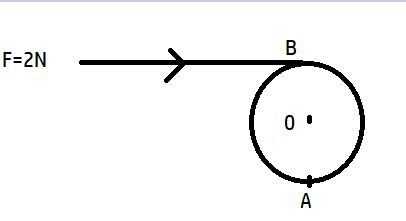
A wheel of diameter 2 m is shown with the axle at 'O'. A force F=2 N is applied at B in the direction as shown in the figure.
Calculate the moment of force about
(i) centre 'O', and
(ii) point A
A. 2 Nm and 4 Nm
B. 2 Nm and 2 Nm
C. 4 Nm and 4 Nm
D. 4 Nm and 2 Nm

Answer 1

Hint: Here we need to find the moment of force and that two at two different points. First of all let us define what is a moment of force. The moment of force is a measure of the ability of the force acting on a particular body to rotate it around a given axis.

Complete step by step answer:
The moment of force is also known as the torque. In order for a torque to develop, the force must act upon the body in such a manner that the body would begin to rotate.
Torque = \[\overrightarrow{\tau }=\overrightarrow{r}\times \overrightarrow{F}\]
The cross product involves the angle between the two vectors and here in both the cases the vectors are perpendicular to each other.
So, the magnitude of torque can be given by \[\tau =rF\]
Diameter is 2m, so the radius will be 1 m just half of the dimeter
For first case:
\[\begin{align}
& \overrightarrow{\tau }=\overrightarrow{r}\times \overrightarrow{F} \\
 & \tau =1\times 2=2Nm \\
\end{align}\]
For second case:
\[\tau =2\times 2=4Nm\].

So, the correct answer is “Option A”.

Note:
Moment of force is a vector quantity and in order to find out the direction of the torque acting on a body we have to take the cross product of the two vectors and the resultant vector gives us the direction of the torque acting on the body.