Question

Three charges $ - \sqrt 2 \mu C,2\sqrt 2 \mu C$and $ - \sqrt 2 \mu C$ are arranged as shown. Total electric field intensity at P is:

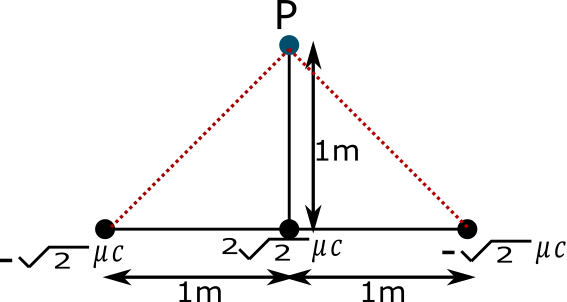
A) $18 \times {10^3}N/C$
B) $\left( {2\sqrt 2 - 1} \right) \times 9 \times {10^3}N/C$
C) Zero
D) $\left( {2\sqrt 2 + 1} \right) \times 9 \times {10^3}N/C$

Answer 1

Hint: We simply apply here formula for electric field intensity at a point due to a point charge. Here in question three charges are given so we find three electric field intensity on point P. To find the net electric field on point P can be calculated by vector addition of all three electric fields.

Complete step by step solution:
Step 1
First we draw diagram mark electric field direction due to all three charges


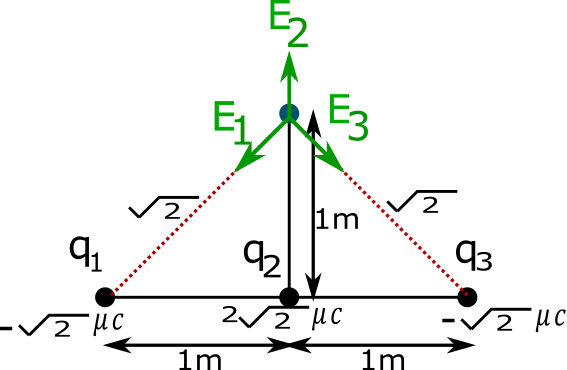
Here charge ${q_1} = - \sqrt 2 \mu C,{q_2} = 2\sqrt 2 \mu C$ and ${q_3} = - \sqrt 2 \mu C$
Electric field due to charge ${q_1}$ is ${E_1}$ toward charge because of negative charge
Electric field due to charge ${q_2}$ is ${E_2}$ away from ${q_2}$ because of positive charge
Electric field due to ${q_3}$ is ${E_3}$ toward ${q_3}$ because of negative charge
Electric field at a point due to a point charge is given by $E = \dfrac{{kq}}{{{r^2}}}$
Here E is the electric field
k is a constant $k = 9 \times {10^9}$
$r$ Is the distance between charge and point
Calculate ${E_1}$
$ \Rightarrow {E_1} = \dfrac{{k{q_1}}}{{{r^2}}}$
Here $r = \sqrt 2 $ from figure
$ \Rightarrow {E_1} = \dfrac{{k\sqrt 2 \times {{10}^{ - 6}}C}}{{{{\left( {\sqrt 2 } \right)}^2}}}$
$ \Rightarrow {E_1} = \dfrac{{k \times {{10}^{ - 6}}}}{{\sqrt 2 }}N/C$ At ${45^\circ }$ from negative y-axis toward negative x-axis
Electric field due to ${q_2}$
$ \Rightarrow {E_2} = \dfrac{{k{q_2}}}{{{r^2}}}$
Here $r = 1m$ given in question
$ \Rightarrow {E_2} = \dfrac{{k \times 2\sqrt 2 \times {{10}^{ - 6}}}}{1}$
$ \Rightarrow {E_2} = k\left( {2\sqrt 2 \times {{10}^{ - 6}}} \right)N/C$ Toward positive y- axis direction
Now ${E_3}$
$ \Rightarrow {E_3} = \dfrac{{k{q_3}}}{{{r^2}}}$
Here $r = \sqrt 2 m$ from diagram
$ \Rightarrow {E_3} = \dfrac{{k \times \sqrt 2 \times {{10}^{ - 6}}}}{{{{\left( {\sqrt 2 } \right)}^2}}}$
$ \Rightarrow {E_3} = \dfrac{{k \times {{10}^{ - 6}}}}{{\sqrt 2 }}N/C$ At ${45^\circ }$ from negative y-axis toward positive x- axis.

Step 2
We assume net electric field due to all three charges is $E$ then
$ \Rightarrow \overrightarrow E = {\overrightarrow E _1} + {\overrightarrow E _2} + {\overrightarrow E _3}$
We make diagram for ${E_1},{E_2}$ and ${E_3}$ with x-y axis


X component for net electric field ${E_x}$
$ \Rightarrow {E_x} = {E_{1x}} + {E_{2x}} + {E_{3x}}$
$ \Rightarrow {E_x} = - \dfrac{{k \times {{10}^{ - 6}}}}{{\sqrt 2 }}\sin 45 + 0 + \dfrac{{k \times {{10}^{ - 6}}}}{{\sqrt 2 }}\sin 45$
Solving this
$\therefore {E_x} = 0$
Y component of net electric field ${E_y}$
$ \Rightarrow {E_y} = {E_{1y}} + {E_{2y}} + {E_{3y}}$
$ \Rightarrow {E_y} = \left( { - \dfrac{{k \times {{10}^{ - 6}}}}{{\sqrt 2 }}\cos 45} \right) + \left( {k\left( {2\sqrt 2 \times {{10}^{ - 6}}} \right)} \right) + \left( { - \dfrac{{k \times {{10}^{ - 6}}}}{{\sqrt 2 }}\cos 45} \right)$
$ \Rightarrow {E_y} = \left( { - \dfrac{{k \times {{10}^{ - 6}}}}{{\sqrt 2 }} \times \dfrac{1}{{\sqrt 2 }}} \right) + \left( {k \times 2\sqrt 2 \times {{10}^{ - 6}}} \right) + \left( { - \dfrac{{k \times {{10}^{ - 6}}}}{{\sqrt 2 }} \times \dfrac{1}{{\sqrt 2 }}} \right)$
Solving this
$ \Rightarrow {E_y} = \left( {k \times {{10}^{ - 6}}} \right)\left( { - \dfrac{1}{2} + 2\sqrt 2 - \dfrac{1}{2}} \right)$
$ \Rightarrow {E_y} = \left( {k \times {{10}^{ - 6}}} \right)\left( {2\sqrt 2 - 1} \right)$
Put the value of constant k
$k = 9 \times {10^9}$
$ \Rightarrow {E_y} = \left( {9 \times {{10}^9} \times {{10}^{ - 6}}} \right)\left( {2\sqrt 2 - 1} \right)$
Solving again
$ \Rightarrow {E_y} = \left( {2\sqrt 2 - 1} \right)\left( {9 \times {{10}^3}} \right)N/C$
So net electric field
$ \Rightarrow E = \sqrt {{{\left( {{E_x}} \right)}^2} + {{\left( {{E_y}} \right)}^2}} $
$ \Rightarrow E = \sqrt {{{\left( 0 \right)}^2} + {{\left[ {\left( {2\sqrt 2 - 1} \right)\left( {9 \times {{10}^3}} \right)} \right]}^2}} $
Hence
$\therefore E = \left( {2\sqrt 2 - 1} \right)\left( {9 \times {{10}^3}} \right)N/C$
So we find net electric field $\left( {2\sqrt 2 - 1} \right) \times 9 \times {10^3}N/C$

Hence option B is correct

Note: In the question we take direction of electric field in positive charge away from the charge and in case of negative charge take toward the charge reason is electric field line
In positive charge electric field lines go away from the positive charge means appears to emit from positive charge and field lines appear to converse at negative charge. So we take the field direction according to this assumption.