Maxwell Distribution of Velocities for JEE

The Maxwell-Boltzmann distribution (also known as the Maxwell distribution) is a statistical representation of the energy of molecules in a classical gas. The distribution of velocities among the molecules of gas was initially proposed by Scottish physicist James Clerk Maxwell in 1859, based on probabilistic reasons. German scientist Ludwig Boltzmann expanded Maxwell's result in 1871 to express the distribution of energy among molecules.

The Maxwell-Boltzmann Distribution of Velocity

Gas is made up of thousands of microscopic particles (atoms or molecules) separated by enormous empty gaps. These particles move in all directions all of the time. They collide with each other as well as the container's walls while in motion. The molecules' speed and directions are constantly changing as a result of these collisions. As a result, the speed of all the molecules in a particular gas sample is not the same. Individual molecule speeds vary and are distributed over a broad range. Even if all of the particles started at the same speed, molecular collisions will cause them to move at different speeds. The speeds of various molecules are also changing. However, at a given temperature, the distribution of speeds among different molecules remains constant, despite changes in individual speeds.

As a result, the fraction of molecules with a specific speed remains constant. As they were the first to formulate it, it is known as the distribution of speeds as well as the Maxwell-Boltzmann distribution law or Maxwell law. Maxwell and Boltzmann plotted the fraction of molecules that move at different speeds (along the y-axis), against the speeds of the molecules (along the x-axis). The resulting curve is known as ‘Maxwell's distribution curve’.

Maxwell Distribution of Velocities

The following are the key characteristics of the Maxwell distribution of velocities:

(i) The fraction of molecules with extremely low or extremely high velocities is extremely small.

(ii) The fraction of molecules with higher velocities increases until it reaches a plateau, at which point it begins to decline.

(iii) The maximum fraction of molecules has a velocity, which corresponds to the curve's peak. This is referred to as the most likely velocity.

Thus, the most likely speed of a gas is the speed of the greatest fraction of gas molecules at a given temperature.

The Effect of Temperature on Speed Distribution

The increase in molecular motion occurs as the temperature of the gas rises. As the temperature rises, so does the value of the most likely speed (up). In fact, as the temperature rises, the entire distribution curve shifts to the right. In other words, as temperatures rise, the curve broadens. It should be noted, however, that if the temperature remains constant, the distribution of speeds among molecules does not change.

The speed distribution at a given temperature is also affected by the mass of the molecules. At the same temperature, heavier gas molecules move slower than lighter gas molecules. Lighter nitrogen molecules, for example, move faster than heavier chlorine molecules. As a result, at any given temperature, nitrogen molecules are more likely to move than chlorine molecules. Image 2 shows the molecular speed distribution curves for chlorine and nitrogen.

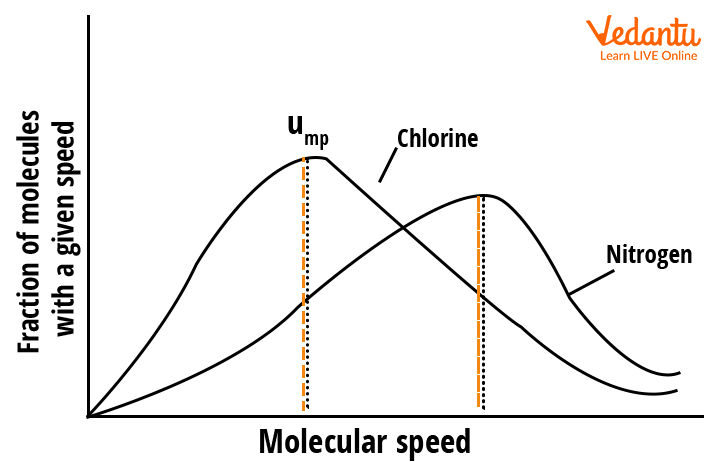
The Molecular Speed Distribution Curves for Chlorine and Nitrogen.

Maxwell Distribution of Molecular Velocities Derivation

The Most Probable Speed

The most probable speed of a gas's molecules is the speed possessed by the greatest fraction of the total number of molecules in the gas.

For example, if the speeds of ten molecules of a gas are 1, 2, 2, 3, 3, 3, 4, 5, 6, 6, km/s, the most likely speed is 3 km/s, because the maximum fraction of total molecules poses this speed, i.e. most probable speed , $C_{mp}=3~\dfrac{km}{s}$ . It should be noted that the subscript maximum refers to the maximum fraction of molecules, not the maximum speed.

It can be shown that,

$C_{m p}=\sqrt{\dfrac{2 k_{B} T}{m}}$

Where m is the molecule's mass, k_B is the Boltzmann constant and T is the gas's temperature. For a given gas, the value of most likely speed v_max decreases as temperature rises.

Mean Speed or Average Speed (Cav)

Average speed is the average speed of a gas molecule. It equals the sum of the individual molecule speeds divided by the number of molecules. If C₁+C₂+C₃+......Cn are the individual molecule speeds, their mean or average speed is 

$C_{a v}=\dfrac{C_{1}+C_{2}+\ldots+C_{n}}{n}$ 

Using Maxwellian speed distribution law, we can show that,

$C_{a v}=\sqrt{\dfrac{8 k_{B} T}{m \pi}}$

Where m is the mass of each molecule, 

kB is the Boltzmann constant and T is the temperature.

Root Mean Square Speed Cmax

The square root of the mean of the squares of the random velocities of individual gas molecules is defined as the root mean square speed of gas molecules. If  C₁,C₂,...,C_n are random velocities of n molecules of a gas,  then the RMS  speed of molecules is:

$C_{r m s}=\sqrt{\dfrac{C_{1}^{2}+C_{2}^{2}+\ldots C_{n}^{2}}{n}}$

We can deduce from the Maxwellian speed distribution law that

$C_{r m s}=\sqrt{\dfrac{{ }^{3 k_{B} T}}{m}}$

where the symbols have their usual meaning.

Relationship between different types of Speeds

The root mean square speed $(\bar{u_{rms}})$, most probable speed, ump and average speed, uav  are given by the expressions: 

The root mean square speed is a common measurement that can be calculated using the following relationships:

$u_{r m s}=\sqrt{\dfrac{3 R T}{M}} \text { or } \sqrt{\dfrac{3 p V}{M}} \text { or } \sqrt{\dfrac{3 p}{\rho}}$

Application of Distribution Law

The Maxwell-Boltzmann equation, which serves as the foundation of gas kinetic theory, defines the distribution of speeds for gas at a given temperature. This distribution function can calculate the most likely speed, average speed, and root-mean-square speed.

The Maxwell–Boltzmann distribution is a probability distribution used in physics and chemistry. The field of statistical mechanics is the most common application. The temperature of any (massive) physical system is determined by the motions of its molecules and atoms.

Conclusion

From the above discussion, we understand the Maxwell distribution and distribution of velocities of various molecules and distribution of molecular velocities derivation and different types of speed. There are some characteristics of Maxwell distribution, like the most likely velocity is the velocity that the maximum fraction of molecules has, which corresponds to the curve's peak. We also learned the effect of temperature and that the distribution of velocities stays constant if the temperature is unchanged. An increase in temperature increased the molecular speed, hence shifting the curve towards the right.