
For maximum power from the battery the internal resistance of the battery $r$ is?
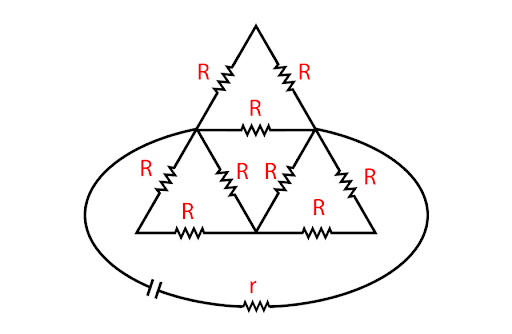
A. 10R
B. 4R/9
C. R/8
D. 10R/9
Answer
172.5k+ views
Hint:The numerical problem is from the current electricity part of physics. We can apply electric power and equivalent resistance concepts to solve the problem.
Formula used:
For maximum power, the equivalent resistance should be equal to internal resistance. That is,
$r = {R_E}$
Where ${R_E}$ = equivalent resistant and r = internal resistance.
Equivalent resistance for a series resistance circuit:
${R_E} = {R_1} + {R_2} + {R_3}$
Where ${R_E}$= equivalent resistance and ${R_1},{R_2},{R_3}$ = component resistance.
Equivalent resistance for a parallel resistance circuit:
$\dfrac{1}{{{R_E}}} = \dfrac{1}{{{R_1}}} + \dfrac{1}{{{R_2}}} + \dfrac{1}{{{R_3}}}$
Where ${R_E}$= equivalent resistance and ${R_1},{R_2},{R_3}$ = component resistance.
Complete step by step solution:
In the triangle part of the circuit, the three sides have two resistances in a series connection. Their equivalent resistance will be ${R_E} = R + R = 2R$. The circuit diagram will be
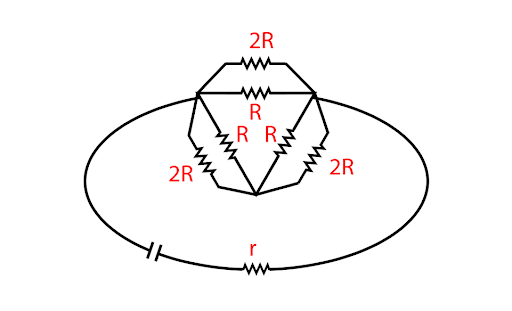
The equivalent resistance for the three parallel combinations in the circuit will become,
$\dfrac{1}{{{R_E}}} = \dfrac{1}{R} + \dfrac{1}{{2R}} = \dfrac{3}{{2R}} \\
\Rightarrow {R_E} = \dfrac{{2R}}{3}$
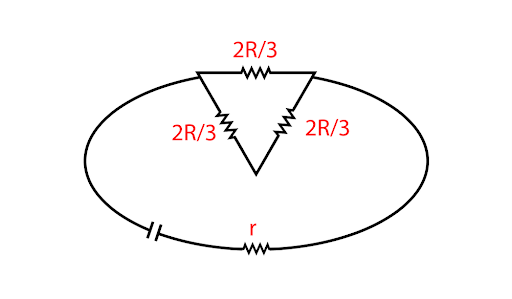
The equivalent resistance for the three-series connection is,
${R_E} = \dfrac{{2R}}{3} + \dfrac{{2R}}{3} = \dfrac{{4R}}{3}$
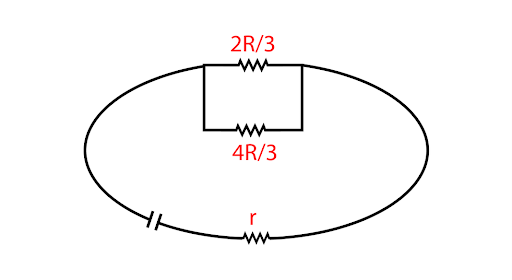
The equivalent resistance for the final parallel connection is,
${R_E} = \dfrac{{\dfrac{{2R}}{3} \times \dfrac{{4R}}{3}}}{{\dfrac{{2R}}{3} + \dfrac{{4R}}{3}}} = \dfrac{{4R}}{9}$
Hence, the correct option is option B.
Additional Information: Resistance blocks the flow of current. The S.I. unit of resistance is ohms. The current decreases as resistance increases. On the other hand, the current increases as the resistance decreases. A conductor's electrical resistance is affected by the following parameters: The conductor's cross-sectional area, the conductor's length, the conductor's material and the conducting material's temperature. Electrical resistance is inversely proportional to the cross-sectional area and directly proportional to the conductor's length.
Note: The rate of electrical energy transfer over an electric circuit per unit of time is measured as electric power. The S.I. unit of power is watt or joule per second. The quantity of electric power is scalar.
Formula used:
For maximum power, the equivalent resistance should be equal to internal resistance. That is,
$r = {R_E}$
Where ${R_E}$ = equivalent resistant and r = internal resistance.
Equivalent resistance for a series resistance circuit:
${R_E} = {R_1} + {R_2} + {R_3}$
Where ${R_E}$= equivalent resistance and ${R_1},{R_2},{R_3}$ = component resistance.
Equivalent resistance for a parallel resistance circuit:
$\dfrac{1}{{{R_E}}} = \dfrac{1}{{{R_1}}} + \dfrac{1}{{{R_2}}} + \dfrac{1}{{{R_3}}}$
Where ${R_E}$= equivalent resistance and ${R_1},{R_2},{R_3}$ = component resistance.
Complete step by step solution:
In the triangle part of the circuit, the three sides have two resistances in a series connection. Their equivalent resistance will be ${R_E} = R + R = 2R$. The circuit diagram will be

The equivalent resistance for the three parallel combinations in the circuit will become,
$\dfrac{1}{{{R_E}}} = \dfrac{1}{R} + \dfrac{1}{{2R}} = \dfrac{3}{{2R}} \\
\Rightarrow {R_E} = \dfrac{{2R}}{3}$

The equivalent resistance for the three-series connection is,
${R_E} = \dfrac{{2R}}{3} + \dfrac{{2R}}{3} = \dfrac{{4R}}{3}$

The equivalent resistance for the final parallel connection is,
${R_E} = \dfrac{{\dfrac{{2R}}{3} \times \dfrac{{4R}}{3}}}{{\dfrac{{2R}}{3} + \dfrac{{4R}}{3}}} = \dfrac{{4R}}{9}$
Hence, the correct option is option B.
Additional Information: Resistance blocks the flow of current. The S.I. unit of resistance is ohms. The current decreases as resistance increases. On the other hand, the current increases as the resistance decreases. A conductor's electrical resistance is affected by the following parameters: The conductor's cross-sectional area, the conductor's length, the conductor's material and the conducting material's temperature. Electrical resistance is inversely proportional to the cross-sectional area and directly proportional to the conductor's length.
Note: The rate of electrical energy transfer over an electric circuit per unit of time is measured as electric power. The S.I. unit of power is watt or joule per second. The quantity of electric power is scalar.
Recently Updated Pages
Sets, Relations, and Functions Mock Test 2025-26

Faraday's Law - Formula & Example

Molarity vs Molality: Definitions, Formulas & Key Differences

Preparation of Hydrogen Gas: Methods & Uses Explained

Polymers in Chemistry: Definition, Types, Examples & Uses

P Block Elements: Definition, Groups, Trends & Properties for JEE/NEET

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

Displacement-Time Graph and Velocity-Time Graph for JEE

Uniform Acceleration

Electric field due to uniformly charged sphere class 12 physics JEE_Main

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Atomic Structure - Electrons, Protons, Neutrons and Atomic Models

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Enthalpy of Combustion with Examples for JEE

Instantaneous Velocity - Formula based Examples for JEE

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

Important Derivations for CBSE Class 12 Physics (Stepwise Solutions & PDF)




