
What is the area under the curve $y=|x|+|x-1|$ between$x=0$ and $x=1$.
A. $\dfrac{1}{2}$
B. $1$
C. $\dfrac{3}{2}$
D. $2$
Answer
201.3k+ views
Hint: To find the area under the curve $y=|x|+|x-1|$ between$x=0$ and $x=1$,we will write the value of $x$ in the given interval and determine the equation of the curve.
Then we will integrate it using limits by taking lower limit $x=0$ and upper limit $x=1$and determine the area.
Formula Used:The limits of integration of a function can be calculated by $\begin{align}
& \int\limits_{b}^{a}{f(x).dx}=\left[ f(x) \right]_{b}^{a} \\
& =f(a)-f(b)
\end{align}$
Complete step by step solution:We are given an equation of curve$y=|x|+|x-1|$ and we have to find the area enclosed by it in between the interval $x=0$ and $x=1$.
For the interval\[x\ge 0\],
$|x|=x$
And for the interval \[x\le 1\],
$|x-1|=-(x-1)$
The equation will be $y=x-(x-1)$
Now we will integrate it taking $x=0$as lower limit and $x=1$as upper limit.
\[Area=\int\limits_{0}^{1}{x.dx}-\int\limits_{0}^{1}{(x-1)dx}\]
\[=\left[ \dfrac{{{x}^{2}}}{2} \right]_{0}^{1}-\left[ \dfrac{{{x}^{2}}}{2}-x \right]_{0}^{1}\]
Applying the limits,
\[=\left[ \dfrac{1}{2}-\dfrac{0}{2} \right]-\left[ \left( \dfrac{1}{2}-1 \right)-\left( \dfrac{0}{2}-0 \right) \right]\]
\[=\dfrac{1}{2}-\left( \dfrac{1}{2}-1 \right)\]
\[=1\]square unit.
The area under the curve $y=|x|+|x-1|$ between $x=0$ and $x=1$ is $1$ square units.
Thus, the area under the curve $y=|x|+|x-1|$ between$x=0$ and $x=1$
Option ‘D’ is correct
Note:We can also find the area under the curve by another method. In this method we will simplify the equation of the curve first.
The equation of the curve is .
Now we will draw the graph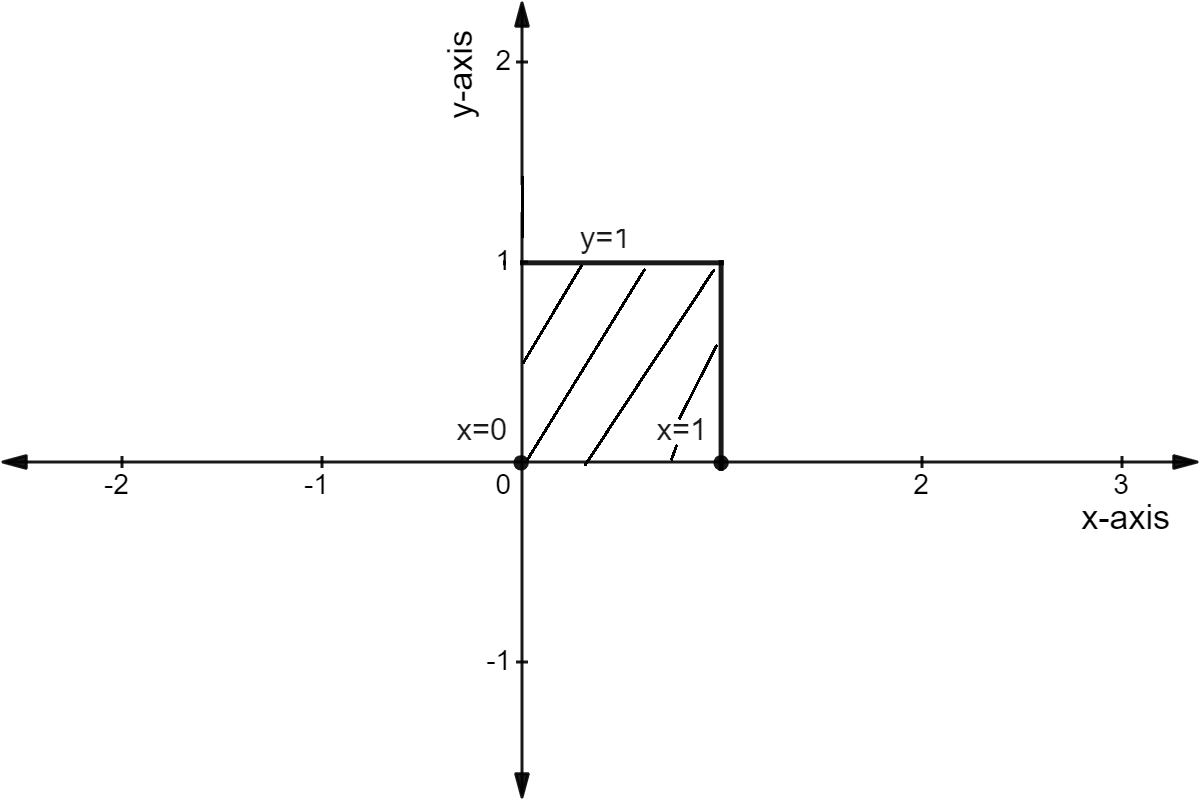
We can see that a quadrilateral is formed on the graph of the curve between the interval l and . The quadrilateral formed is a square because each of the side is of length of unit .
We will calculate the area under the curve by finding the area of the square. As we know the formula of the area of the square is , we will use it.
1 square units.
.
Then we will integrate it using limits by taking lower limit $x=0$ and upper limit $x=1$and determine the area.
Formula Used:The limits of integration of a function can be calculated by $\begin{align}
& \int\limits_{b}^{a}{f(x).dx}=\left[ f(x) \right]_{b}^{a} \\
& =f(a)-f(b)
\end{align}$
Complete step by step solution:We are given an equation of curve$y=|x|+|x-1|$ and we have to find the area enclosed by it in between the interval $x=0$ and $x=1$.
For the interval\[x\ge 0\],
$|x|=x$
And for the interval \[x\le 1\],
$|x-1|=-(x-1)$
The equation will be $y=x-(x-1)$
Now we will integrate it taking $x=0$as lower limit and $x=1$as upper limit.
\[Area=\int\limits_{0}^{1}{x.dx}-\int\limits_{0}^{1}{(x-1)dx}\]
\[=\left[ \dfrac{{{x}^{2}}}{2} \right]_{0}^{1}-\left[ \dfrac{{{x}^{2}}}{2}-x \right]_{0}^{1}\]
Applying the limits,
\[=\left[ \dfrac{1}{2}-\dfrac{0}{2} \right]-\left[ \left( \dfrac{1}{2}-1 \right)-\left( \dfrac{0}{2}-0 \right) \right]\]
\[=\dfrac{1}{2}-\left( \dfrac{1}{2}-1 \right)\]
\[=1\]square unit.
The area under the curve $y=|x|+|x-1|$ between $x=0$ and $x=1$ is $1$ square units.
Thus, the area under the curve $y=|x|+|x-1|$ between$x=0$ and $x=1$
Option ‘D’ is correct
Note:We can also find the area under the curve by another method. In this method we will simplify the equation of the curve first.
The equation of the curve is .
Now we will draw the graph

We can see that a quadrilateral is formed on the graph of the curve between the interval l and . The quadrilateral formed is a square because each of the side is of length of unit .
We will calculate the area under the curve by finding the area of the square. As we know the formula of the area of the square is , we will use it.
1 square units.
.
Recently Updated Pages
JEE Differential Calculus Important Concepts and Tips for Exam Preparation

Sum to Product Formulae - Important Concepts and Tips for JEE

Chemical Equation - Important Concepts and Tips for JEE

Slip Ring – Definition, Composition, Types, Uses and FAQs

Associative Property Formula - Addition, Multiplication and Examples

Dimensions of Impedance – Formula, Derivation and FAQs

Trending doubts
JEE Main 2026: Exam Date, Syllabus, Eligibility, Application Form & Preparation Tips

JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

JEE Main Syllabus 2026 (Updated)

Newton’s Laws of Motion Explained: Concepts, Formulas & Uses

JEE Main Previous Year Question Paper with Answer Keys and Solutions

JEE Main 2026 Session 2 Registration – Apply Now! Form Link, Last Date and Fees

Other Pages
NCERT Solutions for Class 10 Maths Chapter 11 Areas Related to Circles 2025-26

NCERT Solutions For Class 10 Maths Chapter 12 Surface Area And Volume

NCERT Solutions for Class 10 Maths Chapter Chapter 13 Statistics

All Mensuration Formulas with Examples and Quick Revision

NCERT Solutions for Class 10 Maths Chapter 15 Probability

Pregnancy Week and Due Date Calculator: Find How Far Along You Are




