
A plane mirror is placed on level ground at a distance $60m$ from the foot of a tower, the ray coming from the top of the tower and its reflected ray from the mirror subtends an angle $90^\circ $. What is the height of the tower?
A) $30m$
B) $60m$
C) $90m$
D) $120m$
Answer
173.1k+ views
Hint:When a plane mirror reflects a ray of light, the angle of reflection is always equal to the angle of incidence. The ray of incidence, reflection and the normal all lie on the same plane. This principle will help us solve the problem in hand. If an incident ray subtends a certain angle then this means that that angle represents the sum of both reflected angle and incident angle.
Complete step by step solution:
Let’s first analyse the scenario. The mirror is at a distance of $60m$ from the foot of the tower.
The incident ray from the top of the tower will hit the mirror to subtend an angle of $90^\circ $. This means that when the incident ray, coming from the tip of the tower hits the mirror it creates a $45^\circ $ angle with the normal and the angle of reflection is $45^\circ $. This is because reflection is based on the principle that angle of reflection is equal to the angle of incidence.
Let’s look at the following figure for better understanding.
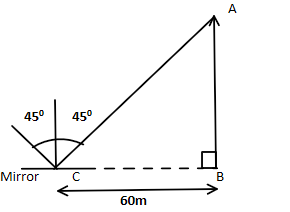
From the figure we can see that in the right angled triangle $\vartriangle ABC$ the value of angle $\angle C$ is $45^\circ $.
This implies that the right-angled triangle is an isosceles triangle too. So the lengths of arms $\overline {AB} $ and $\overline {BC} $ are equal.
So the height of the tower will be $\left| {\overline {AB} } \right| = \left| {\overline {BC} } \right| = 60m$
Thus our correct answer is option (B).
Note:Any kind of reflecting surface works on the principle that angle of incidence is equal to angle of reflection. In some cases we might need to use trigonometric calculations in order to find an angle or length of an arm.
Complete step by step solution:
Let’s first analyse the scenario. The mirror is at a distance of $60m$ from the foot of the tower.
The incident ray from the top of the tower will hit the mirror to subtend an angle of $90^\circ $. This means that when the incident ray, coming from the tip of the tower hits the mirror it creates a $45^\circ $ angle with the normal and the angle of reflection is $45^\circ $. This is because reflection is based on the principle that angle of reflection is equal to the angle of incidence.
Let’s look at the following figure for better understanding.

From the figure we can see that in the right angled triangle $\vartriangle ABC$ the value of angle $\angle C$ is $45^\circ $.
This implies that the right-angled triangle is an isosceles triangle too. So the lengths of arms $\overline {AB} $ and $\overline {BC} $ are equal.
So the height of the tower will be $\left| {\overline {AB} } \right| = \left| {\overline {BC} } \right| = 60m$
Thus our correct answer is option (B).
Note:Any kind of reflecting surface works on the principle that angle of incidence is equal to angle of reflection. In some cases we might need to use trigonometric calculations in order to find an angle or length of an arm.
Recently Updated Pages
Sets, Relations, and Functions Mock Test 2025-26

Molarity vs Molality: Definitions, Formulas & Key Differences

Preparation of Hydrogen Gas: Methods & Uses Explained

Polymers in Chemistry: Definition, Types, Examples & Uses

P Block Elements: Definition, Groups, Trends & Properties for JEE/NEET

Order of Reaction in Chemistry: Definition, Formula & Examples

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

Displacement-Time Graph and Velocity-Time Graph for JEE

Uniform Acceleration

Electric field due to uniformly charged sphere class 12 physics JEE_Main

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Atomic Structure - Electrons, Protons, Neutrons and Atomic Models

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

Enthalpy of Combustion with Examples for JEE

Instantaneous Velocity - Formula based Examples for JEE

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

Important Derivations for CBSE Class 12 Physics (Stepwise Solutions & PDF)




