
A convex lens and convex mirror are placed co axially and separated by distance d. The focal length of both is \[20\,\,cm\] each. A point object is placed at a distance \[30\,\,cm\] from the lens as shown. Then the value of d so that image on the object itself is
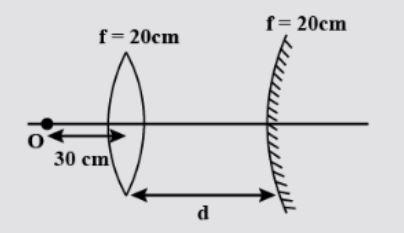
A) $10\,\,cm$
B) \[60\,\,cm\]
C) \[30\,\,cm\]
D) \[20\,\,cm\]
Answer
175.2k+ views
Hint:- The above problem can be solved using the formula derived from the object image and focal distance relationship formulas of the convex lens and the convex mirror of the same focal length. The formula for the focal length of the lens and the mirror is used.
Useful formula:
The distance of the value $d$;
\[\dfrac{1}{d} = \dfrac{1}{f}\]
Where, the $d$ is the distance between the convex lens and convex mirror, $f$ is the focal length of the convex mirror.
Complete step by step solution:
The data given in the problem is;
Focal length of the convex lens is, ${f_1} = 20\,\,cm$.
Focal length of the convex mirror is, ${f_2} = 20\,\,cm$
Distance of the image placed from the image is, ${u_1} = 30\,\,cm$
At convex lens;
$\dfrac{1}{{{f_1}}} = \dfrac{1}{{{v_1}}} + \dfrac{1}{{{u_1}}}$
Substituting the value of focal length and the object distance from the lens
$
\dfrac{1}{{20}} = \dfrac{1}{{{d_i}}} + \dfrac{1}{{30}} \\
\dfrac{1}{{20}} - \dfrac{1}{{30}} = \dfrac{1}{{{d_i}}} \\
$
Where, ${d_i}$ denotes the distance of the image at convex lens.
\[\dfrac{1}{{{d_i}}} = \dfrac{1}{{60}}\]
At convex mirror:
$\dfrac{1}{{{f_2}}} = \dfrac{1}{{{d_i}}} + \dfrac{1}{{{u_o}}}$
Substitutes the values of the focal length and the image distance;
$
\dfrac{1}{{20}} = \dfrac{1}{{60}} + \dfrac{1}{{{d_o}}} \\
\dfrac{1}{{20}} - \dfrac{1}{{60}} = \dfrac{1}{{{d_o}}} \\
$
Where, ${d_o}$ denotes the distance of the object at convex mirror.
\[\dfrac{1}{{{d_o}}} = \dfrac{1}{{30}}\]
The distance of the value $d$;
\[\dfrac{1}{d} = \dfrac{1}{f}\]
That is
\[
\dfrac{1}{d} = \dfrac{1}{f} = \dfrac{1}{{{d_i}}} + \dfrac{1}{{{d_o}}} \\
\dfrac{1}{d} = \dfrac{1}{{60}} + \dfrac{1}{{30}} \\
\dfrac{1}{d} = \dfrac{{90}}{{1800}} \\
d = 20\,\,cm \\
\]
Therefore, the value of the $d$ is 20 cm.
Hence the option (D), \[d = 20\,\,cm\] is the correct answer.
Note: Image distance denotes that when the image is created then the distance between pole and image is known image distance. Focal length is the interval between pole and the principal focus of the mirror.
Useful formula:
The distance of the value $d$;
\[\dfrac{1}{d} = \dfrac{1}{f}\]
Where, the $d$ is the distance between the convex lens and convex mirror, $f$ is the focal length of the convex mirror.
Complete step by step solution:
The data given in the problem is;
Focal length of the convex lens is, ${f_1} = 20\,\,cm$.
Focal length of the convex mirror is, ${f_2} = 20\,\,cm$
Distance of the image placed from the image is, ${u_1} = 30\,\,cm$
At convex lens;
$\dfrac{1}{{{f_1}}} = \dfrac{1}{{{v_1}}} + \dfrac{1}{{{u_1}}}$
Substituting the value of focal length and the object distance from the lens
$
\dfrac{1}{{20}} = \dfrac{1}{{{d_i}}} + \dfrac{1}{{30}} \\
\dfrac{1}{{20}} - \dfrac{1}{{30}} = \dfrac{1}{{{d_i}}} \\
$
Where, ${d_i}$ denotes the distance of the image at convex lens.
\[\dfrac{1}{{{d_i}}} = \dfrac{1}{{60}}\]
At convex mirror:
$\dfrac{1}{{{f_2}}} = \dfrac{1}{{{d_i}}} + \dfrac{1}{{{u_o}}}$
Substitutes the values of the focal length and the image distance;
$
\dfrac{1}{{20}} = \dfrac{1}{{60}} + \dfrac{1}{{{d_o}}} \\
\dfrac{1}{{20}} - \dfrac{1}{{60}} = \dfrac{1}{{{d_o}}} \\
$
Where, ${d_o}$ denotes the distance of the object at convex mirror.
\[\dfrac{1}{{{d_o}}} = \dfrac{1}{{30}}\]
The distance of the value $d$;
\[\dfrac{1}{d} = \dfrac{1}{f}\]
That is
\[
\dfrac{1}{d} = \dfrac{1}{f} = \dfrac{1}{{{d_i}}} + \dfrac{1}{{{d_o}}} \\
\dfrac{1}{d} = \dfrac{1}{{60}} + \dfrac{1}{{30}} \\
\dfrac{1}{d} = \dfrac{{90}}{{1800}} \\
d = 20\,\,cm \\
\]
Therefore, the value of the $d$ is 20 cm.
Hence the option (D), \[d = 20\,\,cm\] is the correct answer.
Note: Image distance denotes that when the image is created then the distance between pole and image is known image distance. Focal length is the interval between pole and the principal focus of the mirror.
Recently Updated Pages
JEE Main 2025-26 Atoms and Nuclei Mock Test: Free Practice Online

JEE Main 2025-26: Dual Nature of Matter and Radiation Mock Test

JEE Main 2025-26 Electronic Devices Mock Test – Free Practice

JEE Main Mock Test 2025-26: Experimental Skills Chapter Online Practice

JEE Main 2025-26 Current Electricity Mock Test: Free Practice Online

JEE Main 2025-26 Rotational Motion Mock Test – Free Practice Online

Trending doubts
JEE Main 2025 Session 2: Application Form (Out), Exam Dates (Released), Eligibility, & More

Displacement-Time Graph and Velocity-Time Graph for JEE

Uniform Acceleration

Electric field due to uniformly charged sphere class 12 physics JEE_Main

JEE Main 2025: Derivation of Equation of Trajectory in Physics

Atomic Structure - Electrons, Protons, Neutrons and Atomic Models

Other Pages
JEE Advanced Marks vs Ranks 2025: Understanding Category-wise Qualifying Marks and Previous Year Cut-offs

Learn About Angle Of Deviation In Prism: JEE Main Physics 2025

What is Hybridisation in Chemistry?

JEE Advanced Weightage 2025 Chapter-Wise for Physics, Maths and Chemistry

Essential Derivations for CBSE Class 12 Physics: Stepwise & PDF Solutions

Wheatstone Bridge for JEE Main Physics 2025




