
Answer
503.1k+ views
Hint: Here, we will be proceeding by drawing a diagram using the given data (radius and slant height of the cone) and determine the curved surface area of this cone with the help of formula for the curved surface area of the cone i.e., ${\text{C}}{\text{.S}}{\text{.A}} = \pi rl$.
Complete step-by-step answer:
Given, Radius of the cone r = 7 cm
Slant height of the cone l = 10 cm
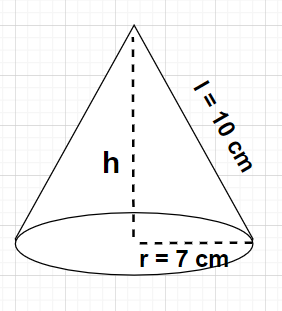
As we know that the formula for the curved surface area of the cone with radius r and slant height l is given by
${\text{C}}{\text{.S}}{\text{.A}} = \pi rl$
Using the above formula, the curved surface area of the given cone is given by
${\text{C}}{\text{.S}}{\text{.A}} = \pi rl = \dfrac{{22}}{7} \times 7 \times 10 = 220{\text{ c}}{{\text{m}}^2}$
Hence, the curved surface area of the cone with a radius of 7 cm and slant height of 10 cm respectively is 220 sq. cm.
Note: In any cone with radius r and height h, the slant height h is given by $l = \sqrt {{r^2} + {h^2}} $. For calculation of curved surface area of the cone (${\text{C}}{\text{.S}}{\text{.A}} = \pi rl$), radius and slant height of the cone is required. But if instead of slant height l, height of the cone h is given then we will be using formula $l = \sqrt {{r^2} + {h^2}} $ to find the value of slant height first.
Complete step-by-step answer:
Given, Radius of the cone r = 7 cm
Slant height of the cone l = 10 cm

As we know that the formula for the curved surface area of the cone with radius r and slant height l is given by
${\text{C}}{\text{.S}}{\text{.A}} = \pi rl$
Using the above formula, the curved surface area of the given cone is given by
${\text{C}}{\text{.S}}{\text{.A}} = \pi rl = \dfrac{{22}}{7} \times 7 \times 10 = 220{\text{ c}}{{\text{m}}^2}$
Hence, the curved surface area of the cone with a radius of 7 cm and slant height of 10 cm respectively is 220 sq. cm.
Note: In any cone with radius r and height h, the slant height h is given by $l = \sqrt {{r^2} + {h^2}} $. For calculation of curved surface area of the cone (${\text{C}}{\text{.S}}{\text{.A}} = \pi rl$), radius and slant height of the cone is required. But if instead of slant height l, height of the cone h is given then we will be using formula $l = \sqrt {{r^2} + {h^2}} $ to find the value of slant height first.
Recently Updated Pages
Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Master Class 9 Maths: Engaging Questions & Answers for Success

Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Trending doubts
Fill the blanks with the suitable prepositions 1 The class 9 english CBSE

How do you graph the function fx 4x class 9 maths CBSE

Distinguish between the following Ferrous and nonferrous class 9 social science CBSE

What is pollution? How many types of pollution? Define it

Voters list is known as A Ticket B Nomination form class 9 social science CBSE

Distinguish between Conventional and nonconventional class 9 social science CBSE




