
How do you find the amplitude, period, and phase shift of \[y=3\sin x\] ?
Answer
490.2k+ views
Hint: These types of trigonometric problems are pretty easy to solve and they are very straight forward. To find the amplitude, period and other things we first need to define the general equation of \[\sin x\] , which is defined as, \[y=A\sin \left( bx+c \right)+d\] . From this general equation we can find out all our required outputs accordingly.
Complete step by step answer: Now we start off our given solution, by writing the general form of the equation, \[y=A\sin \left( bx+c \right)+d\] , and from this, each and every variable represents some of the output values, which affect the nature of the graph of \[y=3\sin x\]. In the defined equation,
\[A\], represents, the amplitude,
From \[y=A\sin \left( bx+c \right)+d\] we can find the period of the function by using the formula of period of \[\sin x\] that \[period=\dfrac{\left| 2\pi \right|}{b}\]
\[c\] , represents the horizontal phase shift or phase shift and,
\[d\] , represents the vertical phase shift.
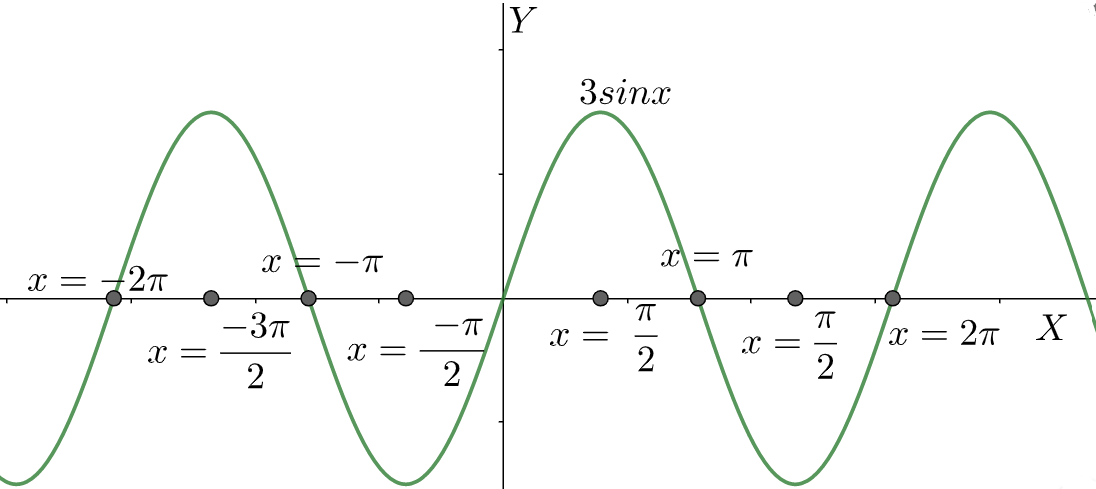
Now, writing our given equation of the form of the general defined one we get,
\[y=3\sin \left( 1.x+0 \right)+0\],
From this equation, we can write, that the Amplitude of the function is defined as,
\[A=3\]
We can also conclude that the value of the period of the function is defined as,
\[\begin{align}
& period=\dfrac{\left| 2\pi \right|}{1} \\
& \Rightarrow period=2\pi \\
\end{align}\]
We can conclude that the value of the phase shift or the horizontal shift of the function is given by,
\[c=0\] .
Note: While solving these types of problems, we must be very careful in representing the general form of the equation properly. Once the general form is represented correctly, we can find all the necessary things by comparing the given equation with the general form. We also need to remember all the variables and parameters, and what variables represent what.
Complete step by step answer: Now we start off our given solution, by writing the general form of the equation, \[y=A\sin \left( bx+c \right)+d\] , and from this, each and every variable represents some of the output values, which affect the nature of the graph of \[y=3\sin x\]. In the defined equation,
\[A\], represents, the amplitude,
From \[y=A\sin \left( bx+c \right)+d\] we can find the period of the function by using the formula of period of \[\sin x\] that \[period=\dfrac{\left| 2\pi \right|}{b}\]
\[c\] , represents the horizontal phase shift or phase shift and,
\[d\] , represents the vertical phase shift.

Now, writing our given equation of the form of the general defined one we get,
\[y=3\sin \left( 1.x+0 \right)+0\],
From this equation, we can write, that the Amplitude of the function is defined as,
\[A=3\]
We can also conclude that the value of the period of the function is defined as,
\[\begin{align}
& period=\dfrac{\left| 2\pi \right|}{1} \\
& \Rightarrow period=2\pi \\
\end{align}\]
We can conclude that the value of the phase shift or the horizontal shift of the function is given by,
\[c=0\] .
Note: While solving these types of problems, we must be very careful in representing the general form of the equation properly. Once the general form is represented correctly, we can find all the necessary things by comparing the given equation with the general form. We also need to remember all the variables and parameters, and what variables represent what.
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Master Class 11 Physics: Engaging Questions & Answers for Success

Trending doubts
1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

At which age domestication of animals started A Neolithic class 11 social science CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

Whales are warmblooded animals which live in cold seas class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

How much is 23 kg in pounds class 11 chemistry CBSE




