
Explain how you will convert a galvanometer into voltmeter.
Answer
463.1k+ views
Hint: First we know about galvanometer and voltmeter. A galvanometer is a device which is used to detect small electric current flowing in the circuit and Voltmeter is an instrument used to measure the potential difference across the two ends of a circuit element.
Complete step by step answer:
A galvanometer can be converted into a voltmeter by connecting a large resistance in series to the galvanometer which is shown in diagram.
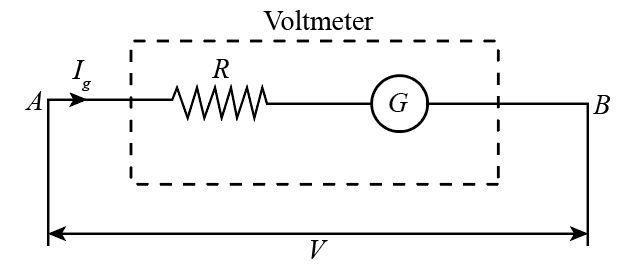
Let G and R be the resistance of a galvanometer and a conductor connected in series with it respectively.
Let V volt be the potential difference to be measured by the voltmeter and Ig, be the current.
Now potential difference between point A and B :
$\eqalign{
& V = {I_g}R + {I_g}G = {I_g}(R + G) \cr
& \cr
& \therefore R + G = \dfrac{V}{{{I_g}}} \cr} $
$R = \dfrac{V}{{{I_g}}} - G$
This is the required value of resistance which must be connected in series to the galvanometer to convert it into a voltmeter of range 0-V volt.
Additional information: Voltmeter is a high resistance device. Resistance of an ideal voltmeter is infinite. A voltmeter is always connected in parallel to the circuit component across which voltage is to be measured.
Note: If we connect a simple galvanometer in parallel to the circuit, it will draw some current and hence the potential difference of the circuit will change and the measurement will not be accurate. For accurate measurement of the potential difference, it is essential that current between the two points should remain the same after connecting a measuring device. This is possible if the resistance of the device is infinite.
Complete step by step answer:
A galvanometer can be converted into a voltmeter by connecting a large resistance in series to the galvanometer which is shown in diagram.

Let G and R be the resistance of a galvanometer and a conductor connected in series with it respectively.
Let V volt be the potential difference to be measured by the voltmeter and Ig, be the current.
Now potential difference between point A and B :
$\eqalign{
& V = {I_g}R + {I_g}G = {I_g}(R + G) \cr
& \cr
& \therefore R + G = \dfrac{V}{{{I_g}}} \cr} $
$R = \dfrac{V}{{{I_g}}} - G$
This is the required value of resistance which must be connected in series to the galvanometer to convert it into a voltmeter of range 0-V volt.
Additional information: Voltmeter is a high resistance device. Resistance of an ideal voltmeter is infinite. A voltmeter is always connected in parallel to the circuit component across which voltage is to be measured.
Note: If we connect a simple galvanometer in parallel to the circuit, it will draw some current and hence the potential difference of the circuit will change and the measurement will not be accurate. For accurate measurement of the potential difference, it is essential that current between the two points should remain the same after connecting a measuring device. This is possible if the resistance of the device is infinite.
Recently Updated Pages
NCERT Solutions For Class 4 English Marigold (Poem) - Don’t Be Afraid Of The Dark

NCERT Solutions For Class 5 English Marigold (Poem) - Class Discussion

NCERT Solutions For Class 5 English Marigold - Gullivers Travels

NCERT Solutions For Class 5 Hindi Rimjhim - Bagh Aaya Uss Raat

NCERT Solutions For Class 8 Hindi Bharat Ki Khoj - Tanaav

NCERT Solutions For Class 12 Maths - Differential Equations

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?

How many single covalent bonds can nitrogen form class 12 chemistry CBSE

The idea of the Constitution of India was first of class 12 social science CBSE

Draw a velocitytime graph for a uniform accelerated class 12 physics CBSE

What is the feedback mechanism of hormone regulation class 12 biology CBSE




