
Draw a neat and labeled energy level diagram and explain the Balmer series and Brackett series of spectral lines for hydrogen atoms
The work function for a metal surface is 2.2 eV. If light of wavelength $5000\overset {\circ} {\mathop{A}}\, $ is incident on the surface of the metal, find the threshold frequency and incident frequency. Will there be an emission of photoelectrons or not? ($c=3\times {{10}^{8}}\dfrac{m}{s},1eV=1.6\times {{10}^{-19}},h=6.63\times {{10}^{-34}}J.s.$ ).
Answer
470.7k+ views
Hint: We will draw the energy level diagram of the hydrogen atom and locate different energy levels in it. From this respective diagram we are going to explain the blamer and brackett series of spectral lines. In the numerical we are going to find threshold frequency and for this we need to find work function and then, incident frequency.
Complete step-by-step answer:
First part: In the energy level of hydrogen atom, there are 5 energy states:
1) Lyman ; 2) Balmer ; 3) Paschen ; 4) Brackett ; 5) Pfund
These all energy states have different energies.
The figure given below is the energy level diagram of hydrogen atom:
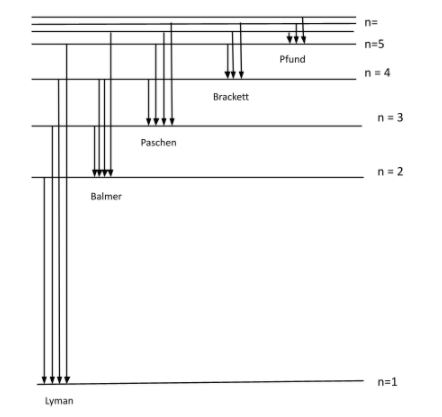
The radiation associated with the transition can be categorized as, ultraviolet, visible and invisible spectrum of radiation.
We can see in the figure above and say that:
Balmer series: It is a series of spectral lines of energy where transition occurs from ${{n}_{i}}=2$ to ${{n}_{0}}=3,4,5,.......$
Its expression is written as –
$\dfrac{1}{\lambda }={{R}_{H}}\left[ \dfrac{1}{{{2}^{2}}}-\dfrac{1}{{{n}_{0}}^{2}} \right]$ ; ${{R}_{H}}$ =Rydberg’s constant
Brackett series: It is a series of spectral lines of energy where transition occurs from ${{n}_{i}}=4$ to ${{n}_{0}}=5,6,7,....$
Its expression is written as –
$\dfrac {1} {\lambda} = {{R} _ {H}}\left [ \dfrac{1}{{{4}^{2}}}-\dfrac{1}{{{n}_{0}}^{2}} \right]$
Second part:
We have already been provided that –
$\begin {align}
& work\, function= {{\phi} _ {0}} =2.2\, eV \\
& =2.2\times 1.6\times {{10} ^ {-19}} J \\
& =3.52\times {{10} ^ {-19}} J \\
\end{align}$
Now, as mentioned in question the values –
$\lambda =5000\overset {\circ} {\mathop{A}}\, $,
$\begin {align}
& c=3\times {{10} ^ {8}}\dfrac {m} {s}, \\
& h=6.63\times {{10} ^ {-34}} J.s. \\
\end{align}$
So, we will apply the formula of incident frequency (v) is –
$\therefore incident\, frequency (v) =\dfrac{c} {\lambda} $
$\begin {align}
& =\dfrac {3\times {{10} ^ {8}}} {5\times {{10} ^ {-7}}} \\
& =\dfrac {3} {5}\times {{10} ^ {15}}\, Hz \\
& =\dfrac {30} {5}\times {{10} ^ {14\,}}\, Hz \\
& =6\times {{10} ^ {14}}\, Hz \\
\end{align}$
And, the formula for threshold frequency (v0) is, ${{v} _ {0}} =\dfrac {{{\phi} _ {0}}} {h} $
$\begin {align}
& \therefore {{v}_{0}}=\dfrac{3.52\times {{10} ^ {-19}} J} {6.63\times {{10} ^ {-34}} Js} \\
& \,\,\,\,\,\,\,\,\,=0.53\times {{10} ^ {15}} Hz \\
& \,\,\,\,\,\,\,\,\,=5.3\times {{10} ^ {14}} Hz \\
\end{align}$
Yes, there will be emission of photoelectrons as incident frequency is greater than threshold frequency.
Note: The potential energy at $n=\infty $is zero as nucleus does not attracts electron so, electrons are free moving and hence, are unstable whereas, in lowest energy state $n=1$, the electrons are tightly bonded, as there is more attractive force between nucleus and electron, and are highly stable and also have negative potential energy.
Complete step-by-step answer:
First part: In the energy level of hydrogen atom, there are 5 energy states:
1) Lyman ; 2) Balmer ; 3) Paschen ; 4) Brackett ; 5) Pfund
These all energy states have different energies.
The figure given below is the energy level diagram of hydrogen atom:

The radiation associated with the transition can be categorized as, ultraviolet, visible and invisible spectrum of radiation.
We can see in the figure above and say that:
Balmer series: It is a series of spectral lines of energy where transition occurs from ${{n}_{i}}=2$ to ${{n}_{0}}=3,4,5,.......$
Its expression is written as –
$\dfrac{1}{\lambda }={{R}_{H}}\left[ \dfrac{1}{{{2}^{2}}}-\dfrac{1}{{{n}_{0}}^{2}} \right]$ ; ${{R}_{H}}$ =Rydberg’s constant
Brackett series: It is a series of spectral lines of energy where transition occurs from ${{n}_{i}}=4$ to ${{n}_{0}}=5,6,7,....$
Its expression is written as –
$\dfrac {1} {\lambda} = {{R} _ {H}}\left [ \dfrac{1}{{{4}^{2}}}-\dfrac{1}{{{n}_{0}}^{2}} \right]$
Second part:
We have already been provided that –
$\begin {align}
& work\, function= {{\phi} _ {0}} =2.2\, eV \\
& =2.2\times 1.6\times {{10} ^ {-19}} J \\
& =3.52\times {{10} ^ {-19}} J \\
\end{align}$
Now, as mentioned in question the values –
$\lambda =5000\overset {\circ} {\mathop{A}}\, $,
$\begin {align}
& c=3\times {{10} ^ {8}}\dfrac {m} {s}, \\
& h=6.63\times {{10} ^ {-34}} J.s. \\
\end{align}$
So, we will apply the formula of incident frequency (v) is –
$\therefore incident\, frequency (v) =\dfrac{c} {\lambda} $
$\begin {align}
& =\dfrac {3\times {{10} ^ {8}}} {5\times {{10} ^ {-7}}} \\
& =\dfrac {3} {5}\times {{10} ^ {15}}\, Hz \\
& =\dfrac {30} {5}\times {{10} ^ {14\,}}\, Hz \\
& =6\times {{10} ^ {14}}\, Hz \\
\end{align}$
And, the formula for threshold frequency (v0) is, ${{v} _ {0}} =\dfrac {{{\phi} _ {0}}} {h} $
$\begin {align}
& \therefore {{v}_{0}}=\dfrac{3.52\times {{10} ^ {-19}} J} {6.63\times {{10} ^ {-34}} Js} \\
& \,\,\,\,\,\,\,\,\,=0.53\times {{10} ^ {15}} Hz \\
& \,\,\,\,\,\,\,\,\,=5.3\times {{10} ^ {14}} Hz \\
\end{align}$
Yes, there will be emission of photoelectrons as incident frequency is greater than threshold frequency.
Note: The potential energy at $n=\infty $is zero as nucleus does not attracts electron so, electrons are free moving and hence, are unstable whereas, in lowest energy state $n=1$, the electrons are tightly bonded, as there is more attractive force between nucleus and electron, and are highly stable and also have negative potential energy.
Recently Updated Pages
Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Who discovered the cell and how class 12 biology CBSE

Draw a labelled sketch of the human eye class 12 physics CBSE




