
Derive mirror equation. State any three experimental observations of photoelectric emission.
Answer
454.5k+ views
Hint: In a spherical mirror, the distance of the object from its pole is called the object distance (u). The distance of the image from the pole of the mirror is called the image distance (v). The distance of the principal focus from the pole is called the focal length (f). There is a relationship between these three quantities given by the mirror formula which is expressed as $\dfrac{1}{v} + \dfrac{1}{u} = \dfrac{1}{f}$.This formula is valid in all situations for all spherical mirrors for all positions of the object.
Complete step by step solution:
Derivation of the Mirror Formula
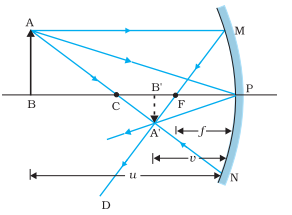
We now derive the mirror equation or the relation between the object distance (u), image distance (v) and the focal length (f). In the above figure, the two right-angled triangles A′B′F and MPF are similar.
$
\Rightarrow \dfrac{{{B'}{A'}}}{{PM}} = \dfrac{{{B'}F}}{{FP}} \\
\Rightarrow \dfrac{{{B'}{A'}}}{{BA}} = \dfrac{{{B'}F}}{{FP}} \\
\because PM = AB...............(1) \\
\Rightarrow \angle APB = \angle {A'}PB \\
\therefore \Delta {A'}{B'}{P'} \sim \Delta A{B'}P \\
\therefore \dfrac{{{B'}{A'}}}{{BA}} = \dfrac{{{B'}P}}{{BP}}............(2) \\
By{\text{ }}comparing{\text{ }}equation(1){\text{ }}and{\text{ }}(2),{\text{ }}we{\text{ }}get \\
\Rightarrow \dfrac{{{B'}F}}{{FP}} = \dfrac{{{B'}P - FP}}{{FP}} = \dfrac{{{B'}P}}{{BP}} \\
\Rightarrow {B'}P = - v,FP = - f,BP = - u \\
U\sin g{\text{ }}these{\text{ }}in{\text{ }}equation,{\text{ }}we{\text{ }}get \\
\Rightarrow \dfrac{{ - v + f}}{{ - f}} = \dfrac{{ - v}}{{ - u}} \\
\Rightarrow \dfrac{{v - f}}{f} = \dfrac{v}{u} \\
On{\text{ }}further{\text{ }}solving,{\text{ }}we{\text{ }}get \\
\therefore \dfrac{1}{v} + \dfrac{1}{u} = \dfrac{1}{f} \\
$
Note: In a spherical mirror, the distance of the object from its pole is called the object distance (u). The distance of the image from the pole of the mirror is called the image distance (v). The distance of the principal focus from the pole is called the focal length (f). There is a relationship between these three quantities given by the mirror formula which is expressed as $\dfrac{1}{v} + \dfrac{1}{u} = \dfrac{1}{f}$.This formula is valid in all situations for all spherical mirrors for all positions of the object.
Complete step by step solution:
Derivation of the Mirror Formula

We now derive the mirror equation or the relation between the object distance (u), image distance (v) and the focal length (f). In the above figure, the two right-angled triangles A′B′F and MPF are similar.
$
\Rightarrow \dfrac{{{B'}{A'}}}{{PM}} = \dfrac{{{B'}F}}{{FP}} \\
\Rightarrow \dfrac{{{B'}{A'}}}{{BA}} = \dfrac{{{B'}F}}{{FP}} \\
\because PM = AB...............(1) \\
\Rightarrow \angle APB = \angle {A'}PB \\
\therefore \Delta {A'}{B'}{P'} \sim \Delta A{B'}P \\
\therefore \dfrac{{{B'}{A'}}}{{BA}} = \dfrac{{{B'}P}}{{BP}}............(2) \\
By{\text{ }}comparing{\text{ }}equation(1){\text{ }}and{\text{ }}(2),{\text{ }}we{\text{ }}get \\
\Rightarrow \dfrac{{{B'}F}}{{FP}} = \dfrac{{{B'}P - FP}}{{FP}} = \dfrac{{{B'}P}}{{BP}} \\
\Rightarrow {B'}P = - v,FP = - f,BP = - u \\
U\sin g{\text{ }}these{\text{ }}in{\text{ }}equation,{\text{ }}we{\text{ }}get \\
\Rightarrow \dfrac{{ - v + f}}{{ - f}} = \dfrac{{ - v}}{{ - u}} \\
\Rightarrow \dfrac{{v - f}}{f} = \dfrac{v}{u} \\
On{\text{ }}further{\text{ }}solving,{\text{ }}we{\text{ }}get \\
\therefore \dfrac{1}{v} + \dfrac{1}{u} = \dfrac{1}{f} \\
$
Note: In a spherical mirror, the distance of the object from its pole is called the object distance (u). The distance of the image from the pole of the mirror is called the image distance (v). The distance of the principal focus from the pole is called the focal length (f). There is a relationship between these three quantities given by the mirror formula which is expressed as $\dfrac{1}{v} + \dfrac{1}{u} = \dfrac{1}{f}$.This formula is valid in all situations for all spherical mirrors for all positions of the object.
Recently Updated Pages
Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which are the Top 10 Largest Countries of the World?

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Draw a labelled sketch of the human eye class 12 physics CBSE

What is a transformer Explain the principle construction class 12 physics CBSE

How much time does it take to bleed after eating p class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE




