
What is the angular displacement for the minute hand of a clock in 600 seconds?
Answer
530.4k+ views
Hint: Angular displacement of a body is the angle in radians through which a point revolves around a centre or a line that has been rotated in a specific sense about a specified axis. So we need to find the value of the angle in degrees for the minute hand of a clock in 600 seconds.
Complete answer:
We know that,
60 minutes is equal to 1 degree
So, 1 minute will be equal to ${{\dfrac{1}{60}}^{\circ }}$ (According to unitary method)
In other words, we can say,
60 seconds is equal to ${{\dfrac{1}{60}}^{\circ }}$
$\therefore $ 1 second is equal to ${{\dfrac{1}{60\times 60}}^{\circ }}$
Hence, in 600 seconds, the displacement of the minute hand is \[{{\dfrac{360\times 600}{60\times 60}}^{\circ }}\] = 60\[^{\circ }\]
Thus, the total angular displacement of the minute hand of the clock is 60 degrees.
Additional Information:
It is very important to keep in mind that 60 minutes is equal to 1 degree and 60 seconds is equal to 1 minute. Such information must be kept in mind while solving problems of this kind. There is another formula for angular displacement besides calculating the angular displacement by unitary method. The formula is as follows:
$\theta =\dfrac{l}{r}$ , where angular displacement is given by the length of the arc divided by the radius of the circle.
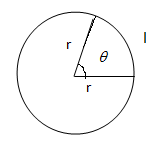
Fig: diagram showing the angular displacement, $\theta $
Angular displacement is generally calculated in radians, but it can also be expressed in degrees or revolutions per second.
Note:
Angular displacement is usually expressed in radians. While solving problems as such, it must be remembered that angular displacement can be calculated by calculating the length of the arc and the radius of the circular motion or even from minutes and seconds by directly calculating the angle. Angular displacement is simply the displacement from initial point to final point in curvilinear motion.
Complete answer:
We know that,
60 minutes is equal to 1 degree
So, 1 minute will be equal to ${{\dfrac{1}{60}}^{\circ }}$ (According to unitary method)
In other words, we can say,
60 seconds is equal to ${{\dfrac{1}{60}}^{\circ }}$
$\therefore $ 1 second is equal to ${{\dfrac{1}{60\times 60}}^{\circ }}$
Hence, in 600 seconds, the displacement of the minute hand is \[{{\dfrac{360\times 600}{60\times 60}}^{\circ }}\] = 60\[^{\circ }\]
Thus, the total angular displacement of the minute hand of the clock is 60 degrees.
Additional Information:
It is very important to keep in mind that 60 minutes is equal to 1 degree and 60 seconds is equal to 1 minute. Such information must be kept in mind while solving problems of this kind. There is another formula for angular displacement besides calculating the angular displacement by unitary method. The formula is as follows:
$\theta =\dfrac{l}{r}$ , where angular displacement is given by the length of the arc divided by the radius of the circle.

Fig: diagram showing the angular displacement, $\theta $
Angular displacement is generally calculated in radians, but it can also be expressed in degrees or revolutions per second.
Note:
Angular displacement is usually expressed in radians. While solving problems as such, it must be remembered that angular displacement can be calculated by calculating the length of the arc and the radius of the circular motion or even from minutes and seconds by directly calculating the angle. Angular displacement is simply the displacement from initial point to final point in curvilinear motion.
Recently Updated Pages
Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Trending doubts
1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

What is the opposite of entropy class 11 chemistry CBSE

Proton was discovered by A Thomson B Rutherford C Chadwick class 11 chemistry CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




