
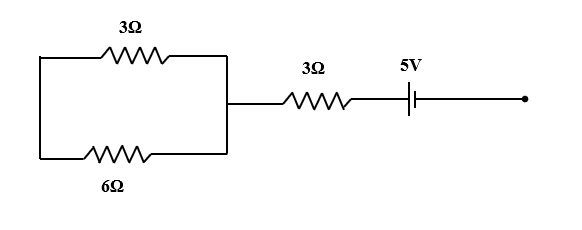
A $3\Omega$ resistor and a $6\Omega$ resistor are connected in parallel and the combination is connected in series to a battery of 5V and a $3\Omega$ resistor. The potential difference across the $6\Omega$ resistor
A. 2V
B. 4V
C. 3V
D. 1V

Answer
538.2k+ views
Hint: To solve this problem, first find the equivalent resistance across $3\Omega$ and $6\Omega$ resistors. Then, find the total resistance in the circuit. Substitute the total resistance and the voltage of the batter in the formula for Ohm’s law. This will give the current flowing through the circuit. Then, find the current passing through $6\Omega$ resistor. Now, using this obtained current across $6\Omega $ resistor, find the potential difference across $6\Omega$ resistor.
Complete answer:
Given: ${ R }_{ 1 }=3\Omega$
${ R }_{ 2 }=6\Omega$
${ R }_{ 1 }=3\Omega$
Voltage (V)= 5V
Resistors ${ R }_{ 1 }$ and ${ R }_{ 2 }$ are connected in parallel.
So, the equivalent resistance in the combination is given by,
$\dfrac { 1 }{ { R }_{ eq } } =\dfrac { 1 }{ { R }_{ 1 } } +\dfrac { 1 }{ { R }_{ 2 } }$
Substituting the values we get,
$\dfrac { 1 }{ { R }_{ eq } } =\dfrac { 1 }{ 3 } +\dfrac { 1 }{ 6 }$
$\Rightarrow \dfrac { 1 }{ { R }_{ eq } } =\dfrac { 9 }{ 18 }$
$\Rightarrow { R }_{ eq }=\dfrac { 18 }{ 9 }$
$\therefore {R}_{eq} = 2\Omega$
Therefore, the total resistance in the circuit is,
$R= {R}_{eq}+ {R}_{3}$
Substituting the values we get,
$R= 2 + 3$
$\Rightarrow R= 5\Omega$
Using Ohm’s Law,
$V=IR$
Substituting the values we get,
$5= I \times 5$
$\Rightarrow I =1A$
Current flowing through $6\Omega$ resistor is given by,
${ I }^{ ' }=\dfrac { { R }_{ 3 } }{ { R }_{ 3 }+{ R }_{ 2 } } I$
Substituting the values in above expression we get,
${ I }^{ ' }=\dfrac { 3 }{ 3+6 } \times 1$
$\Rightarrow { I }^{ ' }=\dfrac { 3 }{ 9 }$
$\Rightarrow { I }^{ ' }=\dfrac { 1 }{ 3 }$
Voltage across $6\Omega $ resistor is given by,
${ V }^{ ' }={ I }^{ ' }\times { R }_{ 2 }$
Substituting the values in above equation we get,
${ V }^{ ' }=\dfrac { 1 }{ 3 } \times 6$
$\therefore { V }^{ ' }= 2V$
Hence, the potential difference across the $6\Omega$ resistor is 2V.
So, the correct answer is “Option A”.
Note:
Students generally get confused between the formula for equivalent resistance in parallel combination and series combination & the equivalent capacitance in series and parallel combination. So, they should clearly know these formulas to solve these types of problems. Students should also remember that the equivalent resistance of a combination is always less than the smallest resistance in the parallel network.
Complete answer:
Given: ${ R }_{ 1 }=3\Omega$
${ R }_{ 2 }=6\Omega$
${ R }_{ 1 }=3\Omega$
Voltage (V)= 5V
Resistors ${ R }_{ 1 }$ and ${ R }_{ 2 }$ are connected in parallel.
So, the equivalent resistance in the combination is given by,
$\dfrac { 1 }{ { R }_{ eq } } =\dfrac { 1 }{ { R }_{ 1 } } +\dfrac { 1 }{ { R }_{ 2 } }$
Substituting the values we get,
$\dfrac { 1 }{ { R }_{ eq } } =\dfrac { 1 }{ 3 } +\dfrac { 1 }{ 6 }$
$\Rightarrow \dfrac { 1 }{ { R }_{ eq } } =\dfrac { 9 }{ 18 }$
$\Rightarrow { R }_{ eq }=\dfrac { 18 }{ 9 }$
$\therefore {R}_{eq} = 2\Omega$
Therefore, the total resistance in the circuit is,
$R= {R}_{eq}+ {R}_{3}$
Substituting the values we get,
$R= 2 + 3$
$\Rightarrow R= 5\Omega$
Using Ohm’s Law,
$V=IR$
Substituting the values we get,
$5= I \times 5$
$\Rightarrow I =1A$
Current flowing through $6\Omega$ resistor is given by,
${ I }^{ ' }=\dfrac { { R }_{ 3 } }{ { R }_{ 3 }+{ R }_{ 2 } } I$
Substituting the values in above expression we get,
${ I }^{ ' }=\dfrac { 3 }{ 3+6 } \times 1$
$\Rightarrow { I }^{ ' }=\dfrac { 3 }{ 9 }$
$\Rightarrow { I }^{ ' }=\dfrac { 1 }{ 3 }$
Voltage across $6\Omega $ resistor is given by,
${ V }^{ ' }={ I }^{ ' }\times { R }_{ 2 }$
Substituting the values in above equation we get,
${ V }^{ ' }=\dfrac { 1 }{ 3 } \times 6$
$\therefore { V }^{ ' }= 2V$
Hence, the potential difference across the $6\Omega$ resistor is 2V.
So, the correct answer is “Option A”.
Note:
Students generally get confused between the formula for equivalent resistance in parallel combination and series combination & the equivalent capacitance in series and parallel combination. So, they should clearly know these formulas to solve these types of problems. Students should also remember that the equivalent resistance of a combination is always less than the smallest resistance in the parallel network.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Trending doubts
When was the first election held in India a 194748 class 12 sst CBSE

Which are the Top 10 Largest Countries of the World?

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

What are the monomers and polymers of carbohydrate class 12 chemistry CBSE

Amount of light entering the eye is controlled by A class 12 physics CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?




