Ways To Multiply And Divide - Exercise-wise Questions and Answers For Class 5 Maths - Free PDF Download
NCERT Class 5 Maths Chapter 13 Solutions of Ways to Multiply and Divide by Vedantu introduces students to the various techniques and strategies for multiplication and division, two of the most important arithmetic operations. Different methods for multiplying and dividing numbers, including traditional algorithms, shortcuts, and mental maths techniques.
 Table of Content
Table of ContentVedantu’s Class 5 Maths NCERT solutions provide clear, step-by-step explanations to help you understand these concepts easily. Each problem is solved in a detailed manner to ensure you grasp the methods and can apply them confidently. Access the latest CBSE Class 5 Maths Syllabus here.
Glance on Maths Chapter 13 Class 5 - Ways to Multiply and Divide
In Class 5 Chapter 13 introduces students to the different methods of multiplication and division.
Multiplication of numbers in columns - For multiplying two or three-digit numbers with another numerical consisting of more than one digit.
Division of numbers - Dividing a number which is 3 to 4 digits long with a single digit number.
Dividing a number with remainder, where the numbers are not dividable at some point.
Dividing a number without remainder which is divisible and gives the remainder as zero.
This article contains chapter notes, important questions, exemplar solutions and video links for Chapter 13 - Ways to Multiply and Divide, which you can download as PDFs.
Access NCERT Solutions for Class 5 Maths Chapter 13 Ways to Multiply and Divide
1. Maniratnam – The Cashier
Maniratnam is the cashier of king Jayan. His job is to find out the salary of all the people who work for the king. This chart shows how much salary each person gets in a day
Person Salary in a day
Minister — ₹ 195
Horse rider — ₹ 76
Cook — ₹ 65
Maniratnam wanted to calculate the salary of the cook for the month of January. He wrote —

Rupees 1800 + 150 + 60 + 5 = ₹ ________
Ans: Rupees 1800 + 150 + 60 + 5 = ₹(1800+210) = ₹ 2015
2. Maniratnam’s daughter Bela has learnt another method to multiply. She wrote like this and showed it to Bhanu, her brother.
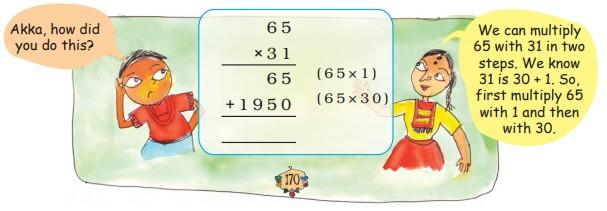
Ans:

3. Now Bhanu tried to find the salary of a minister for the month of January. He wanted to multiply 195 × 31.

Ans:

Practice Time
1. Use Bela‘s method to multiply these numbers.
a) 32 $\times$ 46
b) 67 $\times$ 18
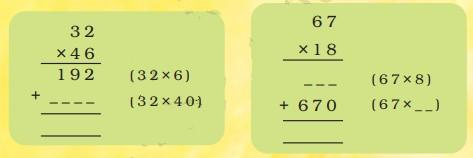
Ans:
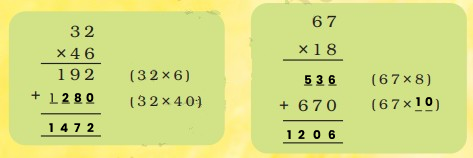
2. Do these in your notebook using Bela’s method.
a) 47 $\times$ 19 b) 188 $\times$ 91
c) 63 $\times$ 57 d) 225 $\times$ 22
e) 360 $\times$ 12 f ) 163 $\times$ 42
Ans:
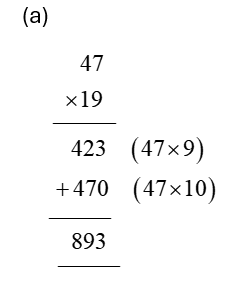


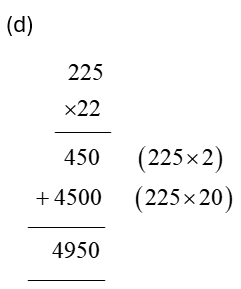

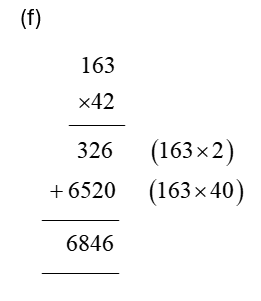
Shantaram a Special Cook
1. Shantaram is a special cook who comes only on party days. Last year he was called for only 28 days. For each day he has to be paid ` 165. Find out how much money he will get in all.
Ans: Given that, Salary paid to Shantaram for 1 day = Rs 165
So, Salary paid to shantaram for 28 days = Rs 165 $\times$ 28
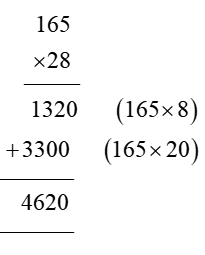
Hence, the money that Shantaram gets in all is Rs. 4620
2. If he is called for all days of the year, how much salary will he get?
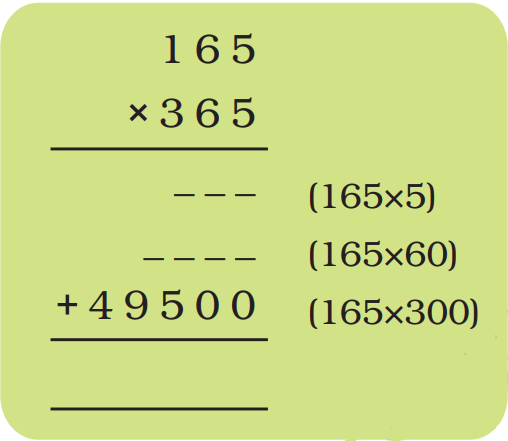
Ans:

Thus, Shantaram the cook will get a salary of Rs 60,225
3. Now find the salaries of the minister and horse rider for 1 year
Ans: The salary that is paid to the minister for 1 day = Rs 195
So, the salary paid to the minister for 365 days = Rs 195 $\times$ Rs 365
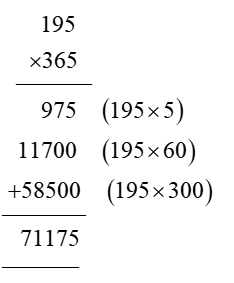
Hence, the salary of the minister for 1 year is Rs 71,175
And,
The salary that is paid to the horse rider for 1 day = Rs 95
So, the salary paid to the minister for 365 days = Rs 95 $\times$ Rs 365
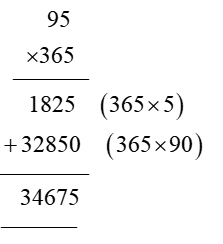
Hence, the salary of the horse rider for 1 year is Rs 34,675
Years and Years
a) Sohan drinks 8 glasses of water every day.
How many glasses will he drink in one month? ___________
How many glasses will he drink in one year?
If 125 people living in a colony drink 8 glasses of water in a day, how much water will they drink in a year?
Ans: The number of glasses of water Sohan drinks everyday = 8
We know that, 1 month = 30 days
So, the number of glasses of water consumed by Sohan = 8 $\times$ 30

Hence, Sohan will drink 240 glasses of water in 1 month.
Now, we also know that 1 year = 365 days
So, the number of glasses of water consumed by Sohan in 365 days = 365 $\times$ 8
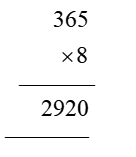
Hence, Sohan will drink 2,920 glasses of water in 1 year.
Now, we know that the number of glasses of water consumed by one person fpr a year = 2,920
0, the number of glasses of water consumed by 125 people of the colony for one year = 2920 $\times$ 125

Therefore, 125 people of the colony will drink 3,65,00 glasses of water in one year.
b) If Soha's heart beats 72 times in one minute, how many times does it beat in one hour?
Now find out how many times it beats in one day.
Count your own heart beats to find out how many times your heart beats in one week.
Ans: Given that, the number of times Soha’s heart beats in one minute =72
We know, 1 hour = 60 minutes
So, the number of times Soha’s heart beats in one hour = 72 $\times$ 60
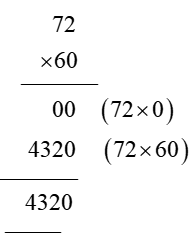
Hence, Sohan’s heart beats 4,320 times in one hour.
Now, we know that one day has 24 hours.
So, the number of times Soha’s heart beats in a day = 4320 $\times$ 24

Hence, the number of times Soha’s heart beat in a day is = 1,03,680
My heart beats 72 times in a minute. We know that there are 7 days in a week.
So, the number if times my heart beats in one week = 1,03,680 $\times$ 7
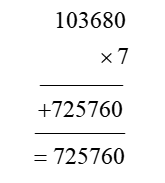
Hence, the number of times my heart beats in a week is 7,25,760.
c) A baby elephant drinks around 12 L of milk every day. How much milk will it drink in two years?
Ans: The number of litres of milk the baby elephant drinks in a day = 12L
We know that the number of days in a year = 365
And the number of days in two years = 2 $\times$ 365 = 730
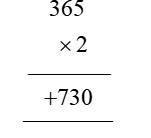
So, the number of litres of milk the baby elephant drinks in two years = 12 $\times$ 730
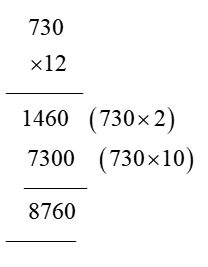
Hence, the number of litres of milk the baby elephant will consume in two years is 8,760 Litres.
d) A baby blue whale drinks around 200 L of milk in one day. Just imagine how much is! Find out in how many days your family would use 200 L milk. How much milk would the baby blue whale drink in eight months?
Ans: Number of litres of milk a baby blue whale drinks in one day = 200
Number of litres of milk used in my family in one day =2 L
So, the number of days for consuming 200 L of milk by my family = $\dfrac{200}{2}$ = 100 days
We know that 1 month = 30 days then 8 months = 8 $\times$ 30 = 240 days
Now, we can calculate the number of litres of milk the baby blue whale will drink in 8 months = 200 $\times$ 240
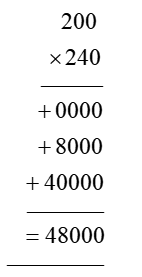
Therefore, the baby blue whale will consume 48,000 L of milk in 8 months.
Karunya — The Landlord
Karunya bought three fields.
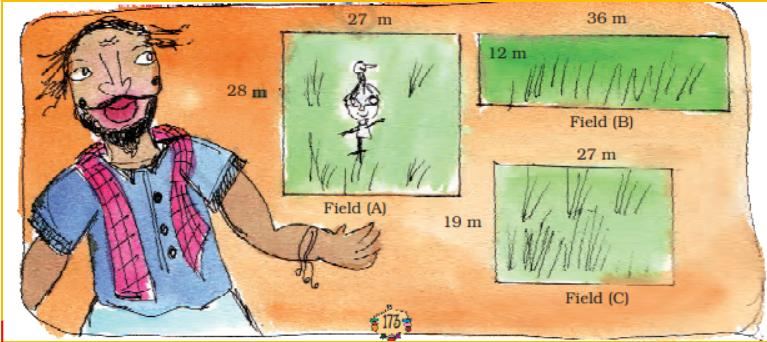
1. Find the area of all the three fields.
(i) Field (A) ____________ square metre.
(ii) Field (B) ____________ square metre.
(iii) Field (C) ____________ square metre.
Ans:
(i) Given dimensions of field (A) is Length(l) = 27m and breadth(b) = 28m
We know that Area (A) = l $\times$ b = 27 $\times$ 28
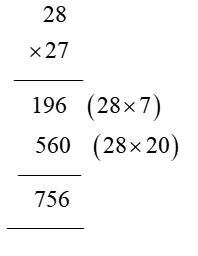
Field (A) is 756 square metre.
(ii) Given dimensions of field (B) is Length(l) = 36m and breadth(b) = 12m
We know that Area (B) = l $\times$ b = 36 $\times$ 12
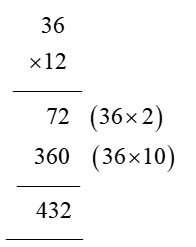
Field (B) is 432 square metre.
(iii) Given dimensions of field (C) is Length(l) = 27m and breadth(b) = 19m
We know that Area (C) = l $\times$ b = 27 $\times$ 19

2. He bought field (A) at the rate of ₹ 95 for a square metre, field (B) at ₹ 110 for a square metre and field (C) at ₹ 120 for a square metre.
Find the cost of all three fields.
Ans: Cost of 1 Square metre of field(A) = Rs 95
So, the cost of 756 square metre of field(A) = 745 $\times$ 95

Therefore, the cost of field(A) is Rs. 71,820
Now, cost of 1 square metre of field(B) is Rs 110
So, the cost of 432 square metre of field(B) = 432 $\times$ 110

Therefore, the cost of field(B) is Rs. 47,520
Now, cost of 1 square metre of field(C) is Rs 120
So, the cost of 513 square metre of field(B) = 513 $\times$ 120
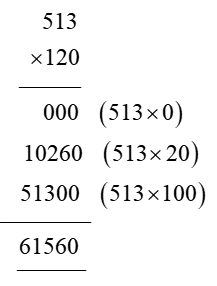
Therefore, the cost of field(C) is Rs. 61,560
Now, the total cost of all the fields is = Rs. 71,820 + Rs. 47,520 + Rs. 61,560 = Rs. 1,80,900
Thulasi and her husband work on Karunya’s farm. The Government has said that farm workers should be paid at least Rs 71 for one day’s work. But he pays Rs 55 to Thulasi and Rs 58 to her husband.
(i) If Thulasi works for 49 days, how much money does she get? ____
(ii) If her husband works for 42 days, how much money does he get? ________
(iii) Find the money they earn together __________
Ans:
(i) Given that,
Thulasi is paid Rs 55 for one day’s work.
So, if she works for 49 days, she will get= Rs 55 $\times$ 49
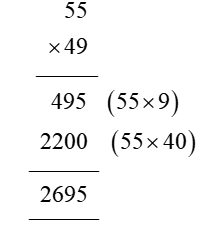
Therefore, Thulasi will get Rs 2,695 for working 49 days in the form.
(ii) Given that,
Thulasi’s husband is paid Rs 58 for one day's work.
So, if he works for 42 days, he will get = Rs 58 $\times$ 42
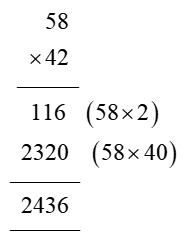
Therefore, Thulasi’s husband will get Rs 2,436 for working 42 days in the form.
(iii) Total money earned by Thulasi and her husband together= Rs 2,695 + Rs. 2,436 = Rs. 5,131
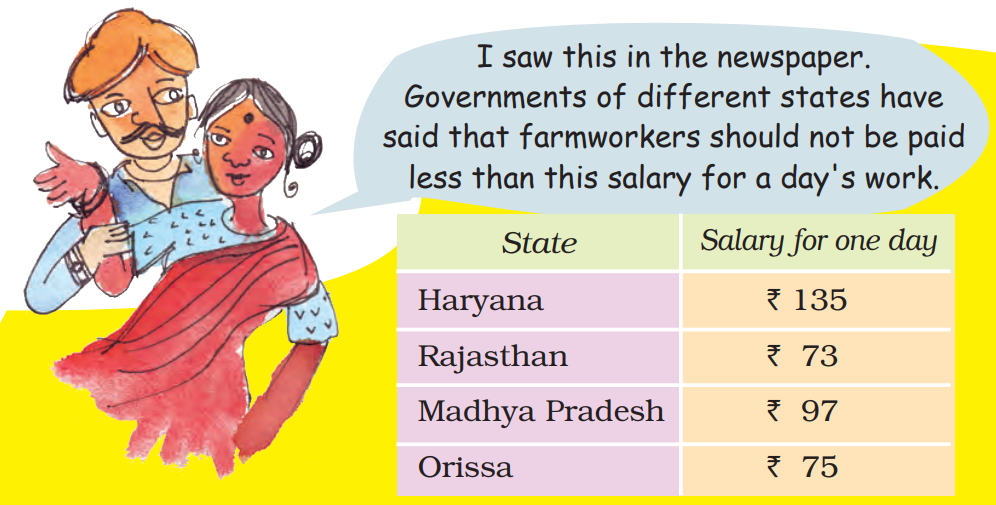
The table shows the amounts fixed by four states.
a) For farm work which state has fixed the highest amount? Which state has fixed the lowest?
b) Bhairon Singh is a worker in Rajasthan. If he works for 8 weeks on the farm, how much will he earn?
c) Neelam is a worker in Haryana. If she works for 2½ months on the farm, how much will she earn?
d) How much more will a farm worker in Madhya Pradesh get than a worker in Orissa after working for 9 weeks?
Ans:
(a) For farm work, Haryana has the highest amount of Rs 135 for one day. And the lowest amount is for Rajasthan paying Rs 73 for one day.
(b) Bhairon singh works in Rajasthan, so he will be paid Rs 73 for one day.
We know that 1 week has 7 days
Then 8 weeks = 7 $\times$ 8 = 56 days
So, Bhairon Singh’s earnings for working in the farm for 56 days = Rs 73 $\times$ 56
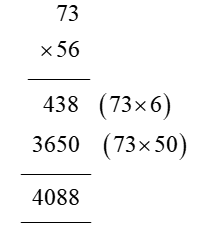
Therefore, Bhairon Singh will earn Rs. 4088 in 8 weeks.
(C) Neelam is working in Haryana so she will be paid Rs 135 for one day.
We know that, 1 month has 30 days and ½ month = $\dfrac{30}{2}$ = 15 days.
Then, 2 ½ month = (2 $\times$ 30) + 15 = 75 days
So, the earnings of Neelam for working in the farm for 2 ½ months = Rs 135 $\times$ 75
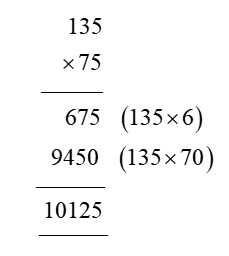
Therefore, Neelam will earn Rs. 10,125 in 2 ½ months.
(d) The salary of a farm workers for one day in Madhya Pradesh = Rs 97
We know that 1 week has 7 days
Then, 9 weeks = 9 $\times$ 7 = 63 days
So, the earnings of a farm worker working for 63 days = Rs 97 $\times$ 63
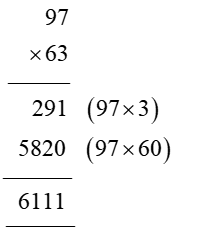
Therefore, a farm worker will get Rs 6,111 in 9 weeks working in Madhya Pradesh.
Now, the salary of a farm workers for one day in Orissa = Rs 75
We know that 1 week has 7 days
Then, 9 weeks = 9 $\times$ 7= 63 days
So, the earning of a farm worker working for 63 days = Rs 75 $\times$ 63

Therefore, a farm worker will get Rs 4,725 in 9 weeks working in Madhya Pradesh.
The difference between the earning of the farm workers = Rs 6,111 - Rs 4,725 = Rs 1,386
Thus, a farm worker in Madhya Pradesh will earn Rs 1,386 more than a farm worker in Orissa after working for 9 weeks.
Satish’s story
Satish is a 13 year old boy. His father had taken a loan for farming. But the crops failed. Now Satish’s mother has to pay Rs 5000 every month for the loan.
Satish started working — he looked after 17 goats of the village.
He earns Rs 1 everyday for one goat.
How much will he earn in one month?
Does he earn enough to help pay the loan every month?
How much will he earn in one year?
Ans: Given that, Satish earns Rs 1 for one goat every day. He looked after 17 goats of his village
So, he will earn = Rs 1 $\times$ 17 = Rs 17 for a day
We know that 1 month = 30 days
For a month he will earn = Rs 17 $\times$ 30
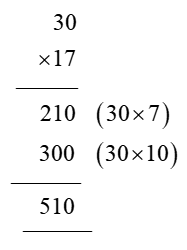
Therefore, Satish will earn Rs 510 in one month.
Satish’s mother has to pay Rs 5,000 every month for the loan. But, the earnings of Satish will not help to pay the loan every month.
We know that, 1 year = 12 months
Satish’s earnings in one year = Rs 510 $\times$ 12
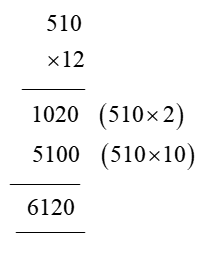
Thus, Satish will earn Rs 6,120 in one year for looking after 17 goats.
Kamla Bai’s story
To help farmers the State Government gave cows. Kamla Bai Gudhe also got a cow. The cost of the cow was Rs 17,500. She had to pay Rs 5,500 and the government spent the rest of the money.
(i) How much did the government spend on the cow?
(ii) If 9 people from her village got cows, how much did the government spend in all?
But Kamla Bai was not happy. She had to spend Rs 85 everyday on the cow. She made some money by selling the milk. But still she wanted to sell the cow.
(iii) If Kamla Bai spends Rs 85 a day, find out how much she will spend in one month.
(iv) he cow gives 8 litre of milk everyday. How much will it give in one month?
(v) If the milk is sold at Rs 9 per litre, how much money will Kamla Bai make in one month? __________
So the money spent on keeping the cow was Rs _______
Money earned by selling the milk Rs _________
Which is more — money spent on the cow or money earned from it? How much?
(vi) Explain why she wanted to sell the cow.
Ans:
(i) Given that, Kamala Bai paid Rs 5,500 for getting the cow.
The cost of the cow = Rs 17,500
So, the amount government spent on the cow = Rs (17,500 - 5,500)
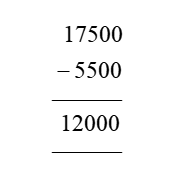
Therefore, the government spent Rs 12,000 on one single cow.
(ii) We know that money spent by the government for one cow = Rs 12,000
So, for 9 cows the money spent by the government for one day = Rs 12,000 $\times$ 9
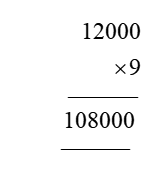
Therefore, the government spent Rs 1,08,000 on 9 cows for 9 people.
(iii) Given that, Kamal Bai spends Rs 85 a day
We know that 1 month = 30 days
So, the money spent by Kamala Bai in one month = Rs 85 $\times$ 30

Therefore, Kamala Bai will spend Rs 2,550 on the cows in one month.
(iv) The cow gives 8 litres a day
We know that 1 month = 30 days
So, the amount of milk the cow will give in 1 month = 8 L $\times$ 30

Therefore, the cow will give 240 litres of milk in 1 month.
(v) The milk is sold per Rs 9 per litre.
Then, the selling price for 240 litres odf milk = Rs 9 $\times$ 240
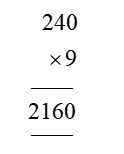
Therefore, Kamala Bai will earn Rs 2,160 by selling 240 litres of milk in 1 month.
So, the money spent for keeping the cow was Rs 2,550
Money earned by selling the milk Rs 2,160
The money spent on the cow is more as compared to the money earned by the cow.
= Money spent for the cow - Money earned by selling the milk
= Rs 2,550 - Rs 2,160
= Rs 390
Therefore, the money spent on keeping the cow is Rs 390 more than the money earned by selling the milk.
(vi) She wanted to sell the cow because she has to spend more money to take care of the cow compared to the money that she earns by selling the milk of the cow.
Practice Time
a) Sukhi works on a farm. He is paid Rs 98 for one day. If he works for 52 days, how much will he earn?
b) Hariya took a loan to build his house. He has to pay back Rs 2,750 every month for two years. How much will he pay back in 2 years?
c) Ratiram is a milk seller in the city. He sells 13 litres of milk everyday at Rs 23 per litre. How much does he earn?
d) A farmer sells 1 litre of milk for Rs 11. In one month he sells 210 litres of milk. How much does he earn in a month?
e) A company sells 1 litre of packed water for Rs 12. A shopkeeper buys 240 litres of packed water. How much does he pay?
Ans:
(a) Given that, Sukhi is paid Rs 98 for working 1 day on a farm.
So, his earning if he works 52 days = Rs 98 $\times$ 52
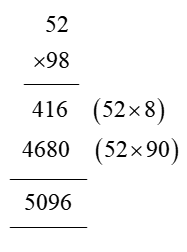
Therefore, Sukhi will earn Rs 5,096 by working in farm for 52 days
(b) Given that Hariya pays back Rs 2,750 every month.
She has to pay the total amount for a period of 2 years.
We know that 2 years = 24 months
Therefore =n Rs 2,750 $\times$ 24
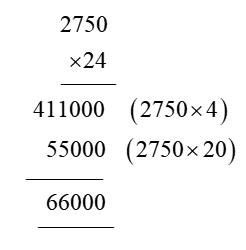
Therefore, Hariya will pay back Rs 66,000 in 2 years to complete the loan
(c) The earnings of Ratiram by selling 1 litre of milk = Rs 23
Then the earnings of Ratiram by selling 13 litres of milk = Rs 23 $\times$ 13
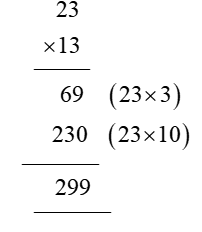
Therefore, Ratiram earns Rs 299 after selling 13 litres of milk every day.
(d) The farmer sells 1 litres of milk at Rs 11.
So, the earning of selling 210 litres of milk in one month = Rs 11 $\times$ 210
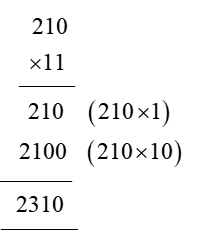
Therefore, the farmer earns Rs 2,310 in a month by selling milk.
(e) Selling price of 1 litre of packed water = Rs 12
Then the amount the shopkeeper has to pay for buying 240 litres of packed water = Rs 12 $\times$ 240

Therefore, the shopkeeper has to pay Rs 2,880 for 240 litres of packed water.
Fun With Multiplication
A) Look for the pattern and take this forward
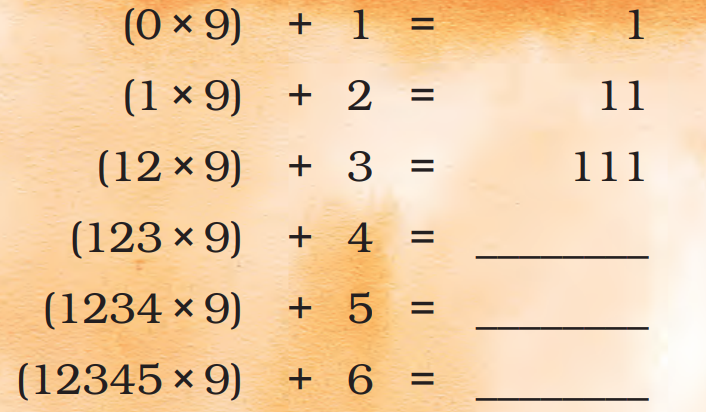
Ans:
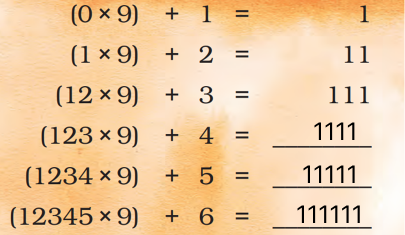
B) Each letter a,b, c stands for a number.

Take a=1, then find what numbers b and c will be.
Ans:
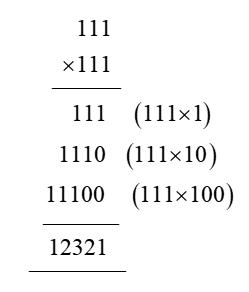
Therefore, the numbers b=2 and c=3.
C) Tricks with your age.
Writeyourage_____________
Multiply itby 7___________
Again multiply the answer by13 __________
Multiply again that answer by11 __________
Now look at your last answer. Can you find your age in that answer? How many times does your age showin the answer?
Now try this trick with other people.
Ans: Let’s take your age = 10
Multiply it by 7 = 10 $\times$ 7 = 70
Now again multiply the answer by 13 = 70 $\times$ 13 = 910
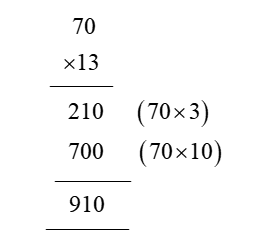
Multiply the above answer by 11 = 910 $\times$ 11 = 10010
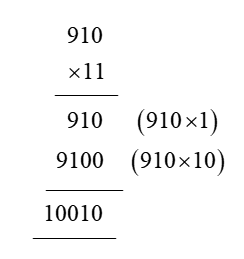
Yes, I can find my age in the final answer. My age appears 2 times in answer.
D) Going round and round!
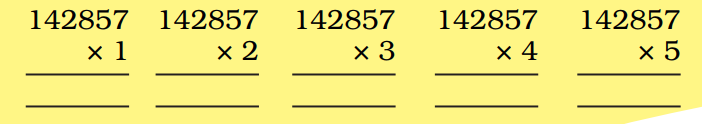
Ans:

Do you find a pattern in all these answers? Discuss this with your friends.
Ans: No, we didn't find any pattern in the answer.
Division
Dolma took a loan from a friend to buy a moped for Rs 9,588. She has to pay it back in equal amounts every month for six months.
How much will she have to pay every month? She asked her children to calculate.
Will both of them get the same answer? Discuss.


Ans: The answer calculated by her daughter = 500+500+500+90+8 =1598
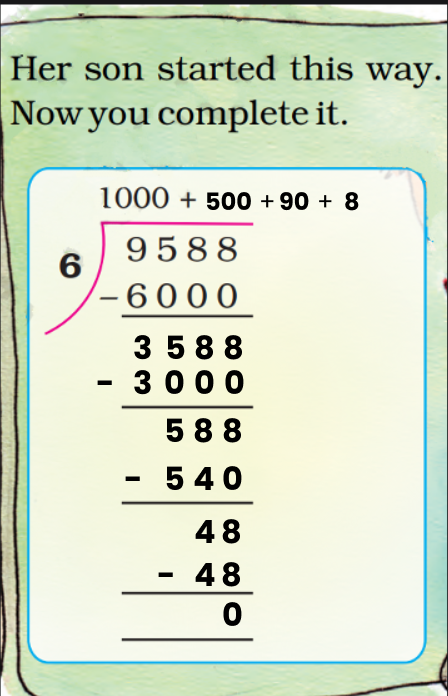
The answer calculated by her son = 1000+500+90+8=1598
So, both the children get the same answer = 1598
Therefore, Dolma has to pay a sum of Rs 1598 every month for 6 months to her friend.
Practice Time
Try to solve these using as few steps as you can.
a) 4228 ÷ 4 b) 770 ÷ 22 c) 9872 ÷ 8
d) 672 ÷ 21 e) 772 ÷ 7 f ) 639 ÷ 13
Ans:

How Much Petrol?
Isha has Rs 1000 with her. She wants to buy petrol. One litre of petrol costs ` 47. How many litres can she buy?
Money with Isha = Rs 1000
Cost of 1litre = Rs 47
Litres of petrol she can buy = Rs 1000 ÷Rs 47 = ?
Isha can buy ______ litres of petrol.
Ans: Dividing Rs 1000 ÷ Rs 47 we have,

Here,
Remainder =13
Therefore, Isha can only buy 21 litres of petrol with Rs 1000.
Find out
If Isha comes to your city, how much petrol can she buy with the same money?
Ans: The cost of petrol in my city = Rs 50
So, the number of litres of petrol she can buy with Rs 1000 = 1000 ÷ 50
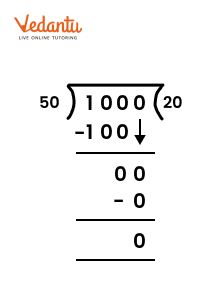
Therefore, Isha can buy 20 litres of petrol in my city.
Children’s Day
Children are happy today. They are celebrating Children’s Day. Each child will be given 4 coloured pencils from school. The school has got 969 pencils. To find out how many children can get pencils the teacher asks them to divide.
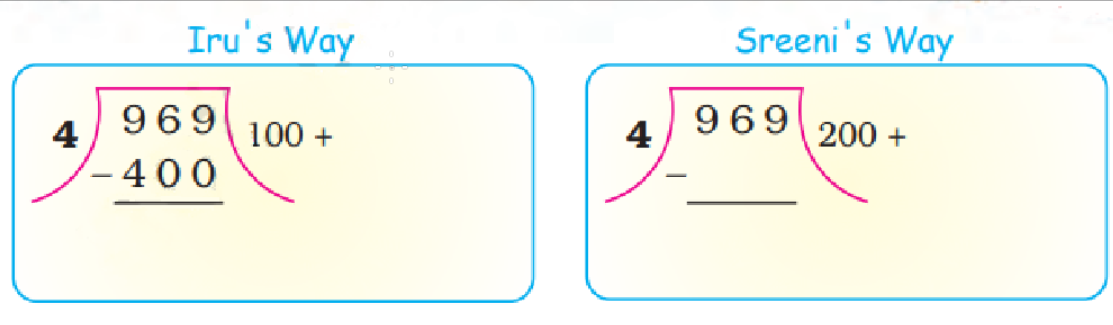
Complete Iru’s and Sreeni’s way of division. What is the answer you get?
Ans:

In both the ways we got the same answer which is 242
Therefore, 242 students can get the colour pencils.
Practice Time
1. 576 books are to be packed in boxes. If one box has 24 books, how many boxes are needed?
Ans: Total number of books to be packed in boxes = 576
Number of books that one box can have = 24
Then, the total number of boxes requires to put 576 books = 576 ÷ 24
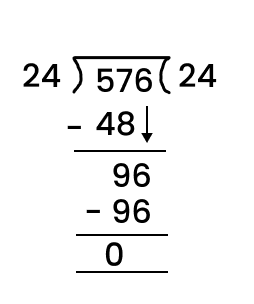
Therefore, 24 boxes are required to pack 576 books.
2. 836 people are watching a movie in a hall. If the hall has 44 rows, how many people can sit in 1 row?
Ans: Given that, the total number of people watching movie in the hall = 836
Number of rows in the hall = 44
Then, the number of people sitting in one row = 836 ÷ 44

Therefore, 19 people are sitting in 1 row in the hall.
3. A gardener bought 458 apple trees. He wants to plant 15 trees in each row. How many rows can he plant?
Ans: Given the total number of trees bought by gardener = 458
Number if trees planted in one row = 15
So the number of rows he can plant = 458 ÷ 15
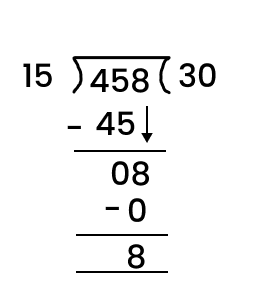
Therefore, the gardener can plant 30 rows of apple trees and he will be left with 8 trees.
Brain Teaser
1. Shyamli bought a battery. She read on it Life: 2000 hours . She uses it throughout the day and the night. How many days will the battery run?
Ans: Given that the life of the battery = 200 hours
She uses it all day and night, i.e. for 24 hours
So, the number of days the battery will run = 2000 ÷ 24

Therefore, the battery will run for 83 days.
More with Multiplication and Division
1. A tank is full of 300 L of water. How much water will be filled in 25 tanks? If 15 buckets can be filled with one tank of water, how many buckets in all can be filled with the water in 25 tanks?
Ans: Given that, the amount of water in 1 tank = 300 L
Then, the amount of water in 25 tanks = 25 $\times$ 200 = 7500 L
The number of buckets that can be filled with one tank of water = 15
So, the number oif buckets that can be filled with 25 tanks of water = 25 $\times$ 15

Therefore, the total number of buckets that can be filled with 25 tanks of water is 375
2. There are 28 in 1 kg. How many will be there in 12 kg? If 16 can be packed in 1 box, how many boxes are needed to pack all these laddoos?
Ans: Given that, the number of laddoos in 1 kg = 28
Then, the number of laddoos in 12 kg = 28 $\times$ 12
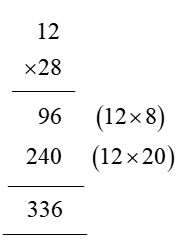
Therefore, there will be 336 laddoos in 12 kg.
Number of laddoos that can be packed in 1 box = 16
So, the number of boxes required to pack 336 laddoos = 336 ÷ 16
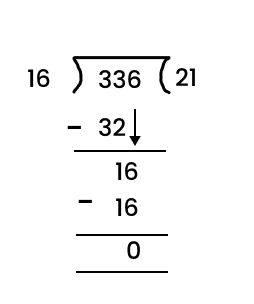
Therefore, the number of boxes needed to pack 336 laddoos is 21.
3. There are 26 rooms in a school. Each room has 4 plants. If each plant needs 2 cups of water, how much water do we need for all the plants?
Ans: Given that number of rooms in the school = 26
Number of plants in each room = 4
So, the total number of plants = 26 $\times$ 4= = 104
Number of cups needed for each plant = 2 cups
Then the total number of cups required for 104 plants = 104 $\times$ 2 = 208
Therefore, for all plants we need 208 cups of water.
Make the Best Story Problem
Each line gives a story. You have to choose the question which makes the best story problem. The first one is already marked.
1) A shopkeeper has 50 boxes. There are 48 fruits in one box
Tick the one question which matches with the given problem.
a) How muych will the shopkeeper pay in all? []
b) How many fruits are there in all?[✓]
c) How many more boxes will he need?[]
Explain why (a) and (c) are not good choices.
Ans: Option (a) is not a good choice because the price is not given in the story.
Option (c) is not a good choice because the total number of boxes is given in the story
2) 352 children from a school went on a camping trip. Each tent had a group of 4 children.
a) How many children did each tent have?
b) How many tents do they need?
c) How many children in all are in the school?
Ans: b) How many tents do they need?
3) A shopkeeper has 204 eggs. He puts them in egg trays. Each tray has 12 eggs.
a) How many more eggs will he need?
b) How many fresh eggs does he sell?
c) How many egg trays does he need?
Ans: c) How many egg trays does he need?
4) The cost of one book is Rs 47. Sonu buys 23 books
a) How much money does she have?
b) How much money does she pay for the books?
c) What is the cost of 47 books?
Ans: b) How much money does she pay for the books?
Practice Time
1) Do these divisions. Check your results by multiplication.
a) 438 ÷ 9 d) 900 ÷ 10
b) 3480 ÷ 12 e) 678 ÷ 6
c) 450 ÷ 7 f ) 2475 ÷ 11
Ans:
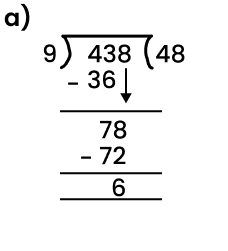
Here,
Quotient = 48, Remainder = 6 and Divisor = 9.
Checking:
We know that,
Divisor $\times$ Quotient + Remainder = 9 $\times$ 48 + 6 = 438 Dividend
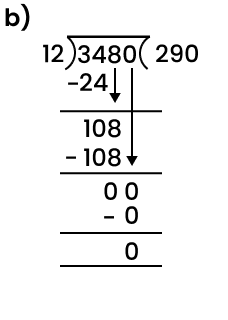
Here,
Quotient = 290, Remainder = 0 and Divisor = 12.
Checking:
We know that,
Divisor $\times$ Quotient + Remainder = 12 $\times$ 290 + 0 =3840 = Dividend
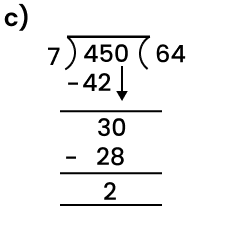
Quotient = 64, Remainder = 2 and Divisor = 7.
Checking:
We know that,
Divisor $\times$ Quotient + Remainder = 7 $\times$ 64 + 2 = 450 = Dividend
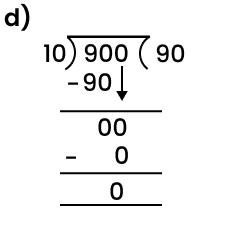
Quotient = 90, Remainder = 0 and Divisor = 10.
Checking:
We know that,
Divisor $\times$ Quotient + Remainder = 10 $\times$ 90 + 0 = 900 = Dividend

Quotient = 113, Remainder = 0 and Divisor = 6.
Checking:
We know that,
Divisor $\times$ Quotient + Remainder = 6 $\times$ 113 + 0 = 678 = Dividend
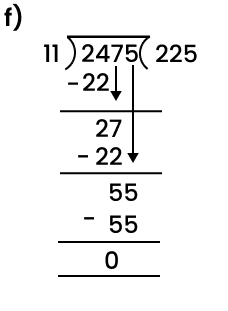
Quotient = 225, Remainder = 0 and Divisor = 11.
Checking:
We know that,
Divisor $\times$ Quotient + Remainder = 11 $\times$ 225 + 0 = 2475 = Dividend
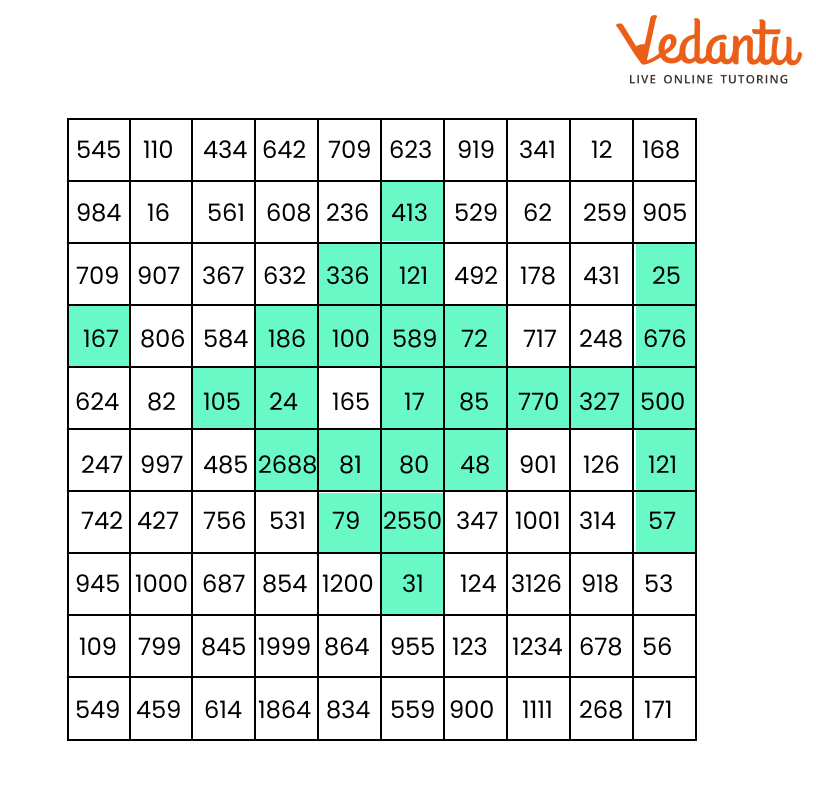
2) Solve the given sums and colour the answers in the grid given below. See what you find.
21 × 16
15 × 7
93 × 2
17 × 5
10 × 10
26 × 26
77 × 10
50 × 10
11 × 11
59 × 7
31 × 19
85 × 30
64 × 42
3200 ÷ 40
19 × 3
248 ÷ 8
432 ÷ 18
729 ÷ 9
825 ÷ 5
221 ÷ 13
576 ÷ 12
288 ÷ 4
869 ÷ 11
847 ÷ 7
981 ÷ 3
475 ÷ 19
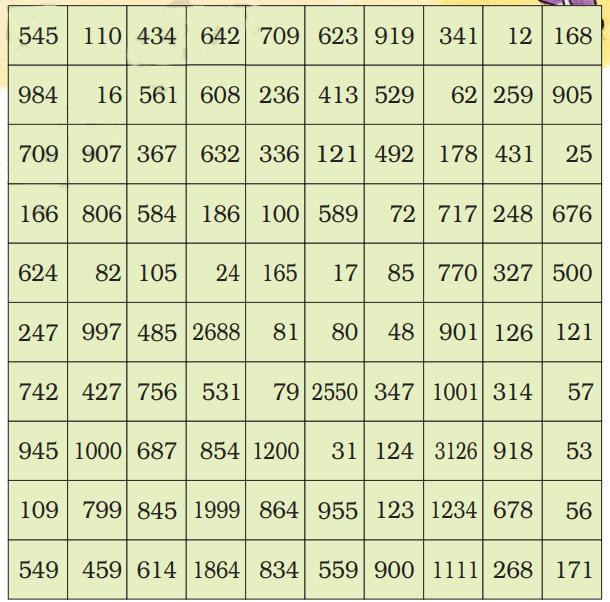
Ans:

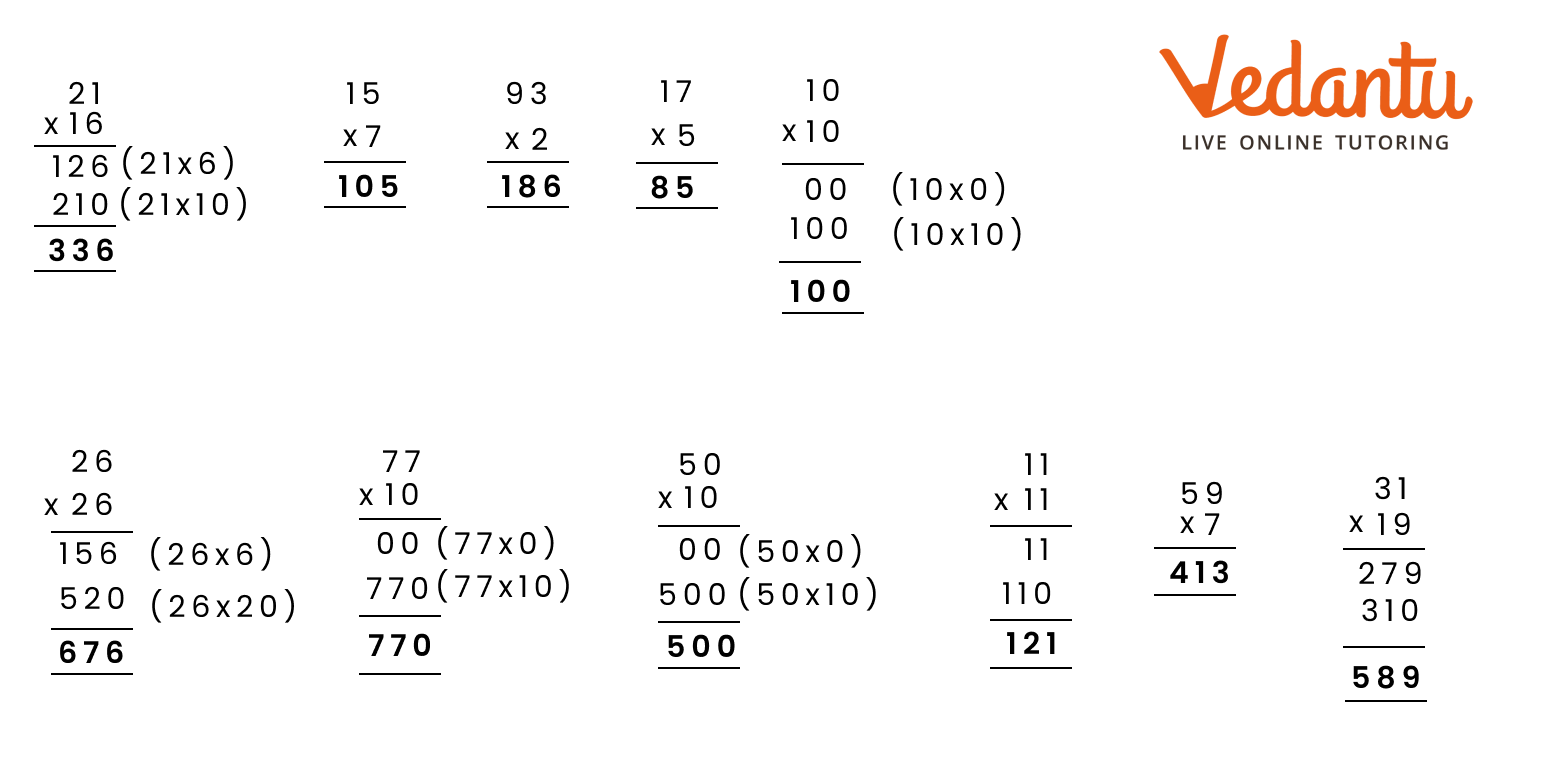
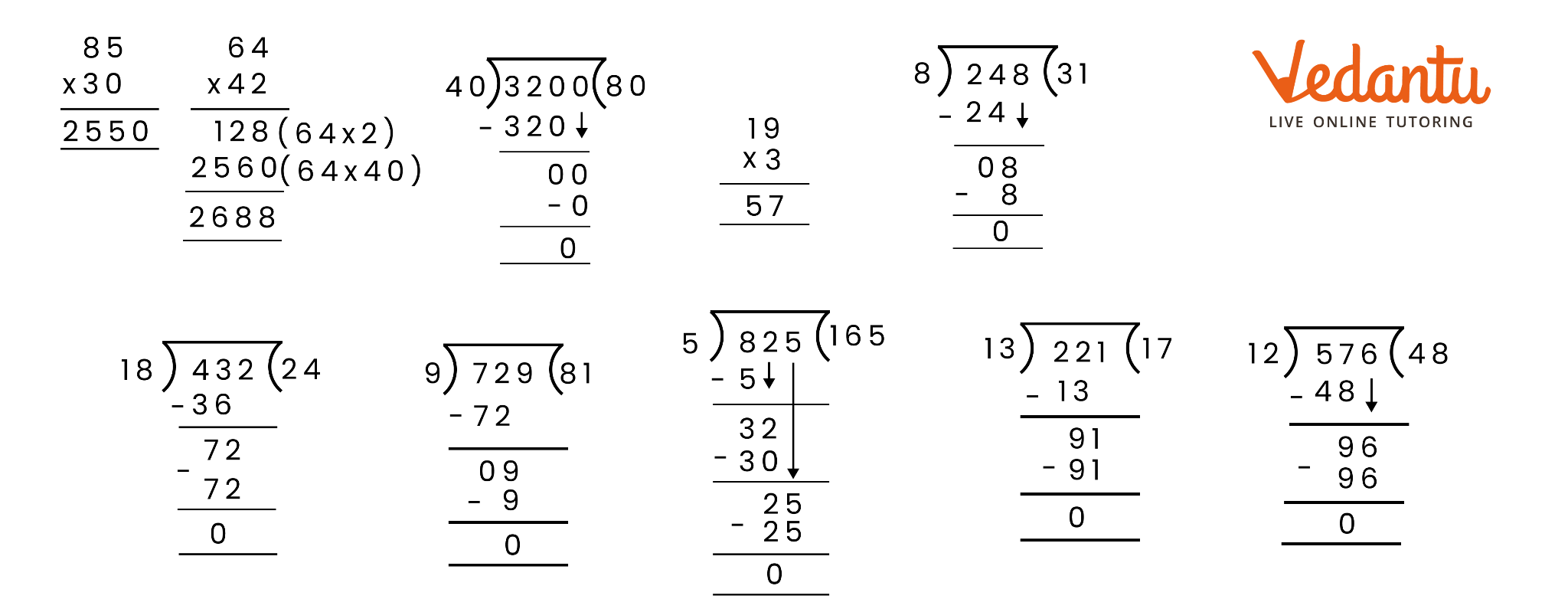
Tips and Tricks for Multiplication:
Use the Times Tables:
Encourage memorization of times tables up to 12.
Use flashcards and timed quizzes to make practice sessions engaging.
For larger numbers, break them down into smaller, more manageable parts.
Example: To multiply 23 by 7, think of it as (20 * 7) + (3 * 7).
Use skip counting for smaller numbers.
Example: To multiply by 4, skip count by fours (4, 8, 12, 16, etc.).
Tips and Tricks for Division:
Explain division as repeated subtracting the divisor from the dividend.
Example: 15 ÷ 3 can be seen as subtracting 3 from 15 until you reach 0.
Practice with simple examples and gradually move to more complex numbers.
Understand the relationship between multiplication and division.
Example: If they know that 4 × 5 = 20, they can figure out that 20 ÷ 4 = 5.
Use the trick of moving the decimal point to the left when dividing by 10 or 100.
Example: 250 ÷ 10 = 25 and 250 ÷ 100 = 2.5.
Estimate the quotient before dividing to make the process easier.
Example: For 47 ÷ 6, they might estimate that 6 × 7 = 42, so the answer is close to 7.
Conclusion
NCERT Solutions for Class 5 Math Chapter 13 "Ways to Multiply and Divide" is fundamental for building a strong mathematical foundation. This chapter introduces various methods for performing multiplication and division, such as the traditional algorithm, lattice multiplication, long division, and short division. What’s important to focus on is understanding each method thoroughly and practising different types of problems. In previous year question papers, around 4–5 questions from this chapter were typically asked, emphasizing its importance in the curriculum. By studying Vedantu’s detailed solutions and practising regularly, you can master these essential arithmetic operations.
Other Study Material for CBSE Class 5 Maths Chapter 13
S. No | Important Links for Chapter 13 Ways to Divide and Multiply |
1. | |
2. | Class 5 Ways to Divide and Multiply Important Questions |
Chapter-Specific NCERT Solutions for Class 5 Maths
Given below are the chapter-wise NCERT Solutions for Class 5 Maths. Go through these chapter-wise solutions to be thoroughly familiar with the concepts.
S. No | NCERT Solutions Class 5 Maths Chapter-wise List |
1 | |
2 | |
3 | |
4 | |
5 | |
6 | |
7 | |
8 | |
9 | |
10 | |
11 | |
12 | |
13 |
Important Related Links for CBSE Class 5 Maths
S. No | Other Study Materials for Class 5 Maths |
1 | |
2 | |
3 | |
4 | Important Questions for CBSE Class 5 Maths |
5 |
























