




Introduction to Gravity
We all have heard of the term gravity. It is what keeps us glued to the surface of the earth, and makes objects in flight fall towards the surface of the earth. It can be said that gravity is a force of attraction between the earth and any object on the surface of the earth. This is obviously a very crude definition of gravity, but it gives an overview of the topic. In physics, gravity is a fundamental interaction that causes all objects with mass or energy to be attracted to one another. Of the four fundamental interactions, gravity is by far the weakest force. Due to the low mass of particles, this force has almost no influence at the microscopic level. However, at the macroscopic level, gravity determines the motion of planets, stars and even light in the universe.
Newton’s Law of Gravitation
Sir Isaac Newton was the first person to lay down a law which would help calculate the force of gravity between any two bodies. Newton discovered the law of universal gravitation in 1687. It states that every particle in the cosmos is attracted to every other particle with a force that is directly proportional to the product of their masses and inversely proportional to the square of the distance from one another. Mathematically, this law can be given as:
$\begin{align} &F \propto \dfrac{M_{1} M_{2}}{R^{2}} \\ \\ &F=G \dfrac{M_{1} M_{2}}{R^{2}} \end{align}$
Here, M1 and M2 are the masses of two objects and R is the distance between those objects. G is the universal gravitational constant and its value is 6.674 $\times$ 10-11 Nm2kg-2.
This law is valid for all the bodies in the universe and is one of the most fundamental laws in physics. This law can explain everything from the motion of planets around the sun, the tides, the motion of satellites to why objects fall to the surface of the earth.
Gravitational Field and Potential
Gravitational Field
A gravitational field in layman's terms can be defined as the region around any object in which when another body is placed, the gravitational force is exerted on that object. More precisely, we can say that a massive body's effects on the surrounding area, which result in a force on another massive body, are described by a gravitational field. It is just a physical model similar to the electric field around charges. The gravitational field is defined as:
$E=\dfrac{F}{m}$
Here, E is the gravitational field, F is the gravitational force and m is the mass of the body on which the force is acting.
Suppose the body which has this field has mass M, then the force it exerts on the body of mass m will be:
$F=\dfrac{G M m}{R^{2}}$
R is the distance between their center of masses.
The expression for the gravitational field will then be:
$\begin{align} &E=\dfrac{\left(\dfrac{G M m}{R^{2}}\right)}{m} \\ \\ &E=\dfrac{G M}{R^{2}} \end{align}$
The SI unit of the gravitational field is Nkg-1.
The gravitational field is a vector quantity since force is a vector quantity.
Potential
Suppose we have a particle of mass m which is taken from a point X to Y, and all the other masses are kept fixed. If UX and UY are the potential energies at X and Y respectively, then the change in potential between two points will be:
$V_{Y}-V_{X}=\dfrac{U_{Y}-U_{X}}{m}$
If we take X to be the reference point such that the potential at X is 0, then we have:
$V_{Y}=\dfrac{U_{Y}-U_{X}}{m}$
Using the above equation, we can define gravitational potential at a point to be equal to the change in potential energy per unit mass, when the mass is brought from the reference point to the given point. The reference point is taken as infinity.
Relationship between Gravitational Field and Gravitational Potential
Using the expression of gravitational field we can write:
F=mE
Suppose, now we move the particle from point r to r+dr, then the work done will be equal to:
$\begin{align} &d W=F \cdot d r \\ \\ &d W=m E \cdot d r \end{align}$
We also know that for a conservative force:
dU=-dW
We know that gravity is a conservative force because the work done by gravity to go from point A and then back to point A is zero. So we can write:
$dU=-mE\cdot dr$
We also know that:
$d V=\dfrac{d U}{m}$
So we get:
$\begin{align} &\dfrac{d U}{m}=-E \cdot d r \\ \\ &d V=-E \cdot d r \end{align}$
Integrating between point x and y we get:
$V(y)-V(x)=-\int_{x}^{y} E \mathrm{~d} r$
If we take the potential at point x to be 0 then we get:
$V(y)=-\int_{x}^{y} E \mathrm{~d} r$
This is the relationship between the gravitational field and gravitational potential.
Gravitational Field and Potential due to Spherical Bodies
We will now calculate the gravitational field and potential due to a solid sphere.
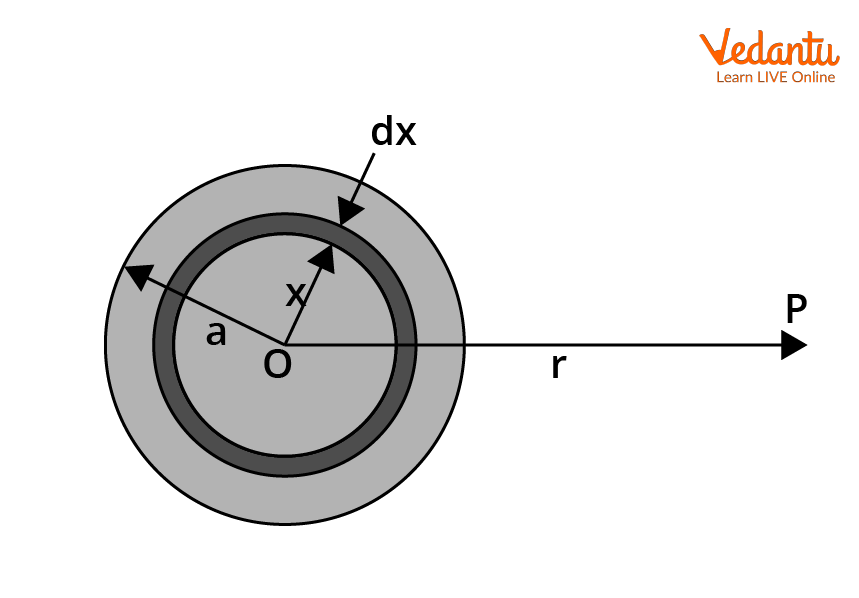
A solid sphere
Suppose we have a solid sphere as shown above, and we have to find the field at a point P outside the sphere. We will divide the sphere into thin concentric shells and let their masses be dm. The field due to this thin shell will be given as:
$\begin{align} &d E=\dfrac{G d m}{r^{2}} \\ \\ &\int d E=\dfrac{G}{r^{2}} \int d m \\ \\ &E=\dfrac{G M}{r^{2}} \end{align}$
Similarly, the expression of gravitational field at the surface of sphere is,
$E=-\dfrac{GM}{a^2}$
Suppose we now want to find the field at a point r inside the sphere. We know that since the sphere we have is uniform, its mass density is constant. We can again write the field as the summation of the fields of thin concentric shells of mass dm, but this time the integral will be till point r. Thus, only the masses of the shells with radii less than r should be added in order to get the integral of dm. We need to find the mass of the sphere of radius r. The radius of the whole sphere is a. We can thus find the mass using the fact that the mass density will be constant.
$\dfrac{M}{\left(\dfrac{4}{3} \pi a^{3}\right)}\left(\dfrac{4}{3} \pi r^{3}\right)=\dfrac{M r^{3}}{a^{3}}$
So for our case we have:
$\int d m=\dfrac{M r^{3}}{a^{3}}$
So the field will be:
$\begin{align} &E=\dfrac{G M r^{3}}{a^{3} r^{2}} \\ \\ &E=\dfrac{G M r}{a^{3}} \end{align}$
We haven’t considered the direction of the fields. The gravitational field always points towards the mass which is creating the field, so if we take a vector away from the mass, then the field will be negative as it points towards the mass. The direction is just a matter of convention. For calculating potential we will consider the direction, and so we’ll take both fields to be negative as to consider the potential we have taken the vector dr from r to infinity which is opposite to the gravitational field.
The potential can be found by integrating the fields. For a point outside the sphere, we can find the potential as:
$\begin{align} &V=-\int_{\infty}^{r}-\dfrac{G M}{r^{2}} \mathrm{~d} r \\ \\ &V=G M\left[-\dfrac{1}{r}\right]_{\infty}^{r} \\ \\ &V=\dfrac{G M}{r} \end{align}$
For a point on the surface of the sphere, the expression of gravitational potential is calculated as follows,
The expression of gravitational field at the surface of sphere is,
$E=-\dfrac{GM}{a^2}$
Hence potential, $V= -\dfrac{GM}{a}$
Similarly, for the point inside the sphere, we can write:
$\begin{align} &V=-\int_{\infty}^{r}-E \mathrm{~d} r \\ \\ &V=-\int_{\infty}^{a}-E \mathrm{~d} r+-\int_{a}^{r}-E \mathrm{~d} r \end{align}$
We have broken up the integral because there is a discontinuity at point a, that is the surface of the sphere.
$\begin{align} &V=G M \int_{\infty}^{a} \dfrac{1}{r^{2}} \mathrm{~d} r+\dfrac{G M}{a^{3}} \int_{a}^{r} r \mathrm{~d} r \\ \\ &V=G M\left[-\dfrac{1}{r}\right]_{\infty}^{a}+\dfrac{G M}{2 a^{3}}\left[r^{2}\right]_{a}^{r} \\ \\ &V=-\dfrac{G M}{a}+\dfrac{G M}{2 a^{3}}\left[r^{2}-a^{2}\right] \end{align}$
Upon further simplification, we get;
$V=-\dfrac{GM}{2a^3}(3a^2-r^3)$
This is the expression of gravitational potential for a point inside the sphere.
Conclusion
Gravity is an attractive force between objects, and it is given by the law of universal gravitation, which states that every particle in the cosmos is attracted to every other particle with a force that is directly proportional to the product of their masses and inversely proportional to their distance from one another. The gravitational field is a model that is used to explain how the gravitational field acts on another body that is at a distance from it.
Any object with a mass has a gravitational field in the region around it and any object that is in this field is acted upon by a force of gravity. The change in potential energy per unit mass, when a mass is transported from a reference point to a specific point, determines the gravitational potential at that location. The relationship between the potential V and the field E is given as:
$V(y)=-\int_{x}^{y} E \mathrm{~d} r$
FAQs on Relationship between Gravitational Field and Potential | JEE
1. Since gravity is unlimited, can we use it as an infinite energy source?
No, gravity can not be used as an infinite energy source. In fact, strictly speaking, gravity itself can not be used as an energy source at all. You are confusing forces with energy, which are very different things. Energy is a property of objects, such as balls, atoms, light beams or batteries. In contrast, forces describe the interaction between objects. Forces are the way that energy is transferred from one object to another when they interact, but forces are not the energy itself.
2. Why is gravity important?
The force that pulls items toward the center of a planet or other entity is called gravity. All the planets are kept in orbit around the sun by the force of gravity. Ocean tides are a result of the moon's gravitational effect on the oceans. The stuff that stars and planets are composed of is pulled together by gravity to form them. Light is also pulled towards matter by gravity. This idea was developed by Albert Einstein.
































