




An Introduction to Apparent Forces
You might be wondering why we started with apparent forces. So, the answer lies in the fact that we calculate effective gravity using the concept of apparent forces. It is better to lay the groundwork for this concept, and then move on to deriving effective gravity and understanding it. An apparent force is a force that appears to act on a mass whose motion is described using a non-inertial frame of reference, such as an accelerating or rotating reference frame. It is also known as a pseudo force, d'Alembert force, or inertial force.
For example, whenever a passenger car accelerates in the forward direction, the passengers feel as though a force is acting on them from the back, forcing them back into their seats. Take another example, when an elevator moves down, it feels like a force is acting on us in the upwards direction. The force that seems to drive things outward and towards the rim of a centrifuge is an illustration of this in a spinning reference frame. These are just a few examples of apparent forces.
Centrifugal Force
The centrifugal force is a type of fictitious force that acts on a body in any rotating frame of reference. It is always directed away from the axis of rotation. We can define centrifugal force as a fictitious force in a circular motion that is directed away from the centre of the circle. When measurements are taken in an inertial frame of reference, the force does not exist. It only becomes relevant when we switch from a ground/inertial reference frame to a rotating reference frame.
Consider an object tied at the end of a string whirled around in a circle. If we consider an inertial frame of reference, then we know that the object is being accelerated towards the centre of the circle, which allows it to continue moving in a circular path. It is this acceleration that changes the direction of the velocity of the object. This acceleration, however, does not change the magnitude of the velocity. This acceleration is known as the centripetal acceleration, and in our case, the force that causes this acceleration is called the tension in the string.
All this is valid from a stationary frame of reference. Consider another frame of reference. Assume that the frame of reference is on the object itself. Then the object will appear stationary to any observer who is observing from the object’s frame of reference. This frame of reference is non-inertial as it is accelerating. So an additional apparent force must be included in the equations to balance the tension due to the string. This apparent force is known as centrifugal force.
The centripetal force formula, which represents a true event, is essentially used to describe the fake centrifugal force by reversing the direction of the force.
The centrifugal force is given as
$F=-\dfrac{mv^2}{R}$
Since,
$v=R\omega$
$F=-m\omega^2R$
Here R is the radius of the circle that the object is moving in, m is the mass of the object, and $\omega$ is the angular velocity. The term $\omega^2R$ is centripetal acceleration.
Effective Gravity
We have discussed that the centrifugal force acts on objects that are in a rotating frame of reference. The earth also rotates around its axis, and it is a rotating frame of reference, so when we are computing gravity, which acts towards the centre of the earth. We also have to include a centrifugal force due to the rotation of the earth, which acts in the direction opposite to that of the gravitational force. This centrifugal force affects the value of the gravitational force due to the earth.
In more precise terms, we can say that all mass is drawn towards the earth's centre of mass by the true gravitational acceleration. All objects are, nevertheless, drawn away from the axis of planetary rotation by centrifugal force. The vector sum of these two forces is the effective gravity. It does not point precisely towards the earth's centre.
Let us pretend that the earth is a sphere with a mass "M" and a radius "R." When the earth is not rotating, the weight of an object of mass "m" at point P at latitude "$\phi$" is mg, as we are all aware. However, the earth rotates at an angular velocity, and so if a frame of reference on the earth is to be considered, there will be a centrifugal force acting on the object.
The following figure depicts the object travelling in a circular path with a radius of r and the centrifugal force acting on it.
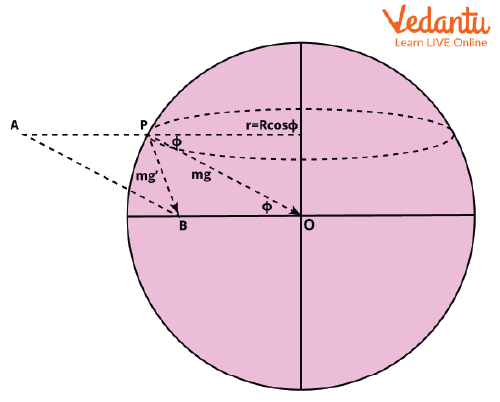
Effective Gravity
From the figure, the angle between PO and PA is 180 - $\phi$. Now using the parallelogram law of vector addition we can find PB as
$P B^{2}=P O^{2}+P A^{2}+2 \times P O \times P A \times \cos (180-\phi)$
PO is the gravitational force, PA is the centrifugal force and PB is the effective gravity that we are trying to calculate. We know the values of PO and PA, so substituting them gives,
$\begin{align} &\left(m g^{\prime}\right)^{2}=(m g)^{2}+\left(m \omega^{2} R \cos \phi\right)^{2}+2(m g)\left(m \omega^{2} R\right)(-\cos \phi) \\ \\ &m^{2} g^{\prime 2}=m^{2} g^{2}+m^{2} \omega^{4} R^{2} \cos ^{2} \phi-2 m^{2} g \omega^{2} R \cos \phi \\ \\ &g^{\prime 2}=g^{2}\left(1+\frac{m^{2} \omega^{4} R^{2} \cos ^{2} \phi}{g^{2}}-\frac{2 m^{2} g \omega^{2} R \cos \phi}{g}\right) \end{align}$
Since the angular acceleration of the Earth is of the order of $\dfrac{10^{–4}}{s}$, we can neglect $\omega^4$ in the above equation.
We then get
$g^{\prime}=g\left(1-\dfrac{2 \alpha^{2} R \cos ^{2} \phi}{g}\right)^{\dfrac{1}{2}}$
Applying binomial expansion and then neglecting higher powers we can write,
$\begin{align} &g^{\prime}=g\left(1-\dfrac{\omega^{2} R \cos ^{2} \phi}{g}\right) \\ \\ &g^{\prime}=g-\omega^{2} R \cos ^{2} \phi \end{align}$
This is the expression for effective gravitational acceleration. If we multiply the mass of the object on both sides, then we get the effective gravity. The earth's gravity is affected by rotation. The term g is the earth’s gravitational acceleration. The gravity at the centre of the earth will be zero.
Conclusion
A force that seems to act on a mass whose motion is defined by a non-inertial frame of reference, such as an accelerating or rotating reference frame, is referred to as an apparent force. Centrifugal force is a type of apparent or fictitious force that acts on a body in a rotating frame of reference. Due to the rotation of the earth around its axis, a centrifugal force acts on the bodies on the surface of the earth and this affects the gravity. The actual gravitational acceleration pulls all mass toward the centre of mass of the earth. Centrifugal force, however, pulls all things away from the axis of planetary rotation. Effective gravity is the vector sum of these two forces.
FAQs on Effective Gravity - JEE Important Topic
1. At what height is gravity zero?
Except perhaps at infinity, gravity can never be zero. Although the gravitational force weakens as we get further from the earth's surface, it will never be zero. The relationship between r2 and the gravitational force is inverse. The distance r between this location and the earth's surface. Since gravity is a weak force, as the distance from the earth increases, the influence of gravity begins to fall, but it does not go to zero.
2. Why is the acceleration due to gravity zero at the centre of the earth?
At the centre of the earth, where the mass of the planet is equally distributed in all directions, there is no acceleration caused by gravity because there is a net-zero pull in all directions. It can also be said that since all the forces at the earth's core negate one another, there is no gravitation. The earth's top and bottom halves each provide a force, but these forces cancel one another out.




















