




Wave in a String - An Introduction
A simple wave is produced in a string when it is stretched between two horizontal poles and pulled. That section of the string will pick up kinetic and elastic energies as the wave passes across it. In general, a wave in a string is the disturbance that moves across the string while carrying energy and velocity. Transverse waves make up the waves in a string.
In this article, we will discuss the velocity of transverse waves in string v along with the properties of the waves produced, mathematical description of the wave velocity and tension, etc.
Properties of Waves
The following are the properties of waves:
Peaks and troughs are features of transverse waves. The top point of the wave is called the crest, while its bottom point is called the trough.
The biggest deviation a particle makes from its equilibrium position is its amplitude.
The distance between one peak and the next peak, or one trough and the next trough is known as the wavelength and is typically symbolised by the Greek letter lambda \[\left(\lambda\right)\], which is measured in metres.
The time it takes for two successive peaks, or one wavelength to pass through a fixed point is known as the period, commonly indicated as T and measured in seconds.
While fixed at the end point or while free at the end point, transverse waves might form.
Wave Speed on a Stretched String
The qualities of the medium affect a wave's speed. For instance, the sound of a guitar is created by the vibration of the strings. The wavelength and wave speed on the strings influence the frequency of the sound that is produced. A guitar's strings can be composed of materials that are similar in composition but varied in thickness. Since the linear density is determined by the mass per length, they each have distinct linear densities.
\[\mu=\dfrac {m}{l}\]
Where ‘m’ and ‘l’ are mass and length of string, respectively.
The properties of the medium affect the wave's velocity in a string. For instance, if a thin guitar string is vibrated while a thick rope is not, the guitar string's waves will move more quickly. As a result, the linear densities of the two threads affect the string's velocity. Linear density is defined as the mass per unit length.
Wave Speed on a String under Tension
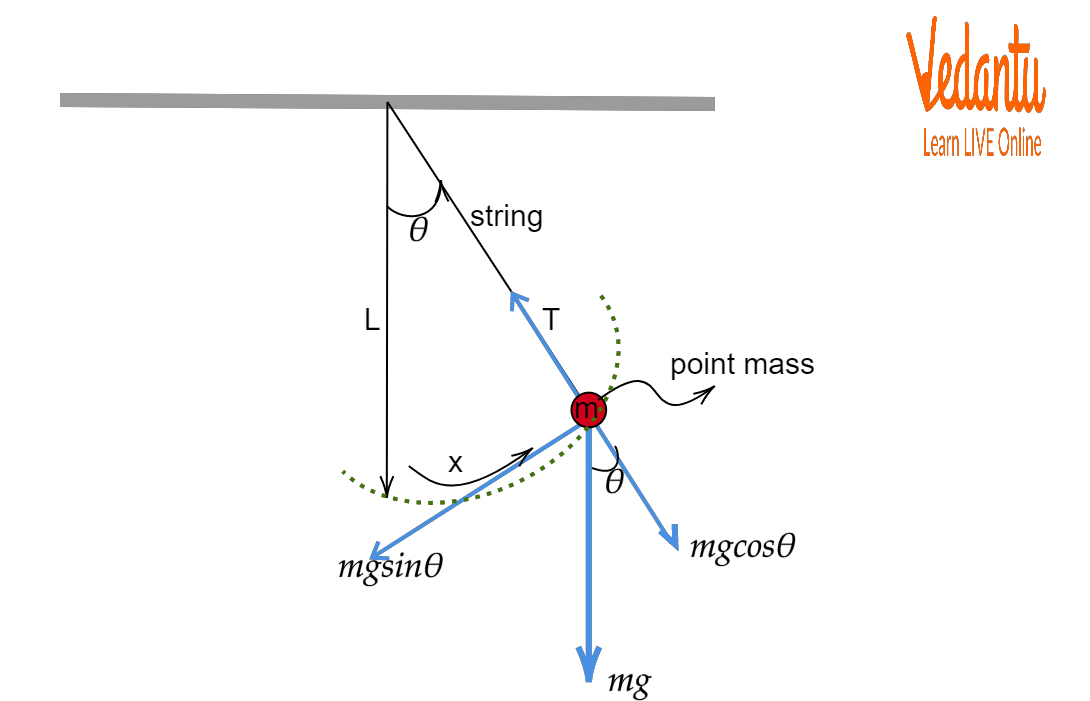
The tension of the strings affects the string's wave velocity as well. Consider a pulse delivered down a taut string to show how the tension and linear density affect the wave's speed. The tension in the string FT is constant while the taut string is at rest in the equilibrium position. Take into account a small string component with mass \[\Delta {m}=\mu ~\delta {x}\]. The tension on either side of the mass element is equal and opposing, and the mass element is at rest and in equilibrium.

Tension on Mass Element of String
A transverse wave moves in the positive x-direction when you pull a string while it's under tension. Although the mass element is tiny, it is expanded to make it more obvious. The string's restoring force causes the small mass element to oscillate perpendicular to the wave motion rather than moving in the x-direction. Approximately constant, regardless of position and time, the tension $F_{T}$ in the string acts in both the positive and negative x-directions.
Assume that the displaced string has a slight inclination with respect to the horizontal axis. The tension in the string and the restoring force are added to provide the net force acting parallel to the string on the string element. The net force is equal to the total of the y-components of the force since the x-components of the force of tension cancel. The magnitude of the x-component of the force is equal to the horizontal force of tension of the string ${{F}_{T}}$. To obtain the y-components of the force, note that \[\tan {{\theta }_{1}}=\frac{-{{F}_{1}}}{{{F}_{T}}}\] and \[\tan {{\theta }_{2}}=\frac{{{F}_{2}}}{{{F}_{T}}}\] . The $\tan \theta$ is equal to the slope of a function at a point, which is equal to the partial derivative of y with respect to x at that point.
Wave Speed on a String
Therefore, \[\frac{{{F}_{1}}}{{{F}_{T}}}\] is equal to the negative slope of the string at \[{{x}_{1}}\] and \[\frac{{{F}_{2}}}{{{F}_{T}}}\] is equal to the slope of the string at x2:
\[\frac{{{F}_{1}}}{{{F}_{T}}}=-{{(\frac{\partial y}{\partial x})}_{{{x}_{1}}}}\] and \[\frac{{{F}_{2}}}{{{F}_{T}}}=-{{(\frac{\partial y}{\partial x})}_{{{x}_{2}}}}\]
The equation for the net force acting on the element with a tiny mass is
\[{{F}_{net}}={{F}_{1}}+{{F}_{2}}={{F}_{T}}[{{(\frac{\partial y}{\partial x})}_{{{x}_{2}}}}-(\frac{\partial y}{\partial x})]\]
The net force is equal to the mass times the acceleration, as stated by Newton's second law. The linear density of the string $\mu$ is the mass per length of the string, and the mass of the portion of the string is $\mu \Delta x$ ,
\[{{F}_{T}}[{{(\frac{\partial y}{\partial x})}_{{{x}_{2}}}}-{{(\frac{\partial y}{\partial x})}_{{{x}_{1}}}}]=\Delta ma=\mu \Delta x\frac{{{\partial }^{2}}y}{\partial {{t}^{2}}}\]
Dividing by ${{F}_{T}}\Delta x$ and taking the limit as $\Delta x$ approaches zero,
\[\Rightarrow \frac{{{\partial }^{2}}y}{\partial {{x}^{2}}}=\frac{\mu }{{{F}_{T}}}\frac{{{\partial }^{2}}y}{\partial {{t}^{2}}}\]
Recall that the linear wave equation is
\[\frac{{{\partial }^{2}}y(x,t)}{\partial {{x}^{2}}}=\frac{1}{{{v}^{2}}}\frac{{{\partial }^{2}}y(x,t)}{\partial {{t}^{2}}}\]
Therefore,
\[\frac{1}{{{v}^{2}}}=\frac{\mu }{{{F}_{T}}}\]
When we solve for v, we can see that the linear density and tension have an impact on the wave's speed. With the use of the following equation, one may determine the speed of a pulse or wave on a string under tension. Transverse waves on a string have wave speed
|v|= \[ \sqrt{\frac{{{F}_{T}}}{\mu }}\]
where ${{F}_{T}}$ is the tension in the string and $\mu$ is the mass per length of the string.
Conclusion
In conclusion, we have learnt that a wave on a string can be visualised as a disturbance starting at one end and travelling to the other end. A wave carries velocity and energy across the ends. A wave is called a transverse wave when the direction of propagation of the wave is perpendicular to the direction of vibrating particles.
A wave is longitudinal when the direction of propagation is the same as the vibrating particles. A wave in physics is depicted by a crest and a trough. The distance between the point from where a crest starts to a point where the trough ends is the wavelength. The wavelength and wave speed on the strings influence the frequency of the sound that is produced. Medium properties affect wave’s properties like frequency. A string under tension affects the wave properties.
FAQs on Wave Velocity on a String - JEE Important Topic
1. Describe the dependence of waves on the medium.
Most waves move at varying speeds depending on the medium. In general, waves move through solids and gases at different speeds. This is due to the fact that particles are more closely spaced in solids than they are in gases. The energy distribution takes longer to move from one particle to another across the medium when the particles are more apart.
2. What effect does string length have on wave speed? Mention some other factors affecting it if any.
The length of the string determines the fundamental wavelength of the wave. So when a string is stretched and the tension increases, the wave speed also increases which in turn increases the frequency.
The linear density of the string apart from the tension is also a factor that affects speed of a wave. The mass per unit length is an elastic property of the string material. The wave speed decreases when the value of the linear density increases.










