




Stokes’ Law: A Brief Introduction
We all know that according to Newton’s law of gravitation, the earth exerts a downward force on all objects on our planet. But have you ever wondered how come we don’t see any clouds falling? The answer for this amazing question is that Stokes theorem concepts are the reason behind this.
A British scientist named Sir George G. Stokes developed the formula of Stokes’ Law in 1851. He also considered the forces operating on a specific particle that sinks through a liquid column under gravity’s influence. Let us read the complete article to obtain a better understanding of Stokes’ Law and terminal velocity concept.
Stokes’ Law Definition
A mathematical equation that expresses the settling velocities of the small spherical particles in a fluid medium is known as Stokes’ Law. This law is derived by considering the forces acting on a particular particle as it sinks through the liquid column under the influence of gravity. The force that retards a sphere moving through a viscous fluid is directly proportional to the velocity and the radius of the sphere, and the fluid’s viscosity.
Stokes Theorem Formula
By Stoke’s Law equation, we can define the drag force acting on the fluids and it is given by:
F = 6πηrv
Here,
F is the drag force or frictional force at the interface
$\eta $ is the viscosity of a liquid
r is the radius of the spherical body
v is the velocity of flow
As a result of drag force acting on the fluid at the same time, gravitational force will also be acting on the fluid and is given by:
${F_g}=\dfrac {4}{3}~ \pi ~{r^3}~\left(\rho-\sigma\right)~g$
Where,
$\rho$ - Density of the liquid
$\sigma$ - Density of the spherical body
Understanding Stokes’ Law
To understand exactly what Stokes’ Law is, we have to dive into the explanation of it. Stokes’ Law describes the viscous forces acting on a body as it is dropped into a fluid. Due to the viscous forces, a body falling through a liquid falls slowly or, in simple words, its velocity does not achieve a higher value. As a spherical body falls under the effect of its weight, the body de-accelerates; the velocity of the body increases but it cannot increase beyond a maximum value.
The law states that when a body falls through a fluid, it drags the layer of the fluid in contact with it. A relative motion is developed between the different layers of the fluid, and as a result of it, the body experiences a retarding force. This retarding force acting on a body as it passes through a viscous fluid is directly proportional to the sphere’s velocity, radius, and fluid viscosity.
Stokes Theorem Examples
A few commonplace examples of such a motion include:
A person falling with a parachute.
Pouring raindrops.
Swinging a pendulum bob.
It has been observed that the viscous force is proportional to the velocity of the object and is opposite to the direction of motion.
Stokes’ Law Derivation
The statement of Stokes’ Law can be presented as: the drag forces which involve the viscous and frictional forces, acting on a spherical particle moving through a fluid is given by the equation:
$F=6\pi \eta rv$
Where
F is the drag force acting on the particle
$\pi $ is a mathematical constant
$\eta $ is the viscosity of the fluid
r is the radius of the particle
v is the velocity of the particle when entering the fluid
When a sphere or a body moves through a fluid, a friction force must be overcome. The Stokes’ Law, named after the scientist George Gabriel Stokes, quantitatively gives the relationship between the frictional force that acts between a liquid and a spherical body that falls through it based on quantities, such as its radius and velocity.
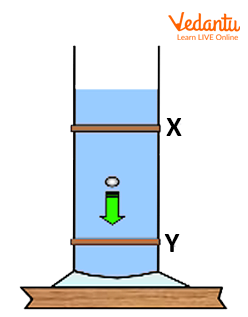
A Ball Moving in Fluid
Mathematically, this can be written as
\[F\propto {{\eta }^{a}}{{r}^{b}}{{v}^{c}}\]
Here, to calculate the values of a, b and c, substitute the proportionality sign with an equality sign as:
\[F=k{{\eta }^{a}}{{r}^{b}}{{v}^{c}}---->(1)\]
Here, K represents the constant of proportionality.
Now, let write the dimensions of the quantity on both the sides of equation (1), then we get:
$[{{M}^{-1}}{{L}^{-1}}{{T}^{-2}}]=k{{[{{M}^{-1}}{{L}^{-1}}{{T}^{-1}}]}^{a}}\text{ }{{[{{M}^{0}}{{L}^{-1}}{{T}^{0}}]}^{b}}\text{ }{{[{{M}^{0}}{{L}^{1}}{{T}^{-1}}]}^{c}}$
Now on simplifying the equation we get:
\[[{{M}^{1}}{{L}^{1}}{{T}^{-2}}]=[{{M}^{a}}{{L}^{-a+b+c}}{{T}^{-a-c}}]----(2)\]
The independent entities of mass, length and time can be equated across the left and right hand sides of the equation as per classical mechanics. Thus, equating the powers of quantities, we get:
$\Rightarrow a=1-->(3)$
$ \Rightarrow -a+b+c=1--->(4) $
$Or\text{ }-a-c=-2 $
$\Rightarrow a+c=2--->(5)$
Now, substitute the value from equation (3) into equation (5), we get:
$1+c=2$
$ c=1$
Using the above obtained values in equation (4), we get:
$ -1+b+1=1$
$b=1$
Substituting these values in the equation (1), we get:
$F=k\eta rv$
Even though various experiments were conducted, the value of k was found to be equal to $6\pi $. Thus, the drag force acting on a spherical body failing through a liquid becomes $F=6\pi \eta rv$. Thus, this is the equation of Stokes’ Law derivation.
What is Terminal Velocity ?
Terminal velocity is defined as the maximum speed that an object can obtain as it falls. The terminal velocity speed will be different for each object because the way that air resistance can act on different objects changes based on the shape of that object.
In fluid mechanics, for an object to attain its terminal velocity, it should have a constant speed against the force exerted by the fluid through which it is moving. This means that an object with a large projected area relative to its mass, such as a parachute, has a lower terminal velocity than one with a small projected area relative to its mass, such as a dart. In general, for the same shape and material, the terminal velocity of an object increases with size.
Terminal Velocity Formula
The formula of terminal velocity is:
\[ {{v}_{t}}=\sqrt{\frac{2mg}{\rho A{{C}_{A}}}} \]
\[ V=\sqrt{\frac{2mg}{\rho A{{C}_{d}}}} \]
Terminal Velocity Derivation
Let’s derive terminal velocity using mathematical terms according to the drag equation, which will be discussed below.
Gravitational force and drag force are the two external forces acting on an object that is
falling through the fluid. The gravitational force is exerted as the weight of the object. This force is equal to the product of the mass of the falling object and gravitational acceleration.
$W=mg$
Here, W is the weight of the object, m is the mass of the object and a is the acceleration.
Drag force is the force exerted by the fluid stream on the object which is falling through it. The drag equation is expressed with the help of the following expression:
\[ F_d=\frac{{{C}_{d}}\rho {{V}^{2}}A}{2} \]
Here, Fd is the drag force, Cd is the drag coefficient, ρ density of the fluid, V is the velocity and A is the area projected by the object.
The value of drag increases with the square of the velocity of the object. For the terminal velocity, the object reaches the maximum velocity and the acceleration becomes equal to zero. With zero vertical acceleration, the constant vertical velocity of the object is called the terminal velocity. For this case, the drag becomes equal to weight and there is no external force now acting on the object. At terminal velocity, the net force becomes equal to zero.
Therefore, this is the required terminal velocity:
$F_d=W$
\[ \frac{{{C}_{d}}\rho {{V}^{2}}A}{2}=mg \]
\[ {{V}^{2}}=\frac{2mg}{\rho A{{C}_{d}}} \]
\[ V=\sqrt{\frac{2mg}{\rho A{{C}_{d}}}} \]
Summary
Let's summarise the article with key points. A mathematical equation that expresses the settling velocities of the small spherical particles in a fluid medium is known as Stokes’ Law. This law is derived by considering the forces acting on a particular particle as it sinks through the liquid column under the influence of gravity. The maximum speed that an object can obtain as it falls is known as terminal velocity. When it comes to the application of terminal velocity, hang gliders and parachutes are examples of terminal velocity used in our daily lives. Here in this article, we have also discussed their formulae and derivations. We hope the article was helpful.
FAQs on Stokes’ Theorem and Terminal Velocity for JEE
1. Does terminal velocity exist in the vacuum?
There is no terminal velocity in the vacuum as terminal velocity is only attained when the gravity force is perfectly equal to the force of air resistance and friction. If the air resistance and friction force is eliminated from the atmosphere, then there is nothing left for opposing the fall of the object, resulting in the object falling faster. Thus, it is proved that the terminal velocity doesn’t exist in the vacuum and rather it is possible in the fluid like air.
2. When do we use the Stokes’ Law? Mention four conditions of Stokes’ Law.
Stokes’ Law is used to determine the terminal velocity, and the size and the density of sphere and liquid, respectively. We also used Stokes’ Law to calculate the viscosity of the fluid.
Conditions under which Stokes’ Law is valid are:
The fluid through which the body moves must have infinite extension.
The body is perfectly rigid and smooth.
There is no slip between the body and the fluid.
The motion of the body does not give rise to turbulent motion.










